(2x – y)3 – 3x(y – 2x)2 – 4x2 + 4xy – y2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
VN
2
9 tháng 8 2021
phân tích đa thức
(2x – y)^3 – 3x(y – 2x)2 – 4x2 + 4xy – y ^2
= -(y-2x)^2(y+x+1)
đây nha bạn
9 tháng 8 2021
\(\left(2x-y\right)^3-3x\left(y-2x\right)^2-4x^2+4xy-y^2\)
\(=\left(2x-y\right)^3-3x\left(2x-y\right)^2-\left(2x-y\right)^2\)
\(=\left(2x-y\right)^3-\left(2x-y\right)^2\left(3x+1\right)\)
\(=\left(2x-y\right)^2\left(2x-y-3x-1\right)\)
\(=\left(2x-y\right)^2\left(-x-y-1\right)\)
7 tháng 10 2021
\(\left(2x-y\right)\left(4x^2-4xy+y^2\right)-8x^2\left(x-y\right)\)
\(=8x^3-y^3-8x^3+8x^2y\)
\(=8x^2y-y^3\)
27 tháng 8 2023
a) \(\left(x+2y\right)^2-\left(x-y\right)^2=\left(x+2y+x-y\right)\left(x+2y-x+y\right)\)
\(=\left(2x+y\right).3y\)
b) \(\left(x+1\right)^3+\left(x-1\right)^3\)
\(=\left(x+1+x-1\right)\left[\left(x+1\right)^2-\left(x+1\right)\left(x-1\right)+\left(x-1\right)^2\right]\)
\(=2x\left[\left(x+1\right)^2-\left(x^2-1\right)+\left(x-1\right)^2\right]\)
c) \(9x^2-3x+2y-4y^2\)
\(=9x^2-4y^2-3x+2y\)
\(=\left(3x-2y\right)\left(3x+2y\right)-\left(3x-2y\right)\)
\(=\left(3x-2y\right)\left[3x+2y-1\right]\)
d) \(4x^2-4xy+2x-y+y^2\)
\(=4x^2-4xy+y^2+2x-y\)
\(=\left(2x-y\right)^2+2x-y\)
\(=\left(2x-y\right)\left(2x-y+1\right)\)
e) \(x^3+3x^2+3x+1-y^3\)
\(=\left(x+1\right)^3-y^3\)
\(=\left(x+1-y\right)\left[\left(x+1\right)^2+y\left(x+1\right)+y^2\right]\)
g) \(x^3-2x^2y+xy^2-4x\)
\(=x\left(x^2-2xy+y^2\right)-4x\)
\(=x\left(x-y\right)^2-4x\)
\(=x\left[\left(x-y\right)^2-4\right]\)
\(=x\left(x-y+2\right)\left(x-y-2\right)\)
KV
27 tháng 8 2023
a) (x + 2y)² - (x - y)²
= (x + 2y - x + y)(x + 2y + x - y)
= 3y(2x + y)
b) (x + 1)³ + (x - 1)³
= (x + 1 + x - 1)[(x + 1)² - (x + 1)(x - 1) + (x - 1)²]
= 2x(x² + 2x + 1 - x² + 1 + x² - 2x + 1)
= 2x(x² + 3)
c) 9x² - 3x + 2y - 4y²
= (9x² - 4y²) - (3x - 2y)
= (3x - 2y)(3x + 2y) - (3x - 2y)
= (3x - 2y)(3x + 2y - 1)
d) 4x² - 4xy + 2x - y + y²
= (4x² - 4xy + y²) + (2x - y)
= (2x - y)² + (2x - y)
= (2x - y)(2x - y + 1)
e) x³ + 3x² + 3x + 1 - y³
= (x³ + 3x² + 3x + 1) - y³
= (x + 1)³ - y³
= (x + 1 - y)[(x + 1)² + (x + 1)y + y²]
= (x - y + 1)(x² + 2x + 1 + xy + y + y²)
g) x³ - 2x²y + xy² - 4x
= x(x² - 2xy + y² - 4)
= x[(x² - 2xy + y²) - 4]
= x[(x - y)² - 2²]
= x(x - y - 2)(x - y + 2)
TH
1
10 tháng 12 2021
Bài 1:
\(=\left(2x-y\right)\left(4x^2+2xy+y^2\right)\cdot\left(2x-y\right)\left(4x^2-4xy+y^2\right)\)
\(=\left(2x-y\right)^4\cdot\left(4x^2+2xy+y^2\right)\)
10 tháng 10 2021
a (3-2x)2 = 6 - 4x
b (xy+5)2 = 2xy + 10
c (2x+1)(1-2x) = 2x - 4x2 + 1 - 2x = 4x2 + 1
d (1-5x)3 = 3-15x
e (2x+y)(4x2 - 4xy + y2) = 8x3 -8x2y+2xy2 + 4x2y-4xy2 + y3 = 8x3 + y3 - 4x2y - 2xy2
ND
1
27 tháng 7 2021
b) \(\left(4x^2+4xy+y^2\right):\left(2x+y\right)=\dfrac{\left(2x+y\right)^2}{2x+y}=2x+y\)
c) \(\left(x^2-6xy+9y^2\right):\left(3y-x\right)=\dfrac{\left(3y-x\right)^2}{3y-x}=3y-x\)
23 tháng 9 2019
Trog những HĐT trên chắc là
bn đánh máy thiếu số mũ nhỉ??
Phải ko
CC
23 tháng 9 2019
1.\(\left(2x+y\right)\left(4x^2-2xy+y^2\right)-\left(2x-y\right)\left(4x^2+2xy+y^2\right)\)
\(=\left(2x\right)^3+y^3-\left(2x\right)^3+y^3=2y^3\)
2. \(2\left(2x+1\right)\left(3x-1\right)+\left(2x+1\right)^2+\left(3x-1\right)^2\)
\(=\left(2x+1+3x-1\right)^2=\left(5x\right)^2=25x^2\)
3. \(\left(x-y+z\right)^2+\left(z-y\right)^2+2\left(x-y+z\right)\left(y-z\right)\)
\(=\left(x-y+z+y-z\right)^2=x^2\)
4. \(\left(x-3\right)\left(x+3\right)-\left(x-3\right)^2\)
\(=\left(x-3\right)\left(x+3-x+3\right)=6\left(x-3\right)\)
5. \(\left(x^2-1\right)\left(x+2\right)-\left(x-2\right)\left(x^2+2x+4\right)\)
\(=x^3+2x^2-x-2-x^3+y^3=2x^2-x-2+y^3\)
6. Áp dụng các hằng đẳng thức đáng nhớ
17 tháng 9 2021
1: Ta có: \(\left(x+3\right)\left(x^2-3x+9\right)-\left(x^3+54\right)\)
\(=x^3+27-x^3-54\)
=-27
2: Ta có: \(\left(2x+y\right)\left(4x^2-2xy+y^2\right)-\left(2x-y\right)\left(4x^2+2xy+y^2\right)\)
\(=8x^3+y^3-8x^3+y^3\)
\(=2y^3\)
18 tháng 9 2021
\(1,=x^3+270-x^3-54=-27\\ 2,=8x^3+y^3-8x^3+y^3=2y^3\\ 3,=x^3-3x^2+3x-1-x^3-8+3x^2-48=3x-57\\ 4,=x^3-x-x^3-1=-x-1\\ 5,=8x^3-5\left(8x^3+1\right)=-32x^3-5\\ 6,=27+x^3-27=x^3\\ 7,làm.ở.câu.3\\ 8,=x^3-6x^2+12x-8+6x^2-12x+6-x^3-1+3x\\ =3x-3\)
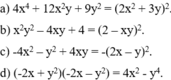
\(\left(2x-y\right)^3-3x.\left(y-2x\right)^2-4x^2+4xy-y^2\)
\(=\left[\left(2x-y\right)^3-3x.\left(y-2x\right)^2\right]-\left[4x^2+4xy-y^2\right]\)
\(=\left[\left(2x-y\right)^2.\left(2x-y\right)-3x.\left(y-2x\right)^2\right]-\left(2x-y\right)^2\)
\(=\left[\left(y-2x\right)^2.\left(2x-y-3x\right)\right]-\left(2x-y\right)^2\)
=\(\left(y-2x\right)^2.\left(-x-y\right)-\left(2x-y\right)^2\)
\(=\left(y-2x\right)^2.\left(-x-y-1\right)\)
Chúc bạn học tốt