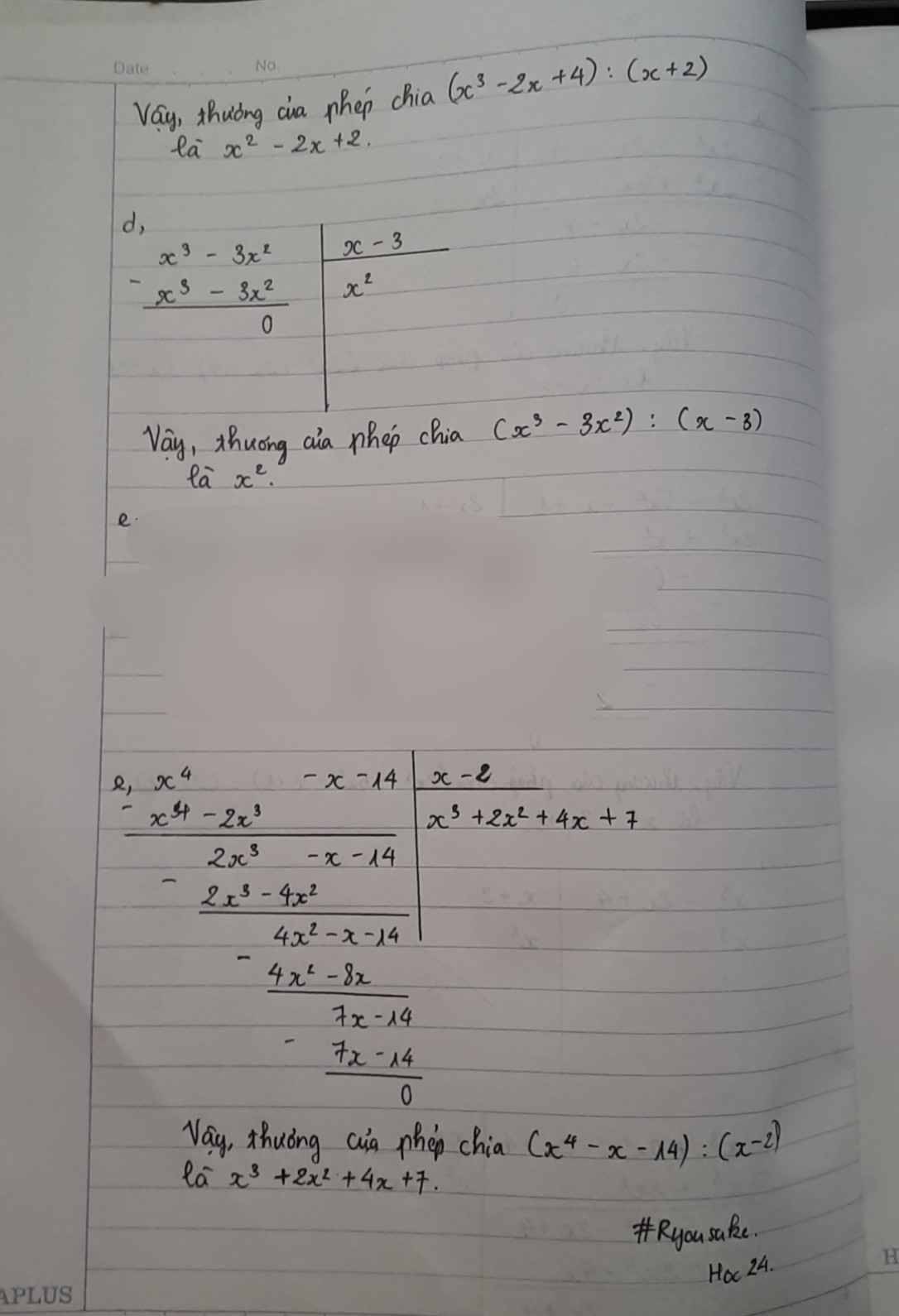Tính: 2 . 2^2x + 4^3 . 2^2x = 642
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PN
1
29 tháng 2 2016
x2 - 4y2 =1
<=>x-2y=1
hoặcx+2y=1
<=>x=1+2y
hoăcx=1-2y=>1+2y=1-2y
<=>4y = 0 <=> y=0=>x=1
OY
7 tháng 9 2021
\(2^x.2^{x+3}=64^2:2^5\)
\(2^x.2^x.2^3=2^{12}:2^5\)
\(2^x.\left(1+8\right)=2^7\)
\(2^x.9=2^7\)
\(\Rightarrow x=\varnothing\)
7 tháng 9 2021
\(2^{2x+3}=64^2:2^5\)
\(\Leftrightarrow2^{2x+3}=2^7\)
hay x=2
TA
21 tháng 7 2021
1) `2x(3x-1)-(2x+1)(x-3)`
`=6x^2-2x-2x^2+6x-x+3`
`=4x^2+3x+3`
2) `3(x^2-3x)-(4x+2)(x-1)`
`=3x^2-9x-4x^2+4x-2x+2`
`=-x^2-7x+2`
3) `3x(x-5)-(x-2)^2-(2x+3)(2x-3)`
`=3x^2-15x-(x^2-4x+4)-(4x^2-9)`
`=3x^2-15x-x^2+4x-4-4x^2+9`
`=-2x^2-11x+5`
4) `(2x-3)^2+(2x-1)(x+4)`
`=4x^2-12x+9+2x^2+8x-x-4`
`=6x^2-5x+5`
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 2 2021
\(A=\dfrac{2tan^2a+\dfrac{5}{cos^2a}}{4-\dfrac{3}{cos^2a}}=\dfrac{2tan^2a+5\left(1+tan^2a\right)}{4-3\left(1+tan^2a\right)}=...\) (bạn tự thay số bấm máy nhé)
\(B=\dfrac{3cot^2a-1}{cot^2a+2}=...\)
BY
0
BY
0
13 tháng 6 2023
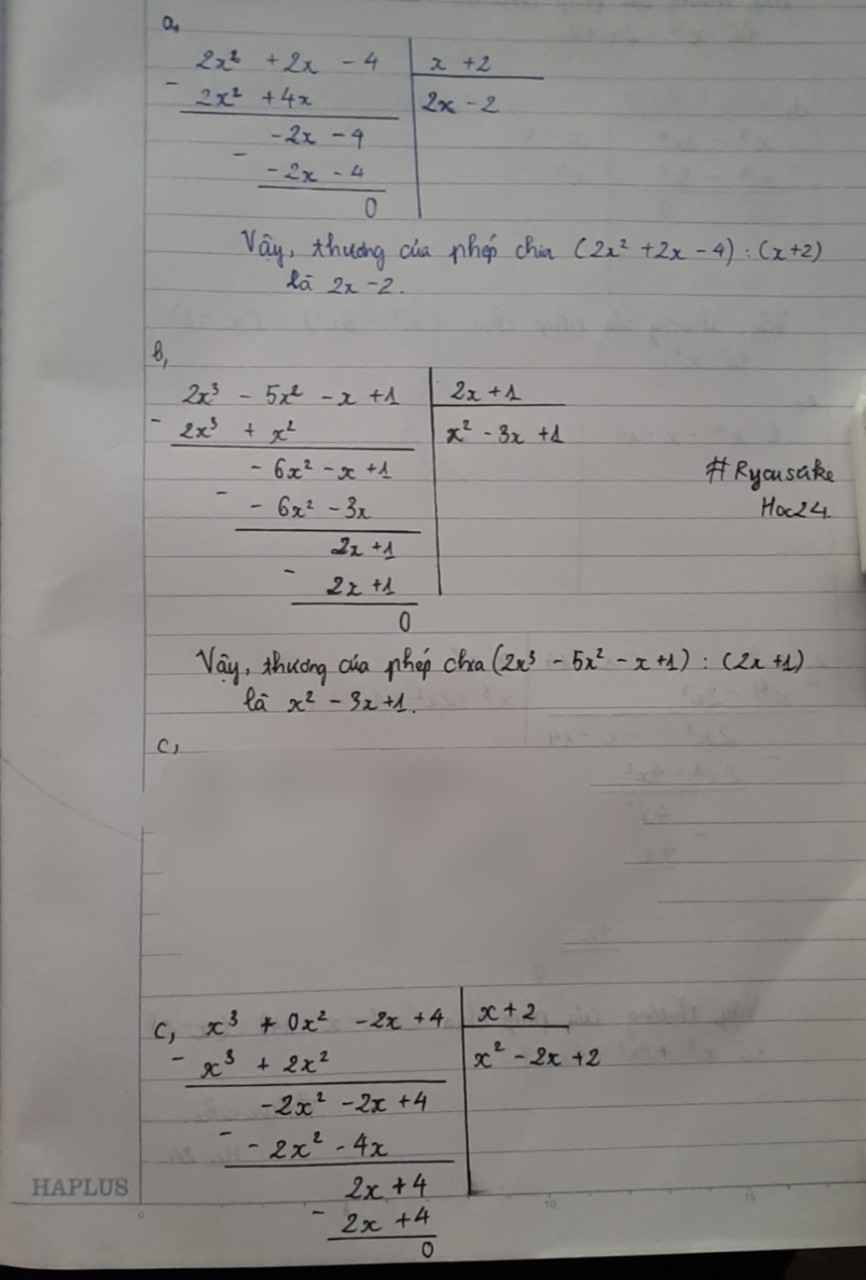
a: \(=\dfrac{2\left(x+2\right)\left(x-1\right)}{x+2}=2x-2\)
b: \(=\dfrac{2x^3+x^2-6x^2-3x+2x+1}{2x+1}=x^2-3x+1\)
c: \(=\dfrac{x^3+2x^2-2x^2-4x+2x+4}{x+2}=x^2-2x+2\)
d: \(=\dfrac{x^2\left(x-3\right)}{x-3}=x^2\)
TT
6 tháng 4 2021
\(a. 2x(3x^2-5x+3) = 6x^3-10x^2+6x \)
\(b. -2x(x^2+5x-3) = -2x^3-10x^2+6x\)
c. \(-\dfrac{1}{2}x^2\left(2x^3-4x+3\right)
=-x^5+2x^3-\dfrac{3}{2}x^2\)
\(d.\left(2x-1\right)\left(x^2+5-4\right)=\left(2x-1\right)\left(x^2+1\right)=2x^3+2x-x^2-1\)
e. \(-\left(5x-4\right)\left(2x+3\right)=10x^2+15x-8x-12=-10x^2+7x-12\)
f.\(\left(2x-y\right)\left(4x^2-2xy+y^2\right)=\left(2x-y\right)\left(2x-y\right)^2=\left(2x-y\right)^3\)
g.\(\left(3x-4\right)\left(x+4\right)+\left(5-x\right)\left(2x^2+3x-1\right)=3x^2+12x-4x-16+10x^2+15x-5-2x^3-3x^2+x=-2x^3+10x^2+24x-21\)
e. \(7x\left(x-4\right)-\left(7x+3\right)\left(2x^2-x+4\right)=7x^2-28x-14x^3+7x^2-28x-6x^2+3x+-12=-14x^3+8x^2-53x-12\)
18 tháng 4 2022
Mình thu gọn 2 đa thức trước r mới cộng nhé
\(P\left(x\right)=3x^2+7+2x^4-3x^2-4-5x+2x^3\)
\(P\left(x\right)=\left(3x^2-3x^2\right)+\left(7-4\right)+2x^4-5x+2x^3\)
\(P\left(x\right)=2x^4+2x^3-5x+3\)
\(Q\left(x\right)=-3x^3+2x^2-x^4+x+x^3+4x-2+5x^4\)
\(Q\left(x\right)=\left(-3x^3+x^3\right)+2x^2+\left(-x^4+5x^4\right)+\left(x+4x\right)-2\)
\(Q\left(x\right)=-2x^3+4x^4+2x^2+5x-2\)
\(P\left(x\right)+Q\left(x\right)=2x^4+2x^3-5x+3-2x^3+4x^4+2x^2+5x-2\)
\(P\left(x\right)+Q\left(x\right)=\left(2x^4+4x^4\right)+\left(2x^3-2x^3\right)+\left(-5x+5x\right)+\left(3-2\right)+2x^2\)
\(P\left(x\right)+Q\left(x\right)=6x^4+1+2x^2\)