Cho đa thức:P(x)=2x3-7x2+5x+1 và Q(x)=2x-1.Tìm các số nguyên x để P(x) chia hết cho Q(x).
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a)\(f\left(x\right)=2x^2-x-3+5=\left(x+1\right)\left(2x-3\right)+5\)
Để \(f\left(x\right)⋮g\left(x\right)\Leftrightarrow\left(x+1\right)\left(2x-3\right)+5⋮\left(x+1\right)\)
\(\Leftrightarrow5⋮\left(x+1\right)\)
mà \(x+1\in Z\Rightarrow x+1\in U\left(5\right)=\left\{-1;1;5;-5\right\}\)
\(\Leftrightarrow x\in\left\{-2;0;4;-6\right\}\)
Vậy...
b) \(f\left(x\right)=3x^2-4x+6=\left(3x^2-4x+1\right)+5=\left(3x-1\right)\left(x-1\right)+5\)
Để \(f\left(x\right)⋮g\left(x\right)\Leftrightarrow\left(3x-1\right)\left(x-1\right)+5⋮\left(3x-1\right)\)
\(\Leftrightarrow5⋮\left(3x-1\right)\) mà \(3x-1\in Z\Rightarrow3x-1\in U\left(5\right)=\left\{-1;1;5;-5\right\}\)
\(\Leftrightarrow x\in\left\{0;\dfrac{2}{3};2;-\dfrac{4}{3}\right\}\) mà x nguyên\(\Rightarrow x\in\left\{0;2\right\}\)
Vậy...
c)\(f\left(x\right)=\left(-2x^3-7x^2-5x+2\right)+3\)\(=\left(-2x^3-4x^2-3x^2-6x+x+2\right)+3\)\(=\left[-2x^2\left(x+2\right)-3x\left(x+2\right)+\left(x+2\right)\right]+3\)
\(=\left(x+2\right)\left(-2x^2-3x+1\right)+3\)
Làm tương tự như trên \(\Rightarrow x+2\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\)
\(\Leftrightarrow x\in\left\{-5;-3;-1;1\right\}\)
Vậy...
d)\(f\left(x\right)=x^3-3x^2-4x+3=x\left(x^2-3x-4\right)+3=x\left(x+1\right)\left(x-4\right)+3\)
Làm tương tự như trên \(\Rightarrow x+1\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\)
\(\Rightarrow x\in\left\{-4;-2;0;2\right\}\)
Vậy...

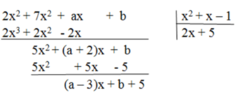
A(x) chia hết cho B(x) khi (a – 3)x + b + 5 là đa thức 0
⇒ a – 3 = 0 hoặc b + 5 = 0 ⇒ a = 3 hoặc b = -5

a)P(x) = 7x3 - x2 + 5x - 2x3 +6 - 8x
=5x^3-x^2-3x+6
Q(x) = -2x + x3 - 4x2 + 3 - 5x2
=x^3-9x^2-2x+3
b)
P(x) - Q(x)=4^3+8x^2-x-3
P(x) + Q(x)=6^3-10x^2-5x+9

a) Thu gọn:
P(x) = x4+(-7x2+4x2)+(x+6x)-2x3-2
P(x) = x4-3x2+7x-2x3-2
Sắp xếp: P(x) = x4-2x3-3x2+7x-2
Thu gọn:
Q(x) = x4+(-3x+x)+(-5x3+6x3)+1
Q(x) = x4-2x+x3+1
Sắp xếp: Q(x)= x4+ x3-2x+1
b/ Nếu x=2, ta có:
P(2) = 24-2.23-3.22+7.2-2
= 16 - 2.8 - 3.4 + 14 -2
= 16-16-12+14-2
= -12+14-2
= 0
=> x=0 là nghiệm của P(x)
Q(2)= 24+ 23-2.2+1
= 16+8-4+1
= 24-4+1
=21
mà 21≠0
Vậy: x=2 không phải là nghiệm của Q(x)
=>

a, \(P\left(x\right)=5x^3-3x+7-x=5x^3-4x+7\)
\(Q\left(x\right)=-5x^3+2x-3+2x-x^2-2=-5x^3-x^2+4x-5\)
b, \(M\left(x\right)=5x^3-4x+7-5x^3-x^2+4x-5=-x^2+2\)
c, Đặt \(M\left(x\right)+2=0\Rightarrow-x^2+4=0\Leftrightarrow x^2=4\Leftrightarrow x=\pm2\)
a: \(P\left(x\right)=5x^3-3x+7-x=5x^3-4x+7\)
\(Q\left(x\right)=-5x^3+2x-3+2x-x^2-2=-5x^3-x^2+4x-5\)
b: Ta có: \(M\left(x\right)=P\left(x\right)+Q\left(x\right)\)
\(=5x^3-4x+7-5x^3-x^2+4x-5\)
\(=-x^2+2\)
c: Đặt M(x)+2=0
\(\Leftrightarrow4-x^2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)

Lời giải:
a. $f(x)=x^4-3x^2+2x-7=x^3(x+2)-2x^2(x+2)+x(x+2)-7$
$=(x+2)(x^3-2x^2+x)-7=g(x)(x^3-2x^2+x)-7$
Vậy $f(x)$ chia $g(x)$ được thương là $x^3-2x^2+x$ và dư là $-7$
b. Theo phần a $f(x)=(x^3-2x^2+x)g(x)-7$
Với $x$ nguyên, để $f(x)\vdots g(x)$ thì $7\vdots g(x)$
$\Leftrightarrow x+2$ là ước của $7$
$\Rightarrow x+2\in\left\{\pm 1;\pm 7\right\}$
$\Leftrightarrow x\in\left\{-3; -1; 5; -9\right\}$
c.
Theo định lý Bezout về phép chia đa thức, để $K(x)=-2x^3+x-m\vdots x+2$ thì: $K(-2)=0$
$\Leftrightarrow -2(-2)^3+(-2)-m=0$
$\Leftrightarrow 14-m=0$
$\Leftrightarrow m=14$

Ta có: \(P\left(x\right)⋮Q\left(x\right)\)
\(\Leftrightarrow2x^3-7x^2+5x+1⋮2x-1\)
\(\Leftrightarrow2x^3-x^2-6x^2+3x+2x-1+2⋮2x-1\)
\(\Leftrightarrow2⋮2x-1\)
\(\Leftrightarrow2x-1\in\left\{1;-1;2;-2\right\}\)
\(\Leftrightarrow2x\in\left\{2;0;3;-1\right\}\)
hay \(x\in\left\{1;0;\dfrac{3}{2};-\dfrac{1}{2}\right\}\)