Giải các phương trình
a) x4 +8x2 -9= 0
b) (x2 -x)2 +4( x2 -x)-12=0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


2:
\(A=\dfrac{x_2-1+x_1-1}{x_1x_2-\left(x_1+x_2\right)+1}\)
\(=\dfrac{3-2}{-7-3+1}=\dfrac{1}{-9}=\dfrac{-1}{9}\)
B=(x1+x2)^2-2x1x2
=3^2-2*(-7)
=9+14=23
C=căn (x1+x2)^2-4x1x2
=căn 3^2-4*(-7)=căn 9+28=căn 27
D=(x1^2+x2^2)^2-2(x1x2)^2
=23^2-2*(-7)^2
=23^2-2*49=431
D=9x1x2+3(x1^2+x2^2)+x1x2
=10x1x2+3*23
=69+10*(-7)=-1

a. (3x - 1)2 - (x + 3)2 = 0
\(\Leftrightarrow\left(3x-1+x+3\right)\left(3x-1-x-3\right)=0\)
\(\Leftrightarrow\left(4x+2\right)\left(2x-4\right)=0\)
\(\Leftrightarrow4x+2=0\) hoặc \(2x-4=0\)
1. \(4x+2=0\Leftrightarrow4x=-2\Leftrightarrow x=-\dfrac{1}{2}\)
2. \(2x-4=0\Leftrightarrow2x=4\Leftrightarrow x=2\)
S=\(\left\{-\dfrac{1}{2};2\right\}\)
b. \(x^3=\dfrac{x}{49}\)
\(\Leftrightarrow49x^3=x\)
\(\Leftrightarrow49x^3-x=0\)
\(\Leftrightarrow x\left(49x^2-1\right)=0\)
\(\Leftrightarrow x\left(7x+1\right)\left(7x-1\right)=0\)
\(\Leftrightarrow x=0\) hoặc \(7x+1=0\) hoặc \(7x-1=0\)
1. x=0
2. \(7x+1=0\Leftrightarrow7x=-1\Leftrightarrow x=-\dfrac{1}{7}\)
3. \(7x-1=0\Leftrightarrow7x=1\Leftrightarrow x=\dfrac{1}{7}\)

`a,x^2 +4x-5=0`
`<=> x^2-x+5x-5=0`
`<=> x(x-1)+5(x-1)=0`
`<=>(x-1)(x+5)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-5\end{matrix}\right.\)
`b, x^2 -x-12=0`
`<=> x^2 +3x-4x-12=0`
`<=>(x^2+3x)-(4x+12)=0`
`<=>x(x+3)-4(x+3)=0`
`<=>(x+3)(x-4)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=4\end{matrix}\right.\)
`c, (2x-7)^2 - 6(2x-7)(x-3)=0`
`<=>(2x-7)(2x-7 -6x+18)=0`
`<=>(2x-7) ( -4x+11)=0`
\(\Leftrightarrow\left[{}\begin{matrix}2x-7=0\\-4x+11=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=7\\-4x=-11\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=\dfrac{11}{4}\end{matrix}\right.\)
a: =>(x+5)(x-1)=0
=>x=1 hoặc x=-5
b: =>(x-4)(x+3)=0
=>x=4 hoặc x=-3
c: =>(2x-7)(2x-7-6x+18)=0
=>(2x-7)(-4x+11)=0
=>x=11/4 hoặc x=7/2

a: Đặt \(a=x^2+x\)
Phương trình ban đầu sẽ trở thành \(a^2+4a-12=0\)
=>\(a^2+6a-2a-12=0\)
=>a(a+6)-2(a+6)=0
=>(a+6)(a-2)=0
=>\(\left(x^2+x+6\right)\left(x^2+x-2\right)=0\)
=>\(x^2+x-2=0\)(Vì \(x^2+x+6=\left(x+\dfrac{1}{2}\right)^2+\dfrac{23}{4}>0\forall x\))
=>\(\left(x+2\right)\left(x-1\right)=0\)
=>\(\left[{}\begin{matrix}x+2=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\)
b:
Sửa đề: \(\left(x^2+2x+3\right)^2-9\left(x^2+2x+3\right)+18=0\)
Đặt \(b=x^2+2x+3\)
Phương trình ban đầu sẽ trở thành \(b^2-9b+18=0\)
=>\(b^2-3b-6b+18=0\)
=>b(b-3)-6(b-3)=0
=>(b-3)(b-6)=0
=>\(\left(x^2+2x+3-3\right)\left(x^2+2x+3-6\right)=0\)
=>\(\left(x^2+2x\right)\left(x^2+2x-3\right)=0\)
=>\(x\left(x+2\right)\left(x+3\right)\left(x-1\right)=0\)
=>\(\left[{}\begin{matrix}x=0\\x+2=0\\x+3=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-2\\x=-3\\x=1\end{matrix}\right.\)
c: \(\left(x-2\right)\left(x+2\right)\left(x^2-10\right)=72\)
=>\(\left(x^2-4\right)\left(x^2-10\right)=72\)
=>\(x^4-14x^2+40-72=0\)
=>\(x^4-14x^2-32=0\)
=>\(\left(x^2-16\right)\left(x^2+2\right)=0\)
=>\(x^2-16=0\)(do x2+2>=2>0 với mọi x)
=>x2=16
=>x=4 hoặc x=-4

d: Ta có: \(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)=24\)
\(\Leftrightarrow\left(x^2+5x+4\right)\left(x^2+5x+6\right)-24=0\)
\(\Leftrightarrow x\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-5\end{matrix}\right.\)


b) x.(x+1). ( x+ 4). (x+ 5) = 12
⇔ [ x. (x + 5)]. [(x+1). (x+ 4)] = 12
⇔ x 2 + 5 x ⋅ x 2 + 4 x + x + 4 − 12 = 0 ⇔ x 2 + 5 x ⋅ x 2 + 5 x + 4 − 12 = 0 ( * )
Đặt t = x 2 + 5 x + 2
= > x 2 + 5 x = t – 2 v à x 2 + 5 x + 4 = t + 2
Khi đó phương trình (*) trở thành:
( t – 2). (t+ 2) - 12 = 0
⇔ t 2 − 4 − 12 = 0 ⇔ t 2 − 16 = 0 ⇔ t 2 = 16 ⇔ t = ± 4
+ Với t = 4 ta có: x 2 + 5 x + 2 = 4
⇔ x 2 + 5 x – 2 = 0 ( * * )
Có a= 1, b = 5, c = - 2 và ∆ = 5 2 – 4 . 1 . ( - 2 ) = 33 > 0
Nên (**) có 2 nghiệm phân biệt là:
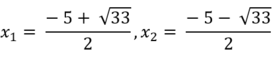
* Với t = - 4 ta có: x 2 + 5 x + 2 = - 4
⇔ x 2 + 5 x + 6 = 0 ( * * * )
Có a= 1, b = 5, c= 6 và ∆ = 5 2 – 4 . 1 . 6 = 1 > 0
Phương trình (***) có 2 nghiệm là:
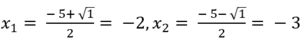
Vậy tập nghiệm của phương trình đã cho là:
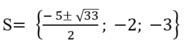


b) x.(x+1). ( x+ 4). (x+ 5) = 12
⇔ [ x. (x + 5)]. [(x+1). (x+ 4)] = 12
⇔ x 2 + 5 x ⋅ x 2 + 4 x + x + 4 − 12 = 0 ⇔ x 2 + 5 x ⋅ x 2 + 5 x + 4 − 12 = 0 ( * )
Đặt t = x 2 + 5 x + 2
= > x 2 + 5 x = t – 2 v à x 2 + 5 x + 4 = t + 2
Khi đó phương trình (*) trở thành:
( t – 2). (t+ 2) - 12 = 0
⇔ t 2 - 4 - 12 = 0 ⇔ t 2 - 16 = 0 ⇔ t 2 = 16 ⇔ t = ± 4
+ Với t = 4 ta có: x 2 + 5 x + 2 = 4
⇔ x2 +5x – 2 = 0 (**)
Có a= 1, b = 5, c = - 2 và ∆ = 5 2 – 4 . 1 . ( - 2 ) = 33 > 0
Nên (**) có 2 nghiệm phân biệt là:
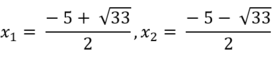
* Với t = - 4 ta có: x 2 + 5 x + 2 = - 4
⇔ x 2 + 5 x + 6 = 0 ( * * * )
Có a= 1, b = 5, c= 6 và ∆ = 5 2 – 4 . 1 . 6 = 1 > 0
Phương trình (***) có 2 nghiệm là:
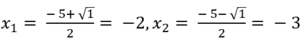
Vậy tập nghiệm của phương trình đã cho là:
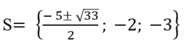

a, \(\Leftrightarrow\left(9x^2-4\right)\left(x+1\right)-\left(3x+2\right)\left(x-1\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(\left(9x^2-4\right)-\left(\left(3x+2\right)\left(x-1\right)\right)\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(9x^2-4-\left(3x^2-x-2\right)\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(9x^2-4-3x^2+x+2\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(3x^2+x-2\right)=0\)
\(\Leftrightarrow\left(x+1\right)=0;3x^2+x-2=0\)
=> x=-1
với \(3x^2+x-2=0\)
ta sử dụng công thức bậc 2 suy ra : \(x=\dfrac{2}{3};x=-1\)
Vậy ghiệm của pt trên \(S\in\left\{-1;\dfrac{2}{3}\right\}\)
b: \(\Leftrightarrow x^2-2x+1-1+x^2=x+3-x^2-3x\)
\(\Leftrightarrow2x^2-2x=-x^2-2x+3\)
\(\Leftrightarrow3x^2=3\)
hay \(x\in\left\{1;-1\right\}\)
c: \(\Leftrightarrow\left(x-1\right)\left(x+1\right)\left(x+2\right)\left(x-3\right)-\left(x-1\right)\left(x-2\right)\left(x+2\right)\left(x+5\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left[\left(x+1\right)\left(x-3\right)-\left(x-2\right)\left(x+5\right)\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left(x^2-2x-3-x^2-3x+10\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left(-5x+7\right)=0\)
hay \(x\in\left\{1;-2;\dfrac{7}{5}\right\}\)
a, Ta có :
x4+8x2-9=0
x4+9x2-x2-9=0
x4-x2+9x2-9=0
x2(x2-1)+9(x2-10=0
(x2-1)(x2+9)=0
\(\Rightarrow x^2-1=0\Rightarrow x=1\)
\(\Rightarrow x^2+9=0\Rightarrow x=-3\)
b, k bt lm
ths