Tính giá trị của biểu thức :\(A=\frac{x-y}{x+y}\)biết x2-2y2=xy và y khác 0, x+y khác 0.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bạn tham khảo bài này nha
Link:https://olm.vn/hoi-dap/detail/266831819020.html
Chúc bạn học tốt

Ta có \(x^2-2y^2=xy\Leftrightarrow x^2-xy-2y^2=0\Leftrightarrow\left(x-2y\right)\left(x+y\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=2y\\x=-y\end{cases}}\)
với x=2y, thao vào, ta có A=1/3
với x=-y thay vào không thỏa mãn
^.^
\(x^2-2y^2=xy\Leftrightarrow x^2-xy-2y^2=0\)
\(\Leftrightarrow x^2+xy-2xy-2y^2=0\)
\(\Leftrightarrow x\left(x+y\right)-2y\left(x+y\right)=0\)
\(\Leftrightarrow\left(x-2y\right)\left(x+y\right)=0\)
\(\Rightarrow x-2y=0\) vì \(x+y\ne0\)
\(\Leftrightarrow x=2y\Rightarrow A=\frac{2y-y}{2y+y}=\frac{1}{3}\)

\(A=\left(x-y\right)\left(x^2-xy\right)-x\left(x^2+2y^2\right)\)
\(=x^3-x^2y-x^2y+xy^2-x^3-2xy^2\)
\(=-2x^2y-xy^2\)
\(=-2\cdot2^2\cdot\left(-3\right)-2\cdot\left(-3\right)^2\)
\(=8\cdot3-2\cdot9\)
=6

\(A=\left(\dfrac{1}{\sqrt{x}+\sqrt{y}}+\dfrac{1}{\sqrt{y}-\sqrt{x}}\right):\dfrac{2\sqrt{xy}}{x-y}\)
\(=\dfrac{\sqrt{x}-\sqrt{y}-\sqrt{x}-\sqrt{y}}{x-y}:\dfrac{2\sqrt{xy}}{x-y}=\dfrac{-2\sqrt{y}}{2\sqrt{xy}}=\dfrac{-1}{\sqrt{x}}=\dfrac{-\sqrt{x}}{x}\)
b, Ta có \(A=\dfrac{-1}{\sqrt{x}}=1\Leftrightarrow\sqrt{x}=-1\left(voli\right)\)
Vậy pt vô nghiệm

\(\dfrac{x+y}{z}+\dfrac{y+z}{x}+\dfrac{x+z}{y}=\dfrac{x^2y+xy^2+y^2z+yz^2+x^2z+xz^2}{xyz}=\dfrac{-3xyz}{xyz}=-3\)
đề cho xy+yz+xz=0 nhân cả 2 vế với -z
=>-xyz-\(z^2\left(y+x\right)\)=0
=>-xyz=\(z^2x+z^2y\)
cmtt bạn nhân với -y và -z
=>-3xyz=\(x^2y+xy^2+y^2z+yz^2+x^2z+xz^2\)
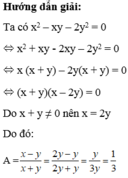
\(x^2-2y^2=xy\Rightarrow x^2-2y^2-xy=0\Rightarrow x^2-y^2-y^2-xy=0\)
\(\Rightarrow\left(x+y\right)\left(x-y\right)-y\left(x+y\right)=0\)
\(\Rightarrow\left(x+y\right)\left(x-2y\right)=0\Rightarrow x-2y=0\)\(\left(x+y\ne0\right)\)
\(\Rightarrow x=2y\)
Thay vào A tính đc giá trị của A