\(x^2_1+x^2_2+x_1x_2\left(x_1+x_2\right)\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
25 tháng 2 2022
\(\Delta'=\left[-\left(m+4\right)\right]^2-1\left(m^2-8\right)=m^2+8m+16-m^2+8=8m+24\)
Để pt có 2 nghiệm thì \(\Delta'\ge0\Leftrightarrow8m+24\ge0\Leftrightarrow m\ge-3\)
Áp dụng định lý Vi-ét ta có:\(\left\{{}\begin{matrix}x_1+x_2=2m+8\\x_1x_2=m^2-8\end{matrix}\right.\)
\(A=x^2_1+x^2_2-x_1-x_2\\ =\left(x_1+x_2\right)^2-2x_1x_2-\left(x_1+x_2\right)\\ =\left(2m+8\right)^2-2\left(m^2-8\right)-\left(2m+8\right)\\ =4m^2+32m+64-2m^2+16-2m-16\\ =2m^2+30m+64\)
Amin=\(-\dfrac{97}{2}\)\(\Leftrightarrow m=-\dfrac{15}{2}\)
\(B=x^2_1+x^2_2-x_1x_2\\ =\left(x_1+x_2\right)^2-3x_1x_2\\ =\left(2m+8\right)^2-3\left(m^2-8\right)\\ =4m^2+32m+64-3m^2+24\\ =m^2+32m+88\)
Bmin=-168\(\Leftrightarrow\)m=-16
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 4 2022
1.
\(a+b+c=0\) nên pt luôn có 2 nghiệm
\(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-1\end{matrix}\right.\)
\(A=\dfrac{2x_1x_2+3}{x_1^2+x_2^2+2x_1x_2+2}=\dfrac{2x_1x_2+3}{\left(x_1+x_2\right)^2+2}=\dfrac{2\left(m-1\right)+3}{m^2+2}=\dfrac{2m+1}{m^2+2}\)
\(A=\dfrac{m^2+2-\left(m^2-2m+1\right)}{m^2+2}=1-\dfrac{\left(m-1\right)^2}{m^2+2}\le1\)
Dấu "=" xảy ra khi \(m=1\)
2.
\(\Delta=m^2-4\left(m-2\right)=\left(m-2\right)^2+4>0;\forall m\) nên pt luôn có 2 nghiệm pb
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-2\end{matrix}\right.\)
\(\dfrac{\left(x_1^2-2\right)\left(x_2^2-2\right)}{\left(x_1-1\right)\left(x_2-1\right)}=4\Rightarrow\dfrac{\left(x_1x_2\right)^2-2\left(x_1^2+x_2^2\right)+4}{x_1x_2-\left(x_1+x_2\right)+1}=4\)
\(\Rightarrow\dfrac{\left(x_1x_2\right)^2-2\left(x_1+x_2\right)^2+4x_1x_2+4}{x_1x_2-\left(x_1+x_2\right)+1}=4\)
\(\Rightarrow\dfrac{\left(m-2\right)^2-2m^2+4\left(m-2\right)+4}{m-2-m+1}=4\)
\(\Rightarrow-m^2=-4\Rightarrow m=\pm2\)
10 tháng 3 2022
\(\Delta'=\left(-2\right)^2-3.\left(-8\right)=4+24=28>0.\)
\(\Rightarrow\) Pt có 2 nghiệm phân biệt \(x_1;x_2.\)
\(\Rightarrow\left\{{}\begin{matrix}x_1=\dfrac{2+2\sqrt{7}}{3}.\\x_2=\dfrac{2-2\sqrt{7}}{3}.\end{matrix}\right.\)
BK
1
11 tháng 2 2023
Giả sử ta định m sao cho pt \(x^2-mx+m-1=0\left(1\right)\) luôn có nghiệm.
Theo định lí Viet ta có: \(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-1\end{matrix}\right.\)
\(C=\dfrac{2x_1x_2+3}{x_1^2+x_2^2+2\left(x_1x_2+1\right)}=\dfrac{2x_1x_2+3}{\left(x_1+x_2\right)^2+2}=\dfrac{2\left(m-1\right)+3}{m^2+2}=\dfrac{2m+1}{m^2+2}\)
\(\Rightarrow C\left(m^2+2\right)=2m+1\Rightarrow Cm^2-2m+\left(2C+1\right)=0\left(2\right)\)
Coi phương trình (2) là phương trình ẩn m tham số C, ta có:
\(\Delta'=1^2-C.\left(2C+1\right)=-2C^2-C+1\)
Để phương trình (2) có nghiệm thì:
\(\Delta'\ge0\Rightarrow-2C^2-C+1\ge0\)
\(\Leftrightarrow\left(2C-1\right)\left(C+1\right)\le0\)
\(\Leftrightarrow-1\le C\le\dfrac{1}{2}\)
Vậy \(MinC=-1;MaxC=\dfrac{1}{2}\)
29 tháng 11 2023
\(x^2-4x-6=0\)
\(\text{Δ}=\left(-4\right)^2-4\cdot1\cdot\left(-6\right)=16+24=40>0\)
=>Phương trình này có hai nghiệm phân biệt
Theo vi-et, ta có:
\(x_1+x_2=\dfrac{-b}{a}=\dfrac{-\left(-4\right)}{1}=4;x_1\cdot x_2=\dfrac{c}{a}=\dfrac{-6}{1}=-6\)
\(A=x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2\)
\(=4^2-2\cdot\left(-6\right)=16+12=28\)
\(B=\dfrac{1}{x_1}+\dfrac{1}{x_2}=\dfrac{x_1+x_2}{x_1\cdot x_2}=\dfrac{4}{-6}=-\dfrac{2}{3}\)
\(C=x_1^3+x_2^3\)
\(=\left(x_1+x_2\right)^3-3\cdot x_1\cdot x_2\cdot\left(x_1+x_2\right)\)
\(=4^3-3\cdot4\cdot\left(-6\right)=64+72=136\)
\(D=\left|x_1-x_2\right|\)
\(=\sqrt{\left(x_1-x_2\right)^2}\)
\(=\sqrt{\left(x_1+x_2\right)^2-4x_1x_2}\)
\(=\sqrt{4^2-4\cdot\left(-6\right)}=\sqrt{16+24}=\sqrt{40}=2\sqrt{10}\)
20 tháng 1 2017
Ta có để pt có 2 nghiệm phân biệt thì:
\(\Delta'=\left(m-2\right)^2-\left(m^2-2m\right)>0\)
\(\Leftrightarrow m< 2\)
Theo vi-et ta có
\(\hept{\begin{cases}x_1+x_2=4-2m\\x_1x_2=m^2-2m\end{cases}}\)
Theo đề ta có: \(\frac{2}{x_1^2+x_2^2}-\frac{1}{x_1x_2}=\frac{1}{15m}\)
\(\Leftrightarrow\frac{2}{\left(x_1+x_2\right)^2-4x_1x_2}-\frac{1}{x_1x_2}=\frac{1}{5m}\)
\(\Leftrightarrow\frac{2}{\left(4-2m\right)^2-4\left(m^2-2m\right)}-\frac{1}{m^2-2m}=\frac{1}{15m}\)
\(\Leftrightarrow\frac{1}{8-4m}-\frac{1}{m^2-2m}=\frac{1}{15m}\)
\(\Leftrightarrow19m+52=0\)
\(\Leftrightarrow m=\frac{52}{19}\)(loại)
Không có m thỏa cái trên
PS: Không biết có nhầm chỗ nào không. Bạn kiểm tra hộ m nhé
3 tháng 6 2017
Xét pt (1) có:
\(\Delta=\left(-2m\right)^2-4\left(m-2\right)\)
= \(4m^2-4m+8\)
= \(\left(2m-1\right)^2+7>0\)
\(\Rightarrow\) Pt (1) luôn có 2 nghiệm phân biệt với mọi m
Áp dụng hệ thức Vi-ét ta có:
\(\left\{{}\begin{matrix}x_1+x_2=2m\\x_1.x_2=m-2\end{matrix}\right.\)
Theo đề bài ta có:
\(\left(1+x_1\right)\left(2-x_2\right)+\left(1+x_2\right)\left(2-x_1\right)=x_1^2+x_2^2+2\)
\(\Leftrightarrow2-x_2+2x_1-x_1x_2+2-x_1+2x_2-x_1x_2=\left(x_1+x_2\right)^2-2x_1x_2+2\) \(\Leftrightarrow-\left(x_1+x_2\right)+2\left(x_1+x_2\right)+2-\left(x_1+x_2\right)^2=0\)
\(\Leftrightarrow-\left(x_1+x_2\right)\left[1-2+\left(x_1+x_2\right)\right]+2=0\)
\(\Leftrightarrow-2m\left(2m-1\right)+2=0\)
\(\Leftrightarrow-4m^2+2m+2=0\)
\(\Leftrightarrow\left(m-1\right)\left(2m+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m-1=0\\2m+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}m=1\\m=\dfrac{-1}{2}\end{matrix}\right.\)
Vậy để pt (1) có 2 nghiệm \(x_1,x_2\) thỏa mãn \(\left(1+x_1\right)\left(2-x_2\right)+\left(1+x_2\right)\left(2-x_1\right)=x_1^2+x_2^2+2\) thì \(m=1\) hoặc \(m=\dfrac{-1}{2}\)
3 tháng 6 2017
\(\Delta\)' = m2 - m + 2 = m2 - 2.m.\(\dfrac{1}{2}\) + \(\dfrac{1}{4}\) - \(\dfrac{1}{4}\) + 2 = \(\left(m-\dfrac{1}{2}\right)^2\) + \(\dfrac{7}{4}\) \(\ge\) \(\dfrac{7}{4}\) > 0
\(\Rightarrow\) phương trình luôn có 2 nghiệm \(\forall\)m
áp dụng hệ thức vi ét ta có : \(\left\{{}\begin{matrix}x_1+x_2=2m\\x_1.x_2=m-2\end{matrix}\right.\)
(1 + x1)(2 - x2) + (1 + x2)(2 - x1) = x12 + x22 + 2
2 - x2 + 2x1 - x1x2 + 2 - x1 + 2x2 - x1x2 = (x1 + x2)2 - 2x1x2 + 2
= (x1 + x2)2 - (x1 + x2) - 2 = 0
thay vào ta có : (2m)2 - 2m - 2 = 0
4m2 - 2m - 2 = 0 ta có : a + b + c = 4 - 2 - 2 = 0
\(\Rightarrow\) phương trình có 2 nghiệm phân biệt
m1 = 1 ; m2 = \(\dfrac{c}{a}\) = \(-\dfrac{1}{2}\)
vậy m = 1 ; m = \(-\dfrac{1}{2}\) thảo mảng điều kiện bài toán
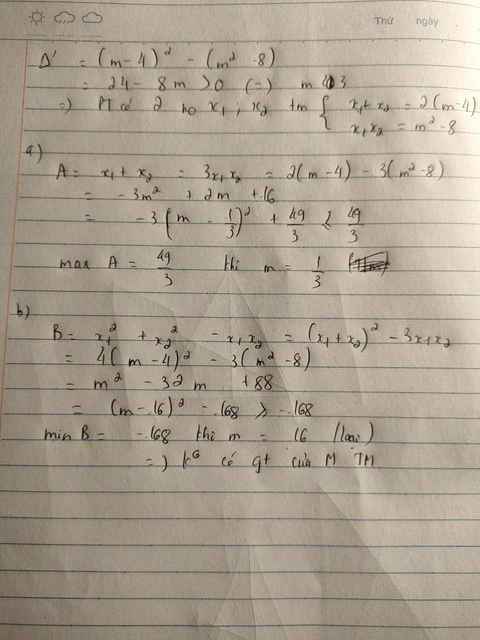
phương trình đâu bạn??
Theo hệ thức Vi-et : \(x_1+x_2=\dfrac{-b}{a}\) và \(x_1x_2=\dfrac{c}{a}\)
\(x_1^2+x_2^2+x_1x_2\left(x_1+x_2\right)\)
=\(\left(x_1+x_2\right)^2-2x_1x_2+x_1x_2\left(x_1+x_2\right)\)
=\(\left(\dfrac{-b}{a}\right)^2-2\dfrac{c}{a}+\dfrac{c}{a}\left(\dfrac{-b}{a}\right)\)
=\(\dfrac{b^2}{a^2}-\dfrac{2c}{a}+\dfrac{-bc}{a^2}\)
=\(\dfrac{b^2-2ac-bc}{a^2}\)