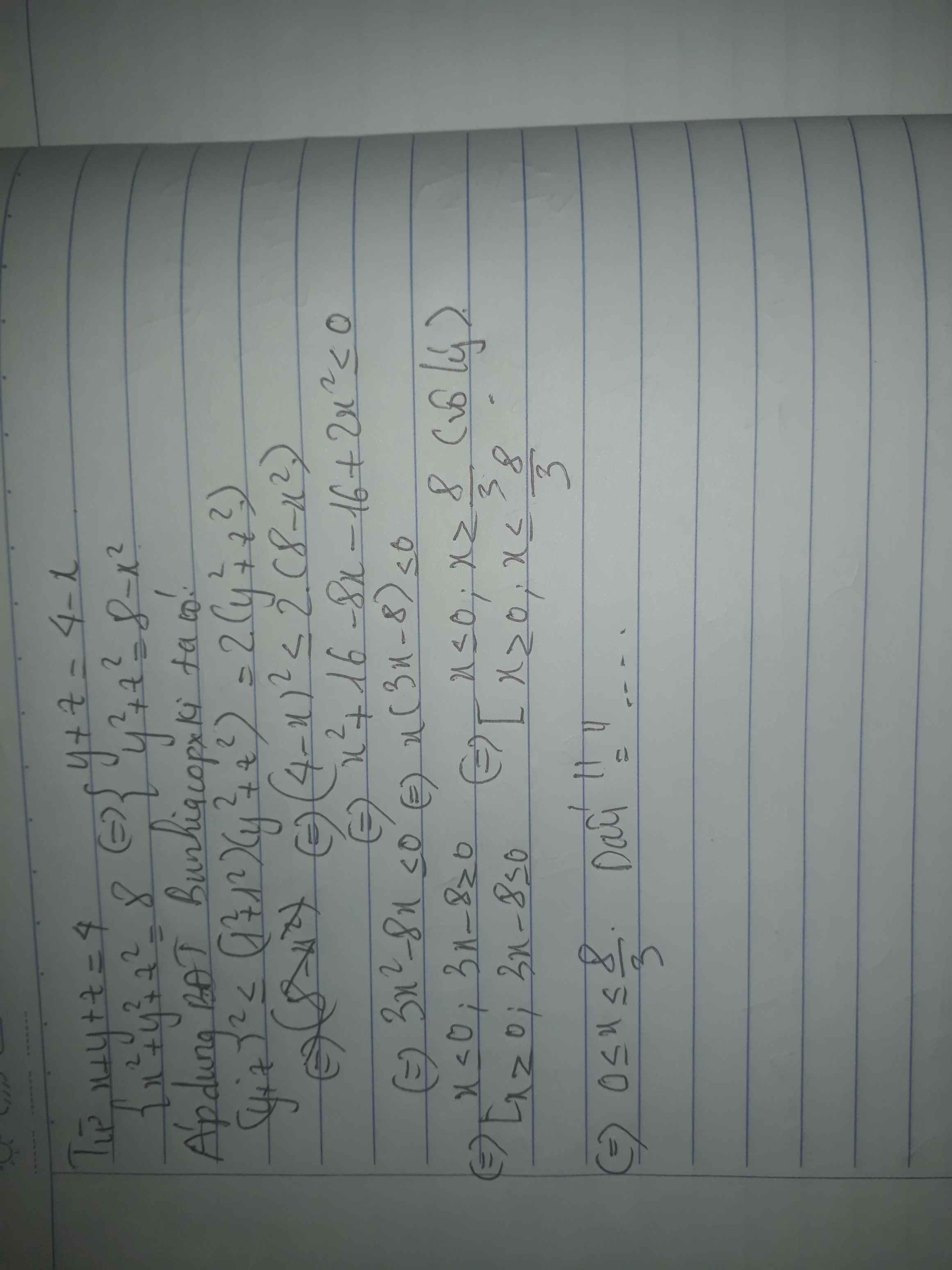Biết \(0\le x\le y\le z\). Tìm nghiệm nguyên của PT: \(2^x+2^y+2^z=1024\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Xét x=0,x=1 thì thỏa mãn
Xét x khác 0,1
Dùng phản chứng là ra mà "<<
Với mọi số nguyên n ta có n <= n2 . Do đó từ đề bài suy ra :
x2 <= y <= y2 <= z <= z2 <= x <= x2.
Do đó x^2 = y = y^2 = z = z^2 = x = x^2.
Ta có : x^2 = x <=> x(x-1) = 0 <=> x = 0 và x = 1
Tương tự như thế
Vậy : ...

cj tìm ra nghiệm r e! x=y=z=1 . Nhưg mà vẫn chưa giải ra đc
Vì \(x,y,z\in\left[0;1\right]\) nên \(\left(x-1\right)\left(z-1\right)\ge0\Leftrightarrow xz+1\ge x+z\)
\(\Rightarrow xz+1+y\ge x+y+z\) \(\Rightarrow\dfrac{x}{1+y+zx}\le\dfrac{x}{x+y+z}\)
Tương tự ta có:
\(\dfrac{x}{1+y+xz}+\dfrac{y}{1+z+xy}+\dfrac{z}{1+x+yz}\le\dfrac{x}{x+y+z}+\dfrac{y}{x+y+z}+\dfrac{z}{z+y+z}=1\)
hay \(\dfrac{3}{x+y+z}\le1\Leftrightarrow x+y+z\ge3\)
Mà \(x;y;z\in\left[0;1\right]\Rightarrow x+y+z\le3\)
\(\Rightarrow x+y+z=3\) và \(x=y=z=1\)

Do \(x\in\left[-1;2\right]\Rightarrow\)\(\left(x+1\right)\left(x-2\right)\le0\Leftrightarrow x^2\le x+2\)
Tương tự: \(y^2\le y+2\) ; \(z^2\le z+2\)
Cộng vế: \(x^2+y^2+z^2\le x+y+z+6=6\) (đpcm)
Mặt khác \(x;y;z\in\left[-1;2\right]\Rightarrow\left(x+1\right)\left(y+1\right)\left(z+1\right)\ge0\)
\(\Leftrightarrow xyz+xy+yz+zx+x+y+z+1\ge0\)
\(\Leftrightarrow xyz+xy+yz+zx+1\ge0\)
\(\Leftrightarrow2xyz+2\ge-2\left(xy+yz+zx\right)\)
\(\Leftrightarrow2xyz+2\ge\left(x^2+y^2+z^2\right)-\left(x+y+z\right)^2\)
\(\Leftrightarrow2xyz+2\ge x^2+y^2+z^2\) (đpcm)

Gợi ý :
Bài 3 :
\(5\left(x^2+2x+1\right)+2\left(y^2+2y+1\right)=13\)
\(\Leftrightarrow5\left(x+1\right)^2+2\left(y+1\right)^2=13\)
Bài 2 :
GTLN: Do a,b tự nhiên nên a,b > 0
Áp dụng Cô si ta có :
\(ab\le\frac{\left(a+b\right)^2}{4}=\frac{n^2}{4}\)

\(c,P=\dfrac{x^2-x^2+8xy-16y^2}{x^2+4y^2}=\dfrac{8\left(\dfrac{x}{y}\right)-16}{\left(\dfrac{x}{y}\right)^2+4}\)
Đặt \(\dfrac{x}{y}=t\)
\(\Leftrightarrow P=\dfrac{8t-16}{t^2+4}\Leftrightarrow Pt^2+4P=8t-16\\ \Leftrightarrow Pt^2-8t+4P+16=0\)
Với \(P=0\Leftrightarrow t=2\)
Với \(P\ne0\Leftrightarrow\Delta'=16-P\left(4P+16\right)\ge0\)
\(\Leftrightarrow-P^2-4P+4\ge0\Leftrightarrow-2-2\sqrt{2}\le P\le-2+2\sqrt{2}\)
Vậy \(P_{max}=-2+2\sqrt{2}\Leftrightarrow t=\dfrac{4}{P}=\dfrac{4}{-2+2\sqrt{2}}=2+\sqrt{2}\)
\(\Leftrightarrow\dfrac{x}{y}=2+2\sqrt{2}\)