Tìm giá trị nhỏ nhất: A= x2 - 12x +34
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có
F = x 2 – 12 x + 34 = x 2 – 2 . x . 6 + 6 2 – 2 = x – 6 2 – 2
Vì x – 6 2 ≥ 0; Ɐx => x – 6 2 – 2 ≥ - 2
Dấu “=” xảy ra khi x – 6 2 = 0 ó x – 6 = 0 ó x = 6
Vậy giá trị nhỏ nhất của E là -2 khi x = 6
Đáp án cần chọn là: A

A=x2−2xy+6y2−12x+2y+45A=x2−2xy+6y2−12x+2y+45
=(x2−2xy+y2−12x+12y+36)+(5y2−10y+5)+4=(x2−2xy+y2−12x+12y+36)+(5y2−10y+5)+4
=[(x−y)2−12(x+y)+62]+5(y2−2y+1)+4=[(x−y)2−12(x+y)+62]+5(y2−2y+1)+4
=(x−y+6)

\(A=x^2-4x+20=x^2-4x+4+16=\left(x-2\right)^2+16\)
Do \(\left(x-2\right)^2\ge0\)
\(\Rightarrow\left(x-2\right)^2+16\ge16\)
\(\Rightarrow Min\left(A\right)=16\)
\(B=x^2-3x+7=x^2-3x+\dfrac{9}{4}-\dfrac{9}{4}+7=\left(x-\dfrac{3}{2}\right)^2+\dfrac{19}{4}\)
Do \(\left(x-\dfrac{3}{2}\right)^2\ge0\)
\(\Rightarrow\left(x-\dfrac{3}{2}\right)^2+\dfrac{19}{4}\ge\dfrac{19}{4}\)
\(\Rightarrow Min\left(B\right)=\dfrac{19}{4}\)
\(C=-x^2-10x+70=-\left(x^2+10x+25\right)+25+70=-\left(x-5\right)^2+95\)
Do \(-\left(x-5\right)^2\le0\)
\(\Rightarrow-\left(x-5\right)^2+95\le95\)
\(\Rightarrow Max\left(C\right)=95\)
\(D=-4x^2+12x+1=-\left(4x^2-12x+9\right)+9+1=-\left(2x-3\right)^2+10\)
Do \(-\left(2x-3\right)^2\le0\)
\(\Rightarrow-\left(2x-3\right)^2+10\le10\)
\(\Rightarrow Max\left(D\right)=10\)

a: =>x^3+2x^2-8x^2-16x+15x+30=0
=>(x+2)(x^2-8x+15)=0
=>(x+2)(x-3)(x-5)=0
=>\(x\in\left\{-2;3;5\right\}\)
b: =x^2-12x+36-3
=(x-6)^2-3>=-3
Dấu = xảy ra khi x=6

a) Ta có: \(25x^2-20x+7\)
\(=\left(5x\right)^2-2\cdot5x\cdot2+4+3\)
\(=\left(5x-2\right)^2+3\ge3\forall x\)
Dấu '=' xảy ra khi \(x=\dfrac{2}{5}\)
b) Ta có: \(9x^2-6x+2\)
\(=9x^2-6x+1+1\)
\(=\left(3x-1\right)^2+1\ge1\forall x\)
Dấu '=' xảy ra khi \(x=\dfrac{1}{3}\)
c) Ta có: \(-x^2+2x-2\)
\(=-\left(x^2-2x+2\right)\)
\(=-\left(x^2-2x+1+1\right)\)
\(=-\left(x-1\right)^2-1\le-1\forall x\)
Dấu '=' xảy ra khi x-1=0
hay x=1
d) Ta có: \(x^2+12x+39\)
\(=x^2+12x+36+3\)
\(=\left(x+6\right)^2+3\ge3\forall x\)
Dấu '=' xảy ra khi x=-6
e) Ta có: \(-x^2-12x\)
\(=-\left(x^2+12x+36-36\right)\)
\(=-\left(x+6\right)^2+36\le36\forall x\)
Dấu '=' xảy ra khi x=-6
f) Ta có: \(4x-x^2+1\)
\(=-\left(x^2-4x-1\right)\)
\(=-\left(x^2-4x+4-5\right)\)
\(=-\left(x-2\right)^2+5\le5\forall x\)
Dấu '=' xảy ra khi x=2

a) Ta có: \(25x^2-20x+7\)
\(=\left(5x\right)^2-2\cdot5x\cdot2+4+3\)
\(=\left(5x-2\right)^2+3\ge3\forall x\)
Dấu '=' xảy ra khi \(x=\dfrac{2}{5}\)
b) Ta có: \(9x^2-6x+2\)
\(=9x^2-6x+1+1\)
\(=\left(3x-1\right)^2+1\ge1\forall x\)
Dấu '=' xảy ra khi \(x=\dfrac{1}{3}\)
c) Ta có: \(-x^2+2x-2\)
\(=-\left(x^2-2x+2\right)\)
\(=-\left(x^2-2x+1+1\right)\)
\(=-\left(x-1\right)^2-1\le-1\forall x\)
Dấu '=' xảy ra khi x=1
( Mình trình bày mẫu câu a các câu khác mình làm tắt lại nhưng tương tự trình bày câu a nha )
a, Ta có : \(25x^2-20x+7=\left(5x\right)^2-2.5x.2+2^2+3\)
\(=\left(5x-2\right)^2+3\)
Thấy : \(\left(5x-2\right)^2\ge0\forall x\in R\)
\(\Rightarrow\left(5x-2\right)^2+3\ge3\forall x\in R\)
Vậy \(Min=3\Leftrightarrow5x-2=0\Leftrightarrow x=\dfrac{2}{5}\)
b, \(=9x^2-2.3x+1+1=\left(3x-1\right)^2+1\ge1\)
Vậy Min = 1 <=> x = 1/3
c, \(=-x^2+2x-1-1=-\left(x^2-2x+1\right)-1=-\left(x-1\right)^2-1\le-1\)
Vậy Max = -1 <=> x = 1
d, \(=x^2+2.x.6+36+3=\left(x+6\right)^2+3\ge3\)
Vậy Min = 3 <=> x = - 6
e, \(=-x^2-2.x.6-36+36=-\left(x+6\right)^2+36\le36\)
Vậy Max = 36 <=> x = -6 .
f, \(=-x^2+4x-4+5=-\left(x^2-4x+4\right)+5=-\left(x-2\right)^2+5\le5\)
Vậy Max = 5 <=> x = 2
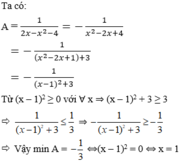
A = (x^2-12x+36) - 2
= (x-6)^2 - 2
>= -2
Dấu "=" xảy ra <=> x-6=0 <=> x=6
Vậy GTNN của A = -2 <=> x=6
Tk mk nha