1+1=? các cao nhân giúp với
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(y\left(x-1\right)=x^2+2\)
\(\Leftrightarrow x^2-xy+y+2=0\)
\(\Leftrightarrow x\left(x-1\right)-y\left(x-1\right)+\left(x-1\right)+3=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-y+1\right)=-3\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-1=-1\\x-y+1=3\end{matrix}\right.\\\left\{{}\begin{matrix}x-1=3\\x-y+1=-1\end{matrix}\right.\\\left\{{}\begin{matrix}x-1=1\\x-y+1=-3\end{matrix}\right.\\\left\{{}\begin{matrix}x-1=-3\\x-y+1=1\end{matrix}\right.\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x=0\\y=-2\end{matrix}\right.\\\left\{{}\begin{matrix}x=4\\y=6\end{matrix}\right.\\\left\{{}\begin{matrix}x=2\\y=6\end{matrix}\right.\\\left\{{}\begin{matrix}x=-2\\y=-2\end{matrix}\right.\end{matrix}\right.\)
Vậy \(\left(x;y\right)\in\left\{\left(0;-2\right),\left(4;6\right),\left(2;6\right),\left(-2;-2\right)\right\}\)
Ta có \(y\left(x-1\right)=x^2+2\)
\(\Leftrightarrow y\left(x-1\right)-x^2=2\)
\(\Leftrightarrow y\left(x-1\right)-x^2+1=3\)
\(\Leftrightarrow y\left(x-1\right)-\left(x^2-1\right)=3\)
\(\Leftrightarrow y\left(x-1\right)-\left(x-1\right)\left(x+1\right)=3\)
\(\Leftrightarrow\left(x-1\right)\left(y-x-1\right)=3\)
Vì x,y nguyên nên ta có bảng
| x-1 | 3 | 1 | -1 | -3 |
| y-x-1 | 1 | 3 | -3 | -1 |
| x | 4 | 2 | 0 | -2 |
| y | 6 | 8 | 2 | 4 |
Vậy\(\left(x,y\right)=\left\{\left(4,6\right),\left(2,8\right),\left(0,2\right),\left(-2,4\right)\right\}\)thỏa mãn

Đặt \(y=\sqrt[3]{\dfrac{23+\sqrt{513}}{4}}+\sqrt[3]{\dfrac{23-\sqrt{513}}{4}}\) ( bạn lập phương cả 2 vế nhé )
\(\Leftrightarrow2y^3=6y+23\left(1\right)\)
theo đề bài,ta có: \(x=\dfrac{1}{3}\left(y-1\right)\)
\(\Leftrightarrow3x=y-1\Leftrightarrow y=3x+1\left(2\right)\Leftrightarrow2y^3=54x^3+54x^2+18x+2\left(3\right)\)
Thế (2) và (3) vào (1)
\(\Leftrightarrow54x^3+54x^2+18x+2=6\left(3x+1\right)+23\)
\(\Leftrightarrow54x^3+54x^2+18x+2=18x+6+23\)
\(\Leftrightarrow54x^3+54x^2=27\)
\(\Leftrightarrow2x^3+2x^2=1\)
\(A=2x^3+2x^2+1\)
\(A=1+1=2\)


Bài 1.
a) ĐKXĐ: \(x^2-1\ne0\Leftrightarrow x\ne\pm1\)
b) Để \(B=2\) thì \(\dfrac{x^2+2x+1}{x^2-1}=2\)
\(\Leftrightarrow\dfrac{\left(x+1\right)^2}{\left(x-1\right)\left(x+1\right)}=2\)
\(\Leftrightarrow\dfrac{x+1}{x-1}=2\)
\(\Rightarrow x+1=2x-2\)
\(\Leftrightarrow2x-x=1+2\)
\(\Leftrightarrow x=3\left(tm\right)\)
Bài 2.
a) ĐKXĐ: \(x+2\ne0\Leftrightarrow x\ne-2\)
b) Với \(x=1\Rightarrow\) thoả mãn đk \(x\ne-2\)
Ta có: \(C=\dfrac{x^2+4x+4}{x+2}=\dfrac{\left(x+2\right)^2}{x+2}=x+2\)
Thay \(x=1\) vào \(C\), ta được:
\(C=1+2=3\)
c) Để \(C=1\) thì \(x+2=1\Leftrightarrow x=-1\left(tm\right)\)
\(\text{#}Toru\)

3:
b: x1^2+x2^2=12
=>(x1+x2)^2-2x1x2=12
=>(2m+2)^2-4m=12
=>4m^2+4m+4=12
=>m^2+m+1=3
=>(m+2)(m-1)=0
=>m=1;m=-2
2:
b: =>|x1|-|x2|=m+3-|-1|=m+2
=>x1^2+x2^2-2|x1x2|=m+2
=>(x1+x2)^2-2x1x2-2|x1x2|=m+2
=>(2m)^2-2(-1)-2|-1|=m+2
=>4m^2-m-2=0
=>m=(1+căn 33)/8; m=(1-căn 33)/8
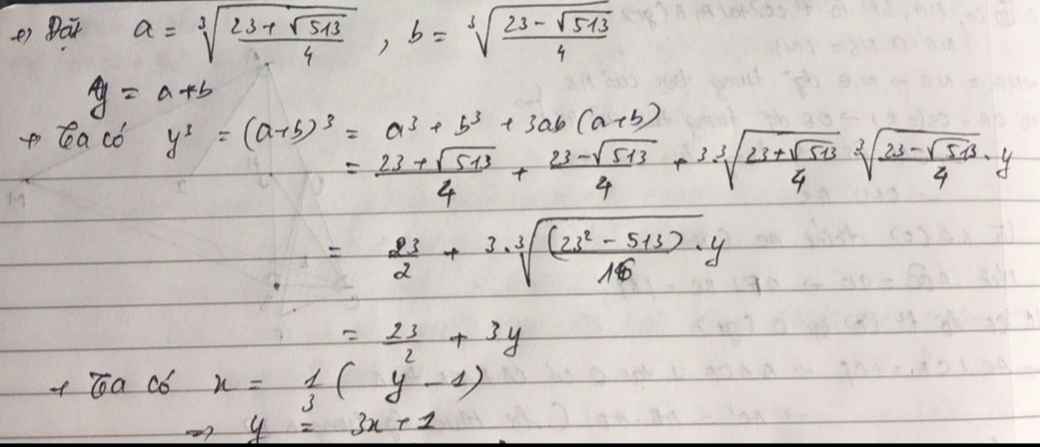

 các cao nhân giúp em với
các cao nhân giúp em với



= 2 nha
1 + 1 = 2
K nha