Cho hàm số y=f(x) = 5.x Tìm x biết f(x)=0; f(x)=1; f(x)=-5; f(x)=2005
Cho hàm số y=f(x)=a.x - 3 Tìm a nếu biết f(3)=9
GIÚP MIK VS! MIK CẦN CÂU TRẢ LỜI GẤP! > . <
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
22 tháng 2 2021
Câu 1:
a)
| \(y=f\left(x\right)=2x^2\) | -5 | -3 | 0 | 3 | 5 |
| f(x) | 50 | 18 | 0 | 18 | 50 |
b) Ta có: f(x)=8
\(\Leftrightarrow2x^2=8\)
\(\Leftrightarrow x^2=4\)
hay \(x\in\left\{2;-2\right\}\)
Vậy: Để f(x)=8 thì \(x\in\left\{2;-2\right\}\)
Ta có: \(f\left(x\right)=6-4\sqrt{2}\)
\(\Leftrightarrow2x^2=6-4\sqrt{2}\)
\(\Leftrightarrow x^2=3-2\sqrt{2}\)
\(\Leftrightarrow x=\sqrt{3-2\sqrt{2}}\)
hay \(x=\sqrt{2}-1\)
Vậy: Để \(f\left(x\right)=6-4\sqrt{2}\) thì \(x=\sqrt{2}-1\)
VP
23 tháng 12 2018
a ) Ta có : f(2) = 5
\(\Leftrightarrow\hept{\begin{cases}f\left(x\right)=f\left(2\right)\\\text{ax}-3=5\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=2\\a.2-3=5\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=2\\a=4\end{cases}}\)
Vậy a = 4
b ) Ta có : f(0) = 3
\(\Leftrightarrow\hept{\begin{cases}f\left(x\right)=f\left(0\right)\\\text{ax}+b=3\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=0\\a.0+b=3\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=0\\b=3\end{cases}}\) ( 1 )
Ta có : f ( 1 ) = 4
\(\Leftrightarrow\hept{\begin{cases}f\left(x\right)=f\left(1\right)\\\text{ax}+b=4\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=1\\a.1+b=4\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=1\\a+b=4\end{cases}}\) ( 2 )
Thay b = 3 ở ( 1 ) vào a+b=4 ở ( 2 ) ta được : a + 3 = 4
a = 1
Vậy a = 1 ; b = 3
Q
0
B
2
25 tháng 12 2021
\(a,f\left(-1\right)=3-\left(-1\right)=4\\ f\left(2\right)=3-2=1\\ b,y=5\Rightarrow3-x=5\Rightarrow x=-2\\ y=2\Rightarrow3-x=2\Rightarrow x=1\\ y=-1\Rightarrow3-x=-1\Rightarrow x=4\)
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 8 2021
\(y'=2f'\left(x\right).f'\left(f\left(x\right)\right)-2f'\left(x\right).f\left(x\right)\)
\(y'=0\Rightarrow\left[{}\begin{matrix}f'\left(x\right)=0\\f'\left(f\left(x\right)\right)=f\left(x\right)\end{matrix}\right.\)
Từ đồ thị ta có \(f'\left(x\right)=0\Rightarrow x=x_1\) với \(-4< x_1< 0\)
Xét phương trình \(f'\left(f\left(x\right)\right)=f\left(x\right)\), đặt \(f\left(x\right)=t\Rightarrow f'\left(t\right)=t\)
Vẽ đường thẳng \(y=t\) (màu đỏ) lên cùng đồ thị \(y=f'\left(t\right)\) như hình vẽ:
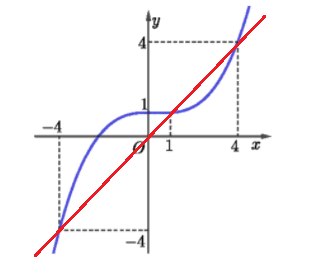
Ta thấy 2 đồ thị cắt nhau tại 3 điểm: \(t=\left\{-4;1;4\right\}\)
\(\Rightarrow\left[{}\begin{matrix}f\left(x\right)=-4\\f\left(x\right)=1\\f\left(x\right)=4\end{matrix}\right.\) (1)
Mặt khác từ đồ thị \(f'\left(x\right)\) và \(f\left(0\right)=-4\) ta được BBT của \(f\left(x\right)\) có dạng:
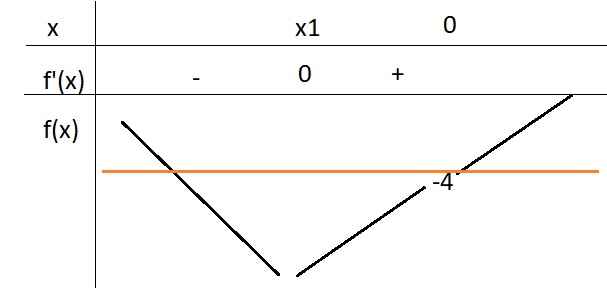
Từ đó ta thấy các đường thẳng \(y=k\ge-4\) luôn cắt \(y=f\left(x\right)\) tại 2 điểm phân biệt
\(\Rightarrow\) Hệ (1) có 6 nghiệm phân biệt (trong đó 3 nghiệm nhỏ hơn \(x_1\) và 3 nghiệm lớn hơn \(x_1\))
Từ đó ta có dấu của y' như sau:
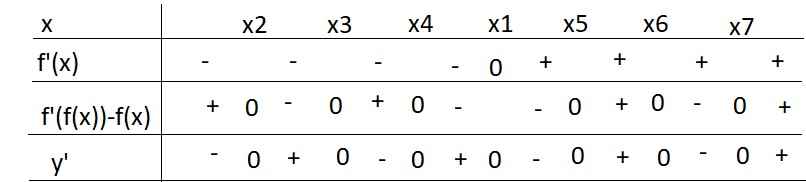
Có 3 lần y' đổi dấu từ dương sang âm nên hàm có 3 cực đại
23 tháng 12 2021
a) Cho hàm số y = f(x) = -2x + 3.
Ta có: f(-2)= -2.(-2)+3
= 4+3=7
Ta có: f(0)= -2.0+3
= 0+3=3
Ta có: f(\(\dfrac{-1}{2}\))= -2.(-\(\dfrac{1}{2}\))+3
=\(\dfrac{-2.\left(-1\right)}{2}\)+3
=\(\dfrac{2}{2}\)+3
= 1+3= 4
Vậy f(-2)=7;f(0)=3;f( \(\dfrac{-1}{2}\))=4
b) Cho hàm số y = f(x) = -2x + 3
mà f(x)=5
Suy ra: f(x) = -2x + 3=5
hay -2x + 3=5
-2x=5-3
-2x=2
x=2:(-2)
x= -1
Cho hàm số y = f(x) = -2x + 3
mà f(x)=1
Suy ra: f(x) = -2x + 3=1
hay -2x + 3=1
-2x=1-3
-2x= -2
x= -2:(-2)
x=1
Vậy f(x)=5 thì x= -1 và f(x) = 1 thì x=1.
AH
Akai Haruma
Giáo viên
23 tháng 12 2021
Lời giải:
a.
$f(-2)=(-2)(-2)+3=7$
$f(0)=(-2).0+3=3$
$f(\frac{-1}{2})=(-2).\frac{-1}{2}+3=4$
b.
$f(x)=-2x+3=5$
$\Rightarrow -2x=2$
$\Rightarrow x=-1$
$f(x)=-2x+3=1$
$\Rightarrow -2x=1-3=-2$
$\Rightarrow x=1$
F
1
16 tháng 1 2022
f(x)=0 =>5x=0
hay x=0
f(x)=1 =>5x=1
=>x=1/5
f(x)=-5
=>5x=-5
=>x=-1
f(x)=2010
=>5x=2010
hay x=402