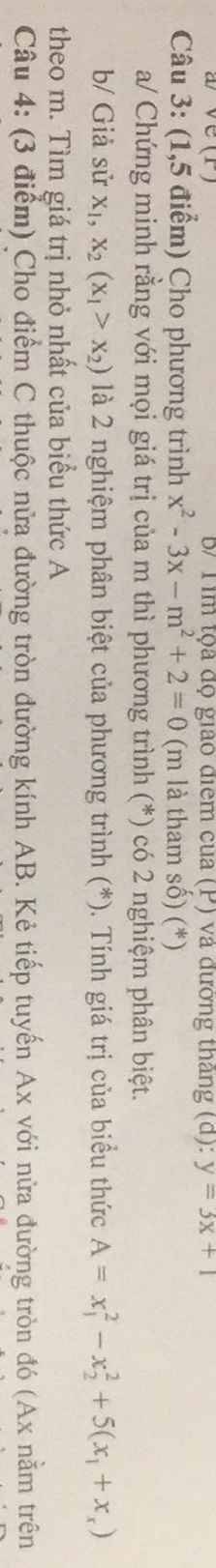
a: Xét tứ giác MHAO có \(\widehat{MHO}=\widehat{MAO}=90^0\)

nên MHAO là tứ giác nội tiếp

=>M,H,A,O cùng thuộc một đường tròn

b: Xét (O) có

MA,MB là các tiếp tuyến

Do đó: MA=MB

=>M nằm trên đường trung trực của AB(1)

ta có: OA=OB

=>O nằm trên đường trung trực của AB(2)

Từ (1),(2) suy ra OM là đường trung trực của AB

=>OM\(\perp\)AB tại I

Xét ΔOIK vuông tại I và ΔOHM vuông tại H có

\(\widehat{IOK}\) chung

Do đó: ΔOIK~ΔOHM

=>\(\dfrac{OI}{OH}=\dfrac{OK}{OM}\)

=>\(OI\cdot OM=OH\cdot OK\)

a: ΔABC vuông tại A

=>\(AB^2+AC^2=BC^2\)

=>\(AC=\sqrt{9^2-5^2}=2\sqrt{14}\left(cm\right)\)

Xét ΔABC vuông tại A có \(sinC=\dfrac{AB}{BC}=\dfrac{5}{9}\)

nên \(\widehat{C}\simeq33^045'\)

=>\(\widehat{B}=90^0-\widehat{C}\simeq56^015'\)

b: ΔABC vuông tại A

=>\(\widehat{ABC}+\widehat{ACB}=90^0\)

=>\(\widehat{ACB}=60^0\)

Xét ΔABC vuông tại A có \(sinB=\dfrac{AC}{BC}\)

=>\(\dfrac{AC}{8}=sin30=\dfrac{1}{2}\)

=>AC=4(cm)

ΔABC vuông tại A

=>\(AB^2+AC^2=BC^2\)

=>\(AB^2=8^2-4^2=48\)

=>\(AB=4\sqrt{3}\left(cm\right)\)

Giúp mk với ạ tối trước 10h mk phải nộp r

b) \(B=\dfrac{1}{\sqrt{x}+1}+\dfrac{\sqrt{x}}{\sqrt{x}-1}+\dfrac{2}{x-1}\left(x\ge0,x\ne1\right)\\ =\dfrac{1}{\sqrt{x}+1}+\dfrac{\sqrt{x}}{\sqrt{x}-1}+\dfrac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ =\dfrac{\sqrt{x}-1+\sqrt{x}\left(\sqrt{x}+1\right)+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ =\dfrac{\sqrt{x}-1+x+\sqrt{x}+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ =\dfrac{x+2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ \)

\(=\dfrac{\left(\sqrt{x}+1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\)

c)

 \(AB\le8\Leftrightarrow\dfrac{4\sqrt{x}}{x-1}.\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\le8\\ \Leftrightarrow\dfrac{4\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}.\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\le8\\ \Leftrightarrow\dfrac{4\sqrt{x}}{\left(\sqrt{x}-1\right)^2}\le8\\ \Leftrightarrow4\sqrt{x}\le8\left(x-2\sqrt{x}+1\right)\\ \) ( Nhân cả 2 vế BPT cho \(\left(\sqrt{x}-1\right)^2>0\) )

\(\Leftrightarrow8x-16\sqrt{x}+8\ge4\sqrt{x}\\ \Leftrightarrow8x-20\sqrt{x}+8\ge0\\ \Leftrightarrow2x-5\sqrt{x}+2\ge0\\ \)

\(\Leftrightarrow\left(2x-4\sqrt{x}\right)-\left(\sqrt{x}-2\right)\ge0\\ \Leftrightarrow2\sqrt{x}\left(\sqrt{x}-2\right)-\left(\sqrt{x}-2\right)\ge0\\ \Leftrightarrow\left(\sqrt{x}-2\right)\left(2\sqrt{x}-1\right)\ge0\\ \)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}\sqrt{x}-2\ge0\\2\sqrt{x}-1\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}\sqrt{x}-2\le0\\2\sqrt{x}-1\le0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}\sqrt{x}\ge2\\\sqrt{x}\ge\dfrac{1}{2}\end{matrix}\right.\\\left\{{}\begin{matrix}\sqrt{x}\le2\\\sqrt{x}\le\dfrac{1}{2}\end{matrix}\right.\end{matrix}\right.\\ \)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge4\\x\ge\dfrac{1}{4}\end{matrix}\right.\\\left\{{}\begin{matrix}x\le4\\x\le\dfrac{1}{4}\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\ge4\\x\le\dfrac{1}{4}\end{matrix}\right.\)

Kết hợp ĐK: \(x\ge0,x\ne1\)

Kết luận: \(x\ge4\) hoặc \(0\le x\le\dfrac{1}{4}\) thì \(AB\le8\)

Lời giải:

ĐK: $x\geq 0; x\neq 1$

$AB=\frac{4\sqrt{x}}{(\sqrt{x}-1)^2}\leq 8$

$\Rightarrow 4\sqrt{x}\leq 8(\sqrt{x}-1)^2$
$\Leftrightarrow \sqrt{x}\leq 2(\sqrt{x}-1)^2$
$\Leftrightarrow \sqrt{x}\leq 2(x-2\sqrt{x}+1)$

$\Leftrightarrow 2x-5\sqrt{x}+2\geq 0$

$\Leftrightarrow (\sqrt{x}-2)(2\sqrt{x}-1)\geq 0$

$\Leftrightarrow \sqrt{x}\geq 2$ hoặc $\sqrt{x}\leq \frac{1}{2}$

$\Leftrightarrow x\geq 4$ hoặc $0\leq x\leq \frac{1}{4}$

Kết hợp đkxđ suy ra $x\geq 4$ hoặc $0\leq x\leq \frac{1}{4}$

\(B=\dfrac{2\sqrt{x}-3}{\sqrt{x}-1}+\dfrac{3-\sqrt{x}}{x-1}\)

\(=\dfrac{2\sqrt{x}-3}{\sqrt{x}-1}+\dfrac{3-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\dfrac{\left(2\sqrt{x}-3\right)\left(\sqrt{x}+1\right)+3-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\dfrac{2x+2\sqrt{x}-3\sqrt{x}-3+3-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\dfrac{2x-2\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{2\sqrt{x}}{\sqrt{x}+1}\)

\(B=\dfrac{2x-3}{\sqrt{x}-1}+\dfrac{3-\sqrt{x}}{x-1}\)

\(=\dfrac{2x-3}{\sqrt{x}-1}+\dfrac{3-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\dfrac{\left(2x-3\right)\left(\sqrt{x}+1\right)+3-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\dfrac{2x\sqrt{x}+2x-3\sqrt{x}-3+3-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\dfrac{2x\sqrt{x}+2x-4\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{2\sqrt{x}\left(x+\sqrt{x}-2\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\dfrac{2\sqrt{x}\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{2\sqrt{x}\left(\sqrt{x}+2\right)}{\sqrt{x}+1}=\dfrac{2x+4\sqrt{x}}{\sqrt{x}+1}\)

Mình sửa đề nhé;-; Đề trước lỗi á

a: \(\text{Δ}=\left(-3\right)^2-4\left(-m^2+2\right)\)

\(=9+4m^2-8=4m^2+1>0\forall m\)

=>Phương trình luôn có hai nghiệm phân biệt

b:

Theo Vi-et, ta có:

\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=3\\x_1x_2=\dfrac{c}{a}=-m^2+2\end{matrix}\right.\)

 \(x_1>x_2\)

=>\(x_1-x_2>0\)

\(\left(x_1-x_2\right)^2=\left(x_1+x_2\right)^2-4x_1x_2\)

\(=3^2-4\left(-m^2+2\right)\)

\(=9+4m^2-8=4m^2+1\)

=>\(x_1-x_2=\sqrt{4m^2+1}\)

\(A=x_1^2-x_2^2+5\left(x_1+x_2\right)\)

\(=\left(x_1-x_2\right)\left(x_1+x_2\right)+5\left(x_1+x_2\right)\)

\(=3\sqrt{4m^2+1}+15>=3\cdot1+15=18\forall m\)

Dấu '=' xảy ra khi m=0