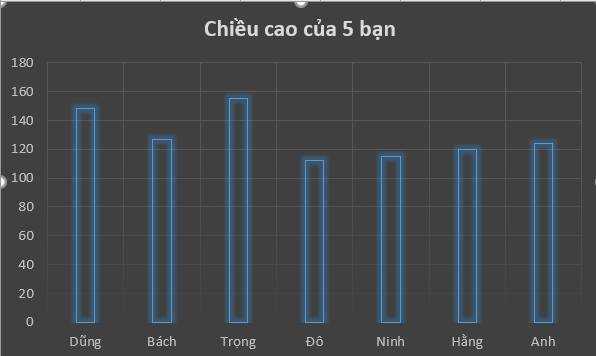
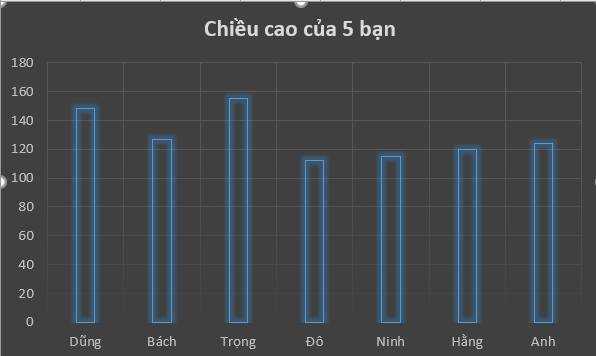
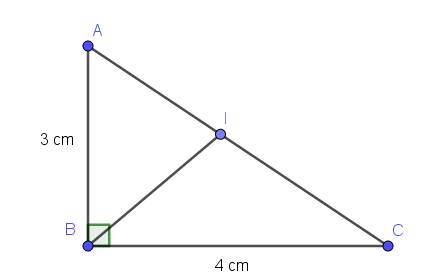
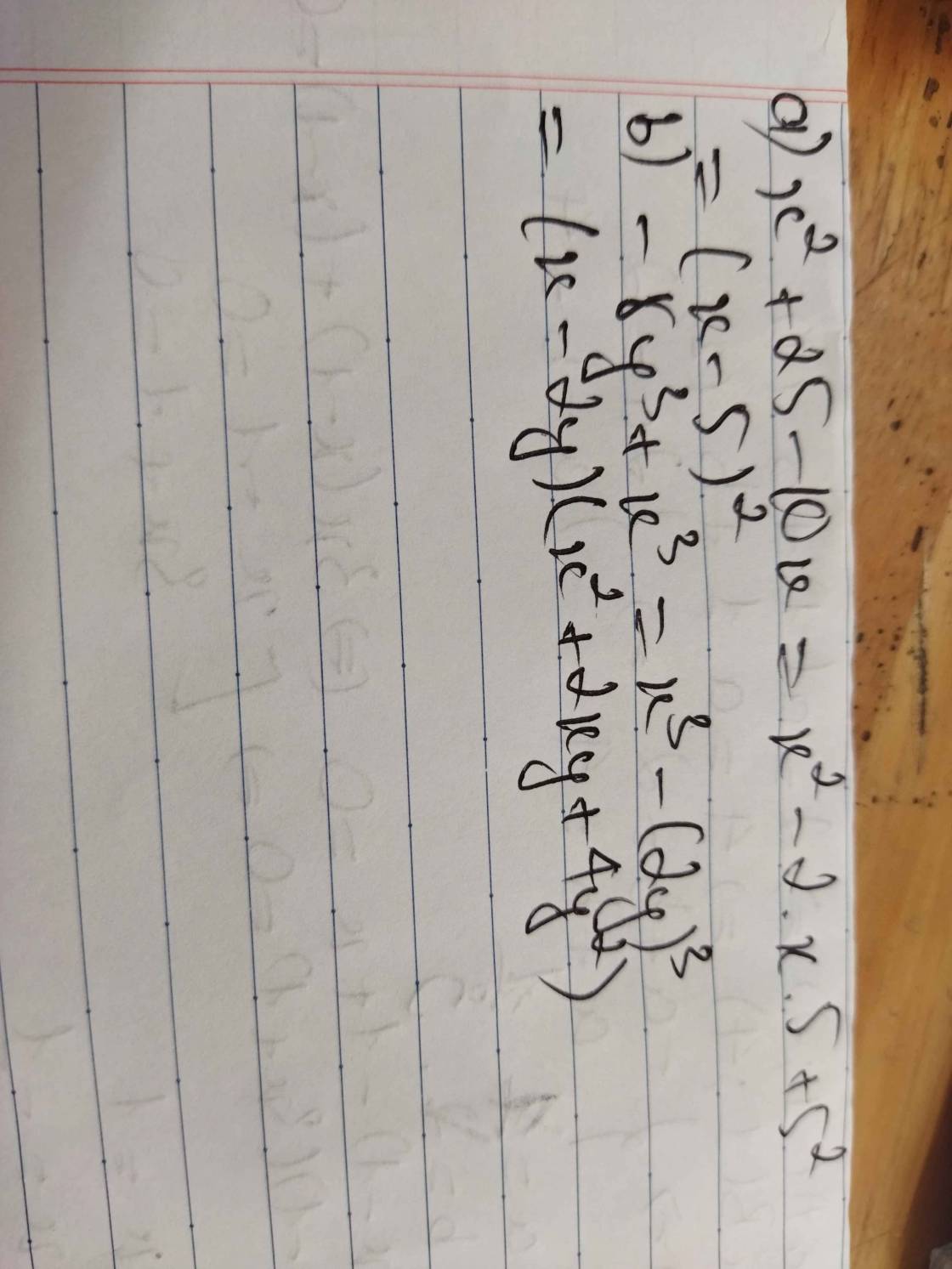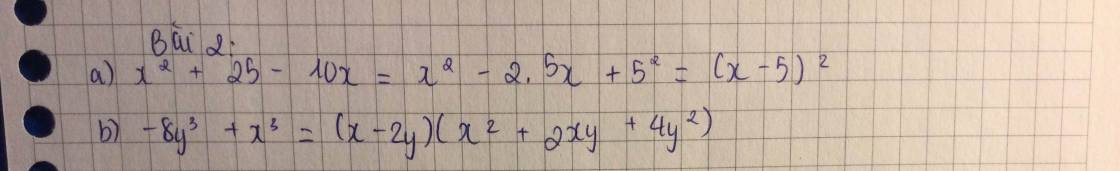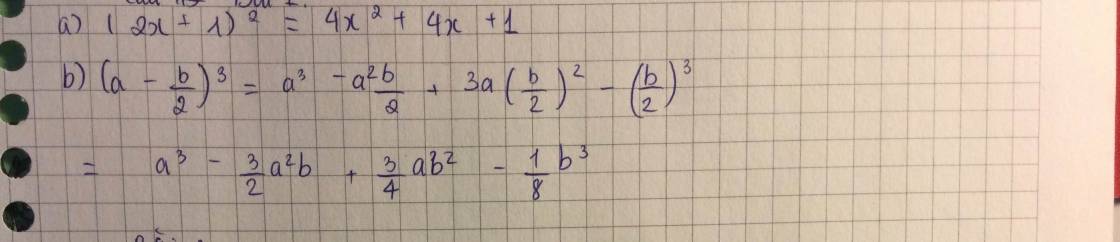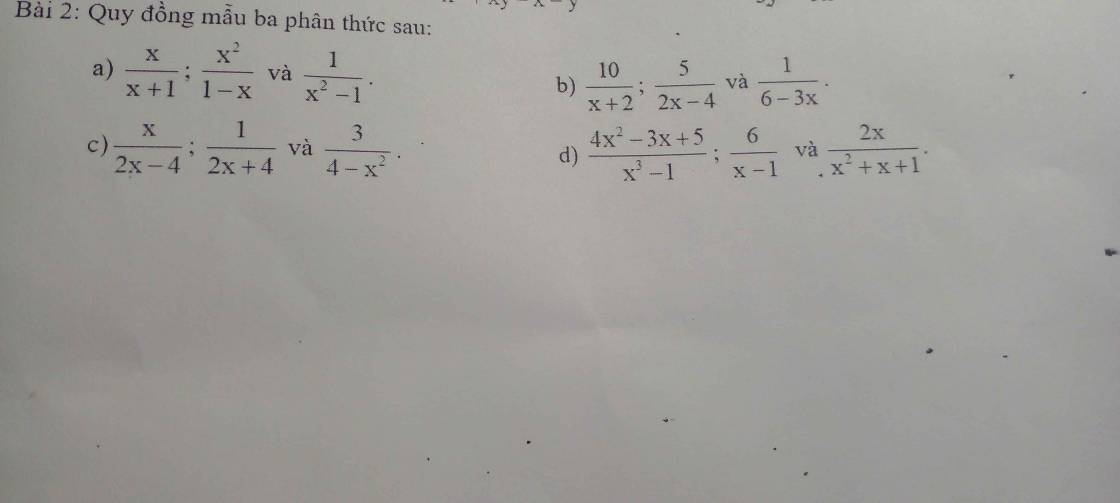Câu 17. (1 điểm) Cho bảng số liệu sau:
|
Học sinh |
Dũng |
Bách |
Trọng |
Đô |
Ninh |
Hằng |
Anh |
|
Chiều cao (cm) |
148 |
127 |
155 |
112 |
115 |
120 |
124 |
Lựa chọn biểu đồ phù hợp biểu diễn bảng thông kê này. Vẽ biểu đồ đó.