Cho tam giác ABC vuông tại A, đường cao AH, góc ACB = 30 độ, phân giác góc ABC cắt AH tại E. Tính các tỉ số BH/AH và sBHE/sCAB (s là diện tích tam giác)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Vẫn đề đó hả em
Câu này dùng BĐT Schur là ra luôn cx đc, nhưng mà thế thì hơi mất hứng, anh thử đề xuất phương án này ha
VT=\(cyc\sum x^5.\left(x-y+z\right)\) Gấp đôi vế trái lên và phá ngoặc ra nhóm về kiểu này
2.VT=(x^6-2x^5y+2xy^5+y^6)+.......tương tự như thế ha
Giờ chỉ cần mỗi cái ngoặc này >=0 là cả lũ >=0 do tương tự
Mà \(x^6-2x^5y+2xy^5+y^6=\left(x^2+y^2\right).\left(x^2-xy-y^2\right)^2\) (Cái này em nhóm 2 cái cuối, 2 cái giữa xong triển khai ra là đc)
Dễ thấy x^2+y^2>=0, cái ngoặc kia là bình phương cũng >=0
Do đó cái TH kia >=0. Các th còn lại thì cx tương tự
Cộng vế với vế suy ra 2VT>=0, Hay VT>=0 (đpcm)
Anh gửi riêng phần phân tích này
\(x^6-2x^5y+2xy^5+y^6=\left(x^2+y^2\right)\left(x^4-x^2y^2+y^4\right)-2xy\left(x^2+y^2\right)\left(x^2-y^2\right)=\left(x^2+y^2\right).\left(x^4-x^2y^2+y^4-2xy\left(x^2-y^2\right)\right)=\left(x^2+y^2\right)\left(\left(x^4-2x^2y^2+y^4\right)-2xy\left(x^2-y^2\right)+x^2y^2\right)\)Viết tiếp cái ngoặc to thành bình phương là ra cái anh vt chỗ trên đầu nhé
Thử xem có đc ko

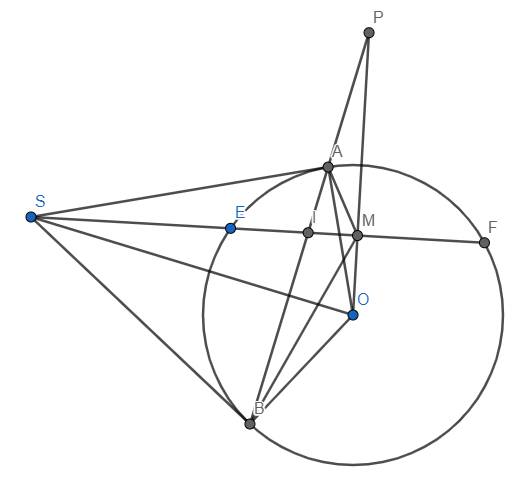
a) Do SA là tiếp tuyến tại A của (O) nên \(\widehat{OAS}=90^o\). Tương tự, ta có \(\widehat{OBS}=90^o\), suy ra \(\widehat{OAS}+\widehat{OBS}=180^o\). Do đó tứ giác SAOB nội tiếp. (đpcm)
Mặt khác, trong đường tròn (O) có M là trung điểm của dây EF nên \(OM\perp EF\) tại M hay \(\widehat{OMS}=90^o\). Từ đó suy ra \(\widehat{OMS}=\widehat{OAS}\),từ đó tứ giác OMAS nội tiếp. Vì vậy 5 điểm O, M, A, S, B cùng thuộc một đường tròn \(\Rightarrow\) Tứ giác SAMO nội tiếp (đpcm)
b) Ta thấy tứ giác OMAB nội tiếp nên \(\widehat{PMA}=\widehat{PBO}\). Từ đó dễ dàng suy ra \(\Delta PAM~\Delta POB\left(g.g\right)\Rightarrow\dfrac{PA}{PO}=\dfrac{PM}{PB}\) \(\Rightarrow PA.PB=PO.PM\) (đpcm)
c) Do tứ giác SAMB nội tiếp nên \(\widehat{SMB}=\widehat{SAB}\) và \(\widehat{SMA}=\widehat{SBA}\). Mặt khác, trong đường tròn (O), có 2 tiếp tuyến tại A và B cắt nhau tại S nên \(SA=SB\) hay \(\Delta SAB\) cân tại S \(\Rightarrow\widehat{SAB}=\widehat{SBA}\) \(\Rightarrow\widehat{SMB}=\widehat{SMA}\) hay MI là phân giác trong của \(\widehat{AMB}\) . Lại có \(MP\perp MI\) nên MP là phân giác ngoài của \(\widehat{AMB}\). Áp dụng tính chất đường phân giác, ta thu được \(\dfrac{IA}{IB}=\dfrac{MA}{MB}\) và \(\dfrac{PA}{PB}=\dfrac{MA}{MB}\). Từ đây suy ra \(\dfrac{IA}{IB}=\dfrac{PA}{PB}\) \(\Rightarrow PA.IB=PB.IA\) (đpcm)

Đề thi chuyên SP hả em, bài này sử dụng Liên hợp với đánh giá em nhé:
Đầu tiên trừ 2 về mình có là
\(x\sqrt{y+4}+x\sqrt{y+11}-y\sqrt{x+4}-y\sqrt{x+11}=0\)
Từ hệ mình dễ dàng suy ra đc x,y>0
Anh liên hợp cho 1 cái nha
\(x\sqrt{y+4}-y\sqrt{x+4}=\sqrt{x^2y+4x^2}-\sqrt{y^2x+4y^2}=\dfrac{x^2y-y^2x+4x^2-4y^2}{\sqrt{.........}+\sqrt{.......}}=\left(x-y\right).\dfrac{xy+4x+4y}{\sqrt{.........}+\sqrt{............}}\)
Cái kia em cx liên hợp tương tự, đặt x-y của cả 2 cái khi liên hợp xong phương trình sẽ là
\(\left(x-y\right)\left(\dfrac{xy+4x+4y}{\sqrt{...}+\sqrt{...}}+\dfrac{xy+11x+11y}{\sqrt{........}+\sqrt{.....}}\right)=0\) Cái trong ngoặc to đùng hiển nhiên >0 với x,y>0. DO đó x-y=0 hay x=y
EM thế vào phương trình ban đầu thì có \(x\sqrt{x+4}+x\sqrt{x+11}=35\)
Đến đây thì nhẩm đc x=5 thoả mãn em giải bằng đánh giá:
Với x=5 suy ra......=35
Với x>5 suy ra......>35
Với x<5 suy ra.....<35
Kết luận đc x=5, do đó y=5
Note: hướng làm em nhé, bổ sung thêm điều kiện xác định linh tinh zô

Lời giải:
Gọi chiều rộng mảnh đất là $a$ (m) thì chiều dài mảnh đất là $a+40$ (m)
Chiều dài bể: $(a+40-10-10)=a+20$ (m)
Chiều rộng bể: $a-10-10=a-20$ (m)
Diện tích bể: $(a+20)(a-20)=6000$
$\Leftrightarrow a^2-400=6000$
$\Leftrightarrow a^2=6400$
$\Rightarrow a=\sqrt{6400}=80$ (m)
Vậy chiều rộng mảnh vườn là $80$ m, chiều dài mảnh vườn là $80+40=120$ m

Gọi số dãy ghế ban đầu là x và số ghế trong mỗi dãy ban đầu là y (với \(x;y\in N\) và \(x;y>0\))
Do hội trường ban đầu có 510 chỗ ngồi nên ta có: \(xy=510\)
Số dãy ghế lúc sau: \(x+3\)
Số ghế mỗi dãy lúc sau: \(y+2\)
Do sau khi tăng thì đủ ghế cho 640 người nên: \(\left(x+3\right)\left(y+2\right)=640\)
Ta được hệ:
\(\left\{{}\begin{matrix}xy=510\\\left(x+3\right)\left(y+2\right)=640\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}xy=510\\xy+2x+3y+6=640\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}xy=510\\2x+3y=124\end{matrix}\right.\)
\(\Rightarrow x\left(124-2x\right)=510.3\)
\(\Rightarrow2x^2-124x+1530=0\Rightarrow\left[{}\begin{matrix}x=45\Rightarrow y=\dfrac{34}{3}\left(loại\right)\\x=17\Rightarrow y=30\end{matrix}\right.\)
Dễ thấy \(\widehat{BAH}=90^o-\widehat{B}=\widehat{C}\), mà \(\widehat{C}=30^o\) nên \(\widehat{BAH}=30^o\). Trong tam giác ABH vuông tại H, ta có \(\dfrac{BH}{AH}=\tan\widehat{BAH}=\tan30^o=\dfrac{\sqrt{3}}{3}\).
Trước hết ta tính \(\dfrac{S_{BHE}}{S_{ABH}}\). Để ý rằng \(\dfrac{S_{BHE}}{S_{ABH}}=\dfrac{EH}{AH}\). Mặt khác, \(\dfrac{EH}{AE}=\dfrac{BH}{AB}=\sin\widehat{BAH}=\sin30^o=\dfrac{1}{2}\) \(\Rightarrow\dfrac{EH}{AH}=\dfrac{1}{3}\) hay \(\dfrac{S_{BHE}}{S_{ABH}}=\dfrac{1}{3}\) (*). Lại thấy \(\dfrac{S_{ABH}}{S_{ABC}}=\dfrac{BH}{BC}\), mà \(\dfrac{BH}{AB}=\dfrac{1}{2}\Rightarrow BH=\dfrac{1}{2}AB\) và \(\dfrac{AB}{BC}=\sin\widehat{C}=\sin30^o=\dfrac{1}{2}\) \(\Rightarrow AB=\dfrac{1}{2}BC\). Từ đó suy ra \(BH=\dfrac{1}{4}BC\) hay \(\dfrac{BH}{BC}=\dfrac{1}{4}\) hay \(\dfrac{S_{ABH}}{S_{ABC}}=\dfrac{1}{4}\) (**)
Từ (*) và (**) \(\Rightarrow\dfrac{S_{BHE}}{S_{ABH}}.\dfrac{S_{ABH}}{S_{ABC}}=\dfrac{1}{3}.\dfrac{1}{4}\Rightarrow\dfrac{S_{BHE}}{S_{ABC}}=\dfrac{1}{12}\)