(3,0 điểm) Giải các phương trình sau:
a) $2x+4=x-1$.
b) $2x(x-3)-5(x-3)=0$.
c) $\displaystyle \frac{2x}{x+1} = \frac{x^2-x+8}{(x+1)(x-4)}$.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

`x^2-4x+y^2-6y+15=2`
`<=>x^2-4x+4+y^2-6y+9=0`
`<=>(x-2)^2+(y-3)^2=0`
`=>x-2=0` và `y-3=0`
`<=>x=2` và `y=3`
Ta có:
�2−4�+�2−6�+15=2⇔�2−4�+4+�2−6�+9=0⇔(�−2)2+(�−3)2=0⇔{(�−2)2=0(�−3)2=0⇔{�=2�=3⇔⇔⇔x2−4x+y2−6y+15=2x2−4x+4+y2−6y+9=0(x−2)2+(y−3)2=0{(x−2)2=0(y−3)2=0⇔{x=2y=3
(vì (�−2)2≥0(x−2)2≥0 với mọi �∈�x∈R, (�−3)2≥0(y−3)2≥0 với mọi �∈�y∈R).
Vậy �=2x=2, �=3y=3.

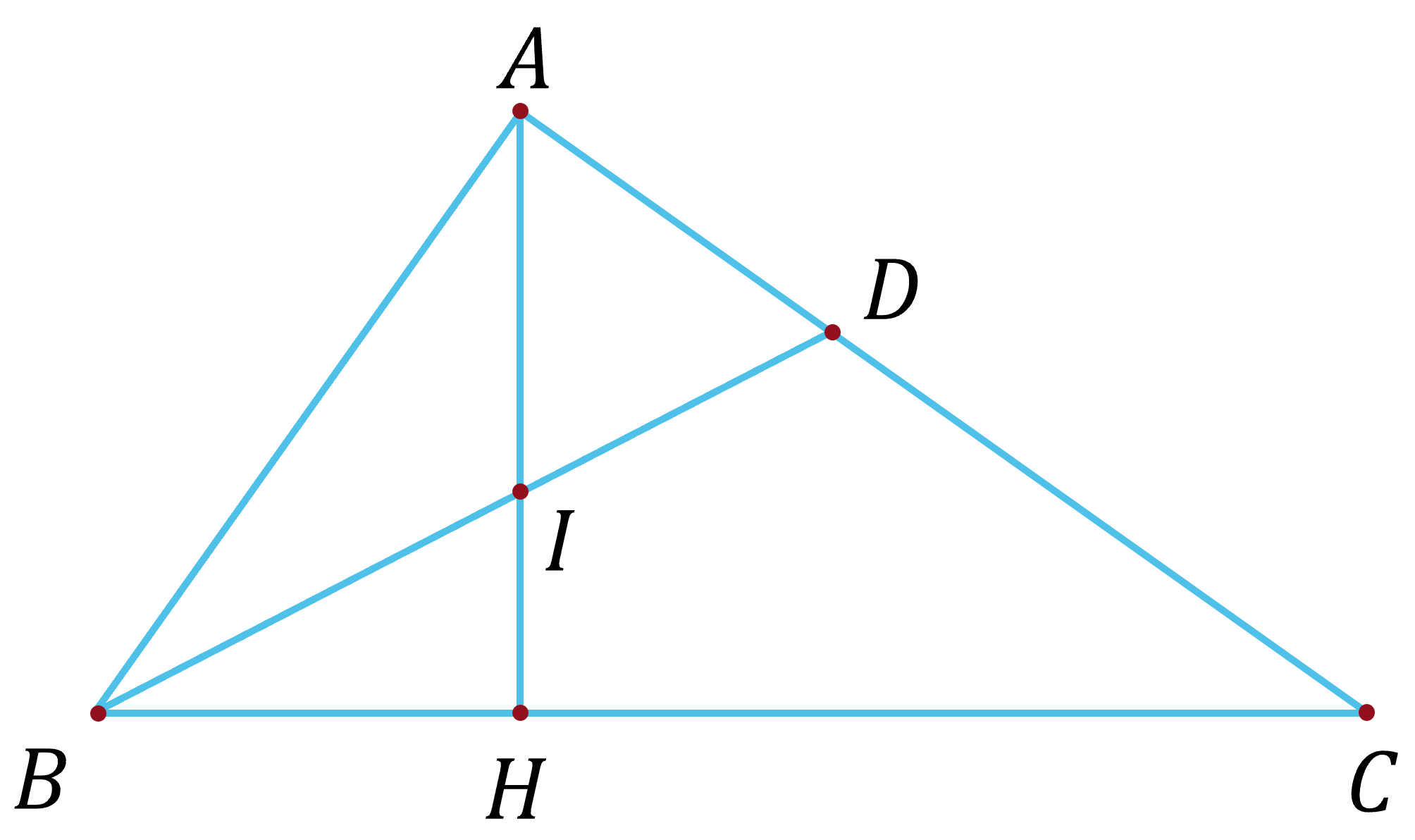
A A B C H D I
a) Vì tam giác ABC vuông tại A
Áp dụng định lý Pytago :
AB2 + AC2 = BC2
<=> 62 + 82 = BC2
<=> BC = 10
BD tia phân giác góc B nên \(\dfrac{AD}{DC}=\dfrac{AB}{BC}=\dfrac{3}{5}\)(1)
mà AD + DC = AC = 8 (2)
Từ (1)(2) ta tìm được AD = 3 ; DC = 5
=> P = AD.DC = 3.5 = 15
b) Mà \(BD\cap AH=\left\{I\right\}\)
\(\Rightarrow\dfrac{AI}{IH}=\dfrac{AB}{BH}\)(3)
Xét tam giác ABH và tam giác ABC có
\(\widehat{ABC}\) chung ; \(\widehat{AHB}=\widehat{BAC}=90^{\text{o}}\)
nên \(\Delta CBA\sim\Delta ABH\)
\(\Rightarrow\dfrac{BH}{AB}=\dfrac{AB}{BC}\)
\(\Rightarrow\dfrac{IH}{IA}=\dfrac{AD}{DC}\)( kết hợp (1);(3))
c) Tương tự dễ thấy
\(\Delta BIH\sim\Delta BDA\) (g-g)
=> \(\widehat{BDA}=\widehat{BIH}\)
lại có \(\widehat{BIH}=\widehat{AID}\) (đối đỉnh)
nên \(\widehat{BDA}=\widehat{AID}\) => Tam giác AID cân tại A
a) Xét tam giác ���ABC vuông tại �A:
��2=��2+��2BC2=AB2+AC2 (định lí Pythagoras)
⇔��2=62+82=100⇔��=10(��)⇔BC2=62+82=100⇔BC=10(cm).
Xét tam giác ���ABC phân giác ��BD có:
����=����⇔����+��=����+��BCAB=DCAD⇔BC+ABAB=DC+ADAD
����=����+��⇔��=3(��)ACAD=BC+ABAB⇔AD=3(cm)
suy ra ��=5(��)DC=5(cm).
b) Xét tam giác ���ABH phân giác ��BI có: ����=����IAIH=ABHB.
Xét △���△HBA và △���△ABC có:
���^=���^HBA=ABC (góc chung)
���^=���^(=90∘)BHA=BAC(=90∘)
suy ra △���∼△���△HBA∼△ABC (g.g).
Suy ra ����=����ABHB=BCBA
⇒����=����⇒BCBA=IAIH.
Mà ta lại có ����=����BCAB=DCAD nên ����=����IAIH=DCAD.
c) Ta có △���∼△���△ABD∼△HBI (g.g)
suy ra ����=����⇒��.��=��.��HBAB=BIBD⇒AB.BI=BD.HB.
���^=���^BDA=BIH (hai góc tương ứng)
mà ���^=���^BIH=AID (hai góc đối đỉnh)
suy ra ���^=���^BDA=AID
do đó tam giác ���AID cân tại �A.

Cái này anh thấy có vẻ chưa đúng đề lắm em ạ
Vì 440 không chia hết cho 3 nên sao bằng được

Gọi số đó là \(\overline{ab}\)
Vì đó là số lẻ chia hết cho `5` nên `b=5`
\(=>\overline{a5}\)
Vì hiện của số đó và chữ số hàng chục của nó bằng `86` nên ta có:
\(overline{a5}-a=86\)
\(<=>10a+5-a=86\)
`<=>a=9`
Vậy số cần tìm là `95`

a)
\(\dfrac{x-3}{5}+\dfrac{1-2x}{3}=6\\ < =>3x-9+5-10x=90\)
\(< =>3x-10x=90+9-5\\ < =>-7x=94\\ < =>x=-\dfrac{94}{7}\)
b)
\(\left(2x-3\right)\left(x^2+1\right)=0\\ < =>\left[{}\begin{matrix}2x-3=0\\x^2+1=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=\dfrac{3}{2}\\x^2=-1\left(voli\right)\end{matrix}\right.\\ < =>x=\dfrac{3}{2}\)
c)
\(\dfrac{2}{x+1}-\dfrac{1}{x-2}=\dfrac{3x-11}{\left(x+1\right)\left(x-2\right)}\left(x\ne-1;x\ne2\right)\)
suy ra: \(2\left(x-2\right)-x-1=3x-11\)
\(< =>2x-4-x-1-3x+11=0\)
\(< =>2x-x-3x=4+1-11\\ < =>-2x=-6\\ < =>x=3\left(tm\right)\)
a) \(\dfrac{x-3}{5}+\dfrac{1-2x}{3}=6\)
\(\Leftrightarrow3\left(x-3\right)+5\left(1-2x\right)=90\)
\(\Leftrightarrow-4-7x=90\)
\(\Leftrightarrow x=-\dfrac{94}{7}\)
b) \(\left(2x-3\right)\left(x^2+1\right)=0\)
\(\Leftrightarrow2x-3=0\) (Vì \(x^2+1>0\))
\(\Leftrightarrow x=\dfrac{3}{2}\)
c) \(\dfrac{2}{x+1}-\dfrac{1}{x-2}=\dfrac{3x-11}{\left(x+1\right)\left(x-2\right)}\left(Đk:x\ne-1;x\ne2\right)\)
\(\Leftrightarrow2\left(x-2\right)-\left(x+1\right)=3x-11\)
\(\Leftrightarrow x-5=3x-11\)
\(\Leftrightarrow x=3\)

a) ĐKXĐ : \(\left\{{}\begin{matrix}3x-2\ne0\\3x+2\ne0\\4-9x^2\ne0\end{matrix}\right.\Leftrightarrow x\ne\pm\dfrac{2}{3}\)
\(C=\dfrac{1}{3x-2}-\dfrac{4}{3x+2}-\dfrac{3x-6}{4-9x^2}\)
\(=\dfrac{3x+2}{\left(3x-2\right)\left(3x+2\right)}-\dfrac{4.\left(3x-2\right)}{\left(3x-2\right)\left(3x+2\right)}+\dfrac{3x-6}{9x^2-4}\)
\(=\dfrac{3x+2-4.\left(3x-2\right)+3x-6}{\left(3x-2\right).\left(3x+2\right)}=\dfrac{-6x+4}{\left(3x-2\right).\left(3x+2\right)}\)
\(=\dfrac{-2}{3x+2}\)
b) Với \(x\inℤ\)
Ta có : \(C\inℤ\Leftrightarrow-2⋮3x+2\)
\(\Leftrightarrow3x+2\inƯ\left(-2\right)\)
\(\Leftrightarrow3x+2\in\left\{1;2;-1;-2\right\}\)
Lập bảng
| 3x + 2 | 1 | 2 | -2 | -1 |
| x | \(-\dfrac{1}{3}\left(\text{loại}\right)\) | 0(tm) | \(-\dfrac{4}{3}\left(\text{loại}\right)\) | -1(tm) |
Vậy \(x\in\left\{0;-1\right\}\)
Ta có :
Lập bảng
| 3x + 2 | 1 | 2 | -2 | -1 |
| x | 0(tm) | -1(tm) |
Vậy

a)
`2x+4=x-1`
`<=>2x-x=-4-1`
`<=>x=-5`
Vậy phương trình có tập nghiệm là : \(S=\left\{-5\right\}\)
b)
`2x(x-3)-5(x-3)=0`
`<=>(x-3)(2x-5)=0`
`=>x-3=0` hoặc `2x-5=0`
`<=>x=3` hoặc `x=5/2`
Vậy tập nghiệm phương trình là : \(S=\left\{3;\dfrac{5}{2}\right\}\)
c)
\(\dfrac{2x}{x+1}=\dfrac{x^2-x+8}{\left(x+1\right)\left(x-4\right)}\left(x\ne\left\{-1;4\right\}\right)\\ < =>\dfrac{2x\left(x-4\right)}{\left(x+1\right)\left(x-4\right)}=\dfrac{x^2-x+8}{\left(x+1\right)\left(x-4\right)}\\ =>2x\left(x-4\right)=x^2-x+8\\ < =>2x^2-8x=x^2-x+8\\ < =>2x^2-x^2-8x+x-8=0\\ < =>x^2-7x-8=0\\ < =>\left(x-8\right)\left(x+1\right)=0\\ =>\left[{}\begin{matrix}x=8\left(N\right)\\x=-1\left(L\right)\end{matrix}\right.\)
Vậy tập nghiệm phương trình là : \(S=\left\{8\right\}\)