Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


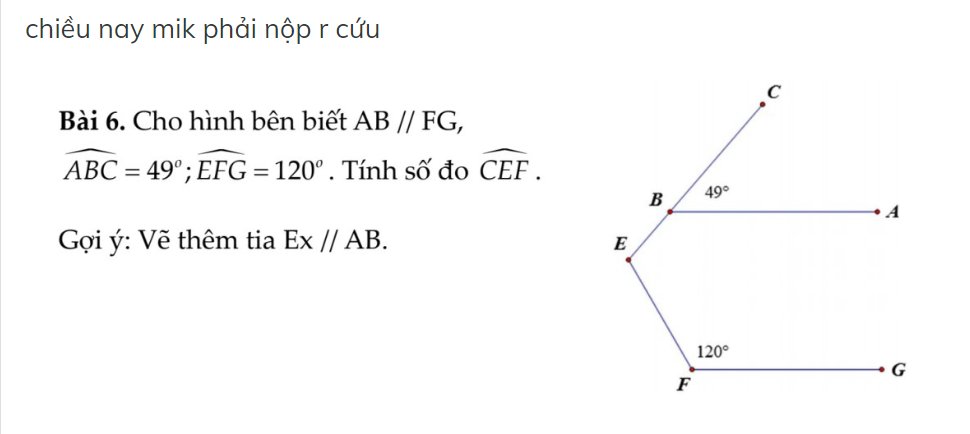
Kẻ Ex//AB(Ex và AB nằm trên cùng mặt phẳng bờ chứa tia BE)
Ta có: Ex//AB
AB//FG
Do đó: Ex//FG
Ex//AB
=>\(\widehat{BEx}=\widehat{CBA}\)(hai góc đồng vị)
=>\(\widehat{xEB}=49^0\)
Ta có: Ex//FG
=>\(\widehat{xEF}+\widehat{EFG}=180^0\)
=>\(\widehat{xEF}=180^0-120^0=60^0\)
\(\widehat{BEF}=\widehat{xEB}+\widehat{xEF}=49^0+60^0=109^0\)

a: Ta có: \(\widehat{xBy}=\widehat{xAz}\left(=60^0\right)\)
mà hai góc này là hai góc ở vị trí đồng vị
nên By//Az
b: Ta có: \(\widehat{ABC}+\widehat{xBC}=180^0\)(hai góc kề bù)
=>\(\widehat{ABC}+60^0=180^0\)
=>\(\widehat{ABC}=120^0\)
AC là phân giác của góc zAB
=>\(\widehat{BAC}=\dfrac{\widehat{xAB}}{2}=30^0\)
Xét ΔBAC có \(\widehat{ABC}+\widehat{BAC}+\widehat{BCA}=180^0\)
=>\(\widehat{BCA}+120^0+30^0=180^0\)
=>\(\widehat{BCA}=30^0\)
c: Ta có: BD là phân giác của góc ABC
=>\(\widehat{ABD}=\dfrac{\widehat{ABC}}{2}=60^0\)
Xét ΔDBA có \(\widehat{DBA}+\widehat{DAB}=60^0+30^0=90^0\)
nên ΔBDA vuông tại D
=>BD\(\perp\)AC

AE//BD
=>\(\widehat{BAE}+\widehat{ABD}=180^0\)(hai góc trong cùng phía)
=>\(\widehat{BAE}+90^0=180^0\)
=>\(\widehat{BAE}=90^0\)
Ta có: AE//BD
=>\(\widehat{AED}+\widehat{BDE}=180^0\)(hai góc trong cùng phía)
=>\(\widehat{BDE}+55^0=180^0\)
=>\(\widehat{BDE}=125^0\)

a: a\(\perp\)HK
b\(\perp\)HK
Do đó: a//b
b: Ta có: \(\widehat{BAH}+45^0=180^0\)
=>\(\widehat{BAH}=180^0-45^0=135^0\)
Ta có: a//b
=>\(\widehat{BAH}+\widehat{ABK}=180^0\)(hai góc trong cùng phía)
=>\(\widehat{ABK}+135^0=180^0\)
=>\(\widehat{ABK}=45^0\)

a: Hiệu vận tốc hai xe là 51-36=15(km/h)
Thời gian ô tô đuổi kịp xe máy là:
45:15=3(giờ)
b: Hai xe gặp nhau lúc:
8h30p+3h=11h30p

\(\dfrac{3}{1\text{x}2}+\dfrac{3}{2\text{x}3}+...+\dfrac{3}{99\text{x}100}\)
\(=3\text{x}\left(\dfrac{1}{1\text{x}2}+\dfrac{1}{2\text{x}3}+...+\dfrac{1}{99\text{x}100}\right)\)
\(=3\text{x}\left(1-\dfrac{1}{2}+\dfrac{1}{2}-\dfrac{1}{3}+...+\dfrac{1}{99}-\dfrac{1}{100}\right)\)
\(=3\text{x}\left(1-\dfrac{1}{100}\right)=3\text{x}\dfrac{99}{100}=\dfrac{297}{100}\)
\(\dfrac{3}{1\times2}+\dfrac{3}{2\times3}+...+\dfrac{3}{99\times100}\)
\(=3\times\left(\dfrac{1}{1\times2}+\dfrac{1}{2\times3}+...+\dfrac{1}{99\times100}\right)\)
\(=3\times\left(1-\dfrac{1}{2}+\dfrac{1}{2}-\dfrac{1}{3}+...+\dfrac{1}{99}-\dfrac{1}{100}\right)\)
\(=3\times\left(1-\dfrac{1}{100}\right)\)
\(=3\times\dfrac{99}{100}\)
\(=\dfrac{297}{100}\)

Số phần quả bóng còn lại so với tổng số bóng ban đầu là:
\(1-\dfrac{1}{7}-\dfrac{1}{5}=1-\dfrac{12}{35}=\dfrac{23}{35}\)

\(d.x^{11}+x^7+1\\ =x^{11}-x^2+x^7-x+x^2+x+1\\ =x^2\left(x^9-1\right)+x\left(x^6-1\right)+\left(x^2+x+1\right)\\ =x^2\left(x^3-1\right)\left(x^6+x^3+1\right)+x\left(x^3-1\right)\left(x^3+1\right)+\left(x^2+x+1\right)\\ =x^2\left(x-1\right)\left(x^2+x+1\right)\left(x^6+x^3+1\right)+x\left(x-1\right)\left(x^2+x+1\right)\left(x^3+1\right)+\left(x^2+x+1\right)\\=\left(x^2+x+1\right)\left[x^2\left(x-1\right)\left(x^6+x^3+1\right)+x\left(x-1\right)\left(x^3+1\right)+1\right]\\ =\left(x^2+x+1\right)\left[\left(x^3-x^2\right)\left(x^6+x^3+1\right)+\left(x^2-x\right)\left(x^3+1\right)+1\right]\\ =\left(x^2+x+1\right)\left(x^9+x^6+x^3-x^8-x^5-x^2+x^5+x^2-x^4-x+1\right)\\ =\left(x^2+x+1\right)\left(x^9-x^8+x^6-x^4+x^3-x+1\right)\)
\(e.x^8+x+1\\ =x^8-x^2+x^2+x+1\\ =x^2\left(x^6-1\right)+\left(x^2+x+1\right)\\ =x^2\left(x^3-1\right)\left(x^3+1\right)+\left(x^2+x+1\right)\\ =x^2\left(x-1\right)\left(x^2+x+1\right)\left(x^3+1\right)+\left(x^2+x+1\right)\\ =\left(x^2+x+1\right)\left[x^2\left(x-1\right)\left(x^3+1\right)+1\right]\\ =\left(x^2+x+1\right)\left[\left(x^3-x^2\right)\left(x^3+1\right)+1\right]\\ =\left(x^2+x+1\right)\left(x^6+x^3-x^5-x^2+1\right)\)

\(\left(x-1\right)+\left(x-2\right)+...+\left(x-20\right)=150\\ x-1+x-2+...+x-20=150\\ \left(x+x+...+x\right)-\left(1+2+...+20\right)\\ 20\cdot x-\left[\left(20-1\right):1+1\right]\cdot\left(20+1\right):2=150\\ 20\cdot x-20\cdot21:2=150\\ 20\cdot x-210=150\\ 20\cdot x=150+210\\ 20\cdot x=360\\ x=360:20\\ x=18\)





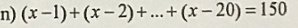
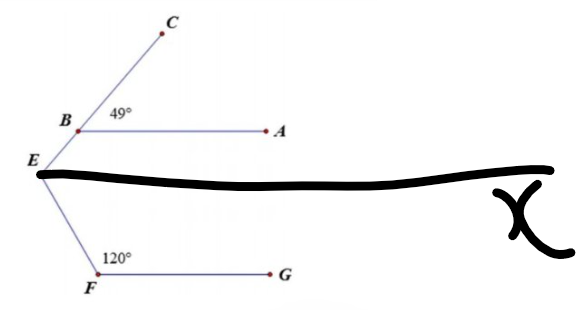
Kẻ Ex // AB
\(\widehat{BEx}\) = \(\widehat{CBA}\) = 490 (đồng vị)
\(\widehat{xEF}\) + \(\widehat{EFG}\) = 1800 (hai góc trong cùng phía)
⇒ \(\widehat{xEF}\) = 1800 - \(\widehat{EFG}\) = 1800 - 1200 = 600
\(\widehat{BEF}\) = \(\widehat{BEx}\) + \(\widehat{xEF}\) = 490 + 600 = 1090
Kết luận: góc BEF là 1090
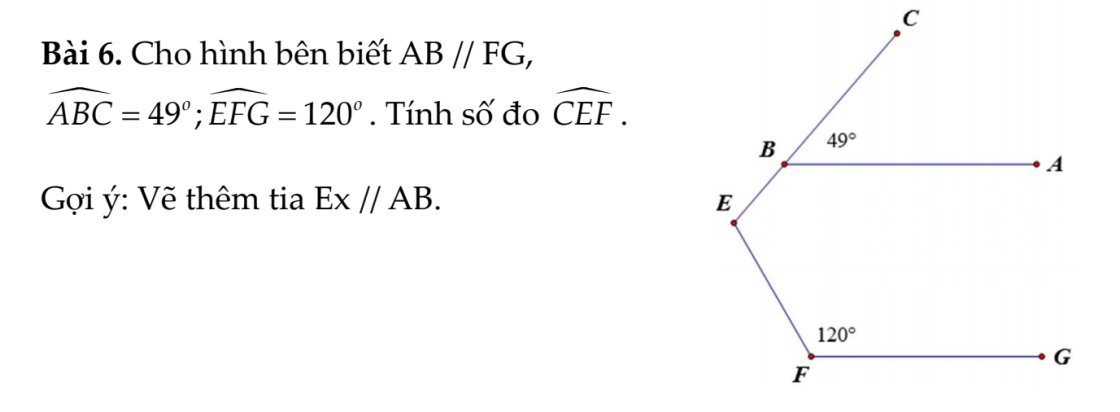
Kẻ Ex//AB(Ex và AB nằm trên cùng mặt phẳng bờ chứa tia BE)
Ta có: Ex//AB
AB//FG
Do đó: Ex//FG
Ex//AB
=>\(\widehat{BEx}=\widehat{CBA}\)(hai góc đồng vị)
=>\(\widehat{xEB}=49^0\)
Ta có: Ex//FG
=>\(\widehat{xEF}+\widehat{EFG}=180^0\)
=>\(\widehat{xEF}=180^0-120^0=60^0\)
\(\widehat{BEF}=\widehat{xEB}+\widehat{xEF}=49^0+60^0=109^0\)