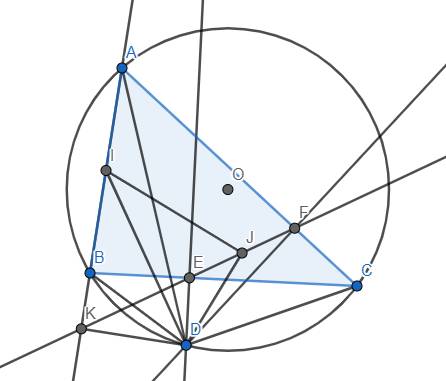
Cho tam giác ABC có ba góc nhọn nội tiếp đường tròn(O).
Các đường cao AD, BE, CF cắt nhau tại H và cắt đường tròn (O) lần lượt tại M, N, P. CMR:
a/. Các tứ giác AEHF, BCEF nội tiếp
b/ AD.BC = BE AC
c/. CMR BHM cân
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) Theo đề bài, ta có \(\widehat{DEC}=\widehat{DFC}=90^o\) \(\Rightarrow\) Tứ giác CDEF nội tiếp do có 2 đỉnh kề nhau E, F cùng nhìn cạnh CD dưới góc vuông. \(\Rightarrow\widehat{DFE}=\widehat{DCE}=\widehat{DCB}=\widehat{DAB}\) (do tứ giác ABDC nội tiếp nên \(\widehat{DCB}=\widehat{DAB}\)). Từ đó suy ra đpcm.
b) Có \(\widehat{KBD}=\widehat{ACD}\) (do tứ giác ABDC nội tiếp) và \(\widehat{ACD}=\widehat{KED}\) (do tứ giác CDEF nội tiếp) \(\Rightarrow\widehat{KBD}=\widehat{KED}\) \(\Rightarrow\) Tứ giác DKBE nội tiếp.
Mặt khác, \(\widehat{BDA}=\widehat{BCA}=\widehat{EDF}\) và \(\widehat{BAD}=\widehat{BCD}=\widehat{EFD}\)
\(\Rightarrow\Delta DBA~\Delta DEF\left(g.g\right)\)\(\Rightarrow\dfrac{DA}{DF}=\dfrac{DB}{DE}\) \(\Rightarrow DA.DE=DB.DF\)
c) \(\Delta DBA~\Delta DEF\Rightarrow\dfrac{DB}{DE}=\dfrac{AB}{EF}=\dfrac{2BI}{2EJ}=\dfrac{BI}{EJ}\) . Lại có \(\widehat{DBI}=\widehat{DEJ}\) nên \(\Delta DBI~\Delta DEJ\left(c.g.c\right)\) \(\Rightarrow\widehat{DIB}=\widehat{DJE}\) hay \(\widehat{DIK}=\widehat{DJK}\) \(\Rightarrow\) Tứ giác DJIK nội tiếp \(\Rightarrow\) \(\widehat{DJI}=180^o-\widehat{DKI}\) . Lại có \(\widehat{DKI}=180^o-\widehat{BED}=90^o\) (do tứ giác DKBE nội tiếp) \(\Rightarrow\widehat{DJI}=90^o\) \(\Rightarrow\) đpcm

a. Để có một trận đấu ta có 5 cách chọn đội thứ nhất, 4 cách chọn đội thứ 2. Vì mỗi đội chỉ đấu với nhau 1 trận nên số trận đấu của bảng đấu là: 5 .4 : 2 = 10 trận.
b. Mỗi trận đấu tổng điểm của hai đội là 3 điểm hoặc 2 điểm
Tổng điểm tối đa của 5 đội là: 10 . 3 = 30 điểm
Tổng điểm thực tế của 5 là: 10 + 9 + 6 + 4 +0 =29 điểm
Điểm thực tế ít hơn điểm tối đa là 1 nên có 1 trận hòa.
Hai đội A và D hòa nhau, vì điểm 2 đội không chia hết cho 3.

Theo định lý vi-ét ta có: x1+x2 = -(-4/2)=2
x1.x2= -3/2
Ta có: A = (x1-x2)2 = (x1+x2)2 - 4.x1.x2 = 22 - 4.(-3/2) = 4 + 6 = 10