Giải các bất phương trình ẩn $x$ sau:
a) $\dfrac{x+2 \, 004}{2 \, 005}+\dfrac{x+2 \, 005}{2 \, 006}<\dfrac{x+2 \, 006}{2 \, 007}+\dfrac{x+2 \, 007}{2 \, 008}$.
b) $\dfrac{x-2}{2 \, 002}+\dfrac{x-4}{2 \, 000}<\dfrac{x-3}{2 \, 001}+\dfrac{x-5}{1 \, 999}$.
c) $\dfrac{x-ab}{a+b}+\dfrac{x-bc}{b+c}+\dfrac{x-ac}{a+c}>a+b+c$ với $(a, \, b, \, c>0)$ .

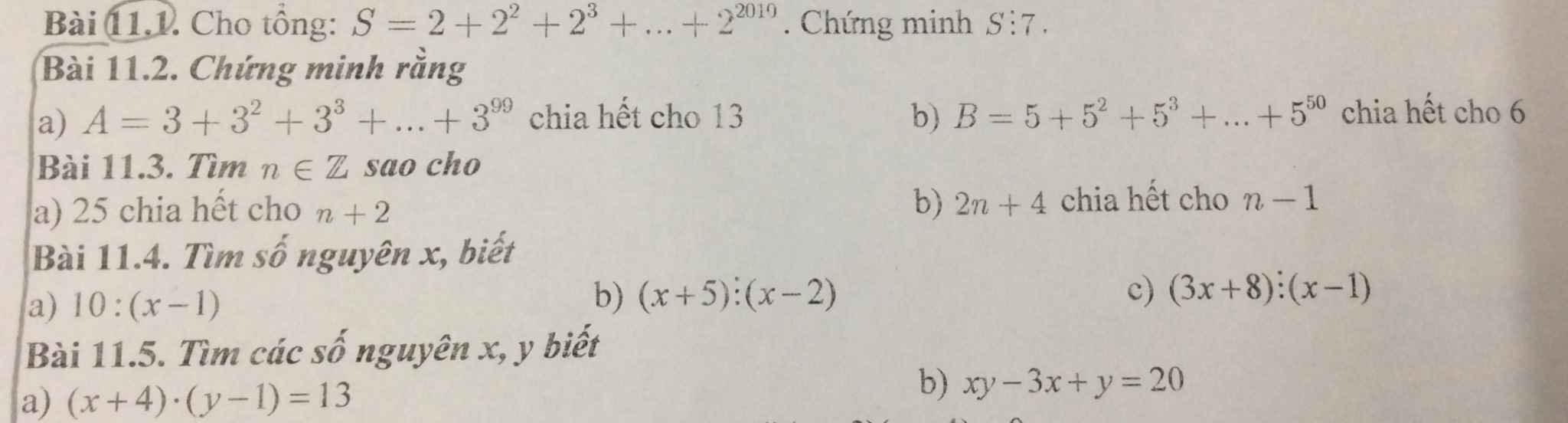
a) \(\dfrac{x+2004}{x+2005}+\dfrac{x+2005}{2006}< \dfrac{x+2006}{2007}+\dfrac{x+2007}{2008}\\ \Rightarrow\left(\dfrac{x+2004}{2005}-1\right)+\left(\dfrac{x+2005}{2006}-1\right)< \left(\dfrac{x+2006}{2007}-1\right)+\left(\dfrac{x+2007}{2008}-1\right)\\ \Rightarrow\dfrac{x-1}{2005}+\dfrac{x-1}{2006}< \dfrac{x-1}{2007}+\dfrac{x-1}{2008}\\ \Rightarrow\dfrac{x-1}{2005}+\dfrac{x-1}{2006}-\dfrac{x-1}{2007}-\dfrac{x-1}{2008}< 0\\ \)
\(\Rightarrow\left(x-1\right)\left(\dfrac{1}{2005}+\dfrac{1}{2006}-\dfrac{1}{2007}-\dfrac{1}{2008}\right)< 0\left(a\right)\)
Nhận thấy: \(\dfrac{1}{2005}>\dfrac{1}{2007},\dfrac{1}{2006}>\dfrac{1}{2008}\\ \Rightarrow\dfrac{1}{2005}-\dfrac{1}{2007}>0,\dfrac{1}{2006}-\dfrac{1}{2008}>0\\ \Rightarrow\dfrac{1}{2005}+\dfrac{1}{2006}-\dfrac{1}{2007}-\dfrac{1}{2008}>0\)
\(\left(a\right)\Rightarrow x-1< 0\Leftrightarrow x< 1\)
Vậy \(S=\left\{x|x< 1\right\}\)
b) \(\dfrac{x-2}{2002}+\dfrac{x-4}{2000}< \dfrac{x-3}{2001}+\dfrac{x-5}{1999}\\ \Rightarrow\left(\dfrac{x-2}{2002}-1\right)+\left(\dfrac{x-4}{2000}-1\right)< \left(\dfrac{x-3}{2001}-1\right)+\left(\dfrac{x-5}{1999}-1\right)\\ \Rightarrow\dfrac{x-2004}{2002}+\dfrac{x-2004}{2000}< \dfrac{x-2004}{2001}+\dfrac{x-2004}{1999}\\ \Rightarrow\dfrac{x-2004}{2002}+\dfrac{x-2004}{2000}-\dfrac{x-2004}{2001}-\dfrac{x-2004}{1999}< 0\\ \)
\(\Rightarrow\left(x-2004\right)\left(\dfrac{1}{2002}+\dfrac{1}{2000}-\dfrac{1}{2001}-\dfrac{1}{1999}\right)< 0\left(b\right)\)
Nhận thấy: \(\dfrac{1}{2002}< \dfrac{1}{2001},\dfrac{1}{2000}< \dfrac{1}{1999}\Rightarrow\dfrac{1}{2002}-\dfrac{1}{2001}< 0,\dfrac{1}{2000}-\dfrac{1}{1999}< 0\\ \Rightarrow\dfrac{1}{2002}+\dfrac{1}{2000}-\dfrac{1}{2001}-\dfrac{1}{1999}< 0\)
\(\left(b\right)\Rightarrow x-2004>0\Leftrightarrow x>2004\)