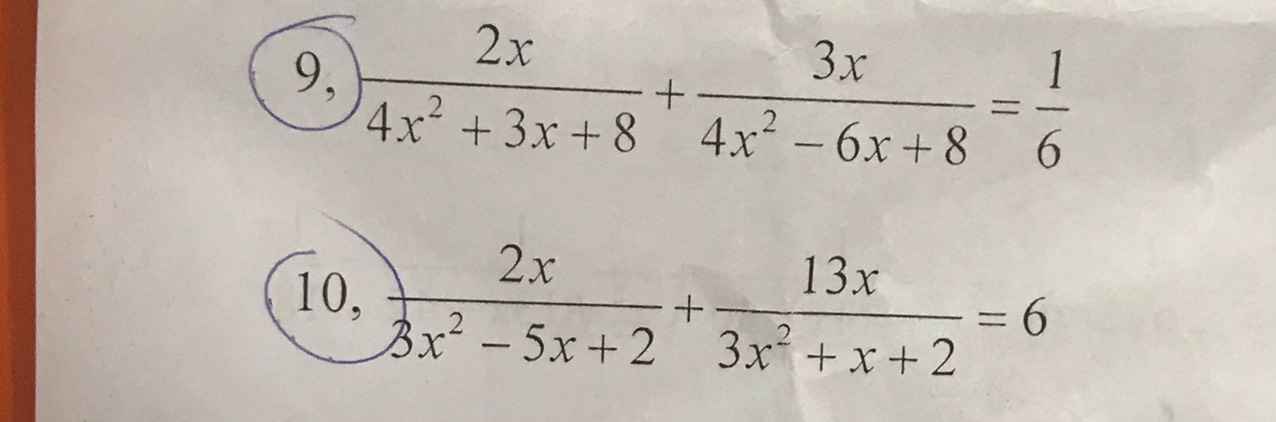Bài 4:

d: 

ĐKXĐ: \(x\notin\left\{1;-1;2;-2\right\}\)

\(\dfrac{x+4}{x-1}+\dfrac{x-4}{x+1}=\dfrac{x+8}{x-2}+\dfrac{x-8}{x+2}+6\)

=>\(\dfrac{\left(x+4\right)\left(x+1\right)+\left(x-4\right)\left(x-1\right)}{\left(x-1\right)\left(x+1\right)}=\dfrac{\left(x+8\right)\left(x+2\right)+\left(x-8\right)\left(x-2\right)+6\left(x-2\right)\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\)

=>\(\dfrac{2x^2+8}{\left(x-1\right)\left(x+1\right)}=\dfrac{2x^2+32+6x^2-24}{\left(x-2\right)\left(x+2\right)}\)

=>\(\dfrac{2x^2+8}{x^2-1}=\dfrac{8x^2+8}{x^2-4}\)

=>\(\left(2x^2+8\right)\left(x^2-4\right)=\left(8x^2+8\right)\left(x^2-1\right)\)

=>\(2x^4-32=8x^4-8\)

=>\(-6x^4=24\)

=>\(x^4=-4\left(loại\right)\)

Vậy: Phương trình vô nghiệm

c:

ĐKXĐ: \(x\notin\left\{-1;-3;-8;-10\right\}\)

 \(\dfrac{2}{x^2+4x+3}+\dfrac{5}{x^2+11x+24}+\dfrac{2}{x^2+18x+80}=\dfrac{9}{52}\)

=>\(\dfrac{2}{\left(x+1\right)\left(x+3\right)}+\dfrac{5}{\left(x+3\right)\left(x+8\right)}+\dfrac{2}{\left(x+8\right)\left(x+10\right)}=\dfrac{9}{52}\)

=>\(\dfrac{1}{x+1}-\dfrac{1}{x+3}+\dfrac{1}{x+3}-\dfrac{1}{x+8}+\dfrac{1}{x+8}-\dfrac{1}{x+10}=\dfrac{9}{52}\)

=>\(\dfrac{1}{x+1}-\dfrac{1}{x+10}=\dfrac{9}{52}\)

=>\(\dfrac{9}{\left(x+1\right)\left(x+10\right)}=\dfrac{9}{52}\)

=>(x+1)(x+10)=52

=>\(x^2+11x-42=0\)

=>(x+14)(x-3)=0

=>\(\left[{}\begin{matrix}x=-14\left(nhận\right)\\x=3\left(nhận\right)\end{matrix}\right.\)

b: 

ĐXKĐ: \(x\notin\left\{-2;-3;-4;-5;-6\right\}\)\(\dfrac{1}{x^2+5x+6}+\dfrac{1}{x^2+7x+12}+\dfrac{1}{x^2+9x+20}+\dfrac{1}{x^2+11x+30}=\dfrac{1}{8}\)

=>\(\dfrac{1}{\left(x+2\right)\left(x+3\right)}+\dfrac{1}{\left(x+3\right)\left(x+4\right)}+\dfrac{1}{\left(x+4\right)\left(x+5\right)}+\dfrac{1}{\left(x+5\right)\left(x+6\right)}=\dfrac{1}{8}\)

=>\(\dfrac{1}{x+2}-\dfrac{1}{x+3}+\dfrac{1}{x+3}-\dfrac{1}{x+4}+\dfrac{1}{x+4}-\dfrac{1}{x+5}+\dfrac{1}{x+5}-\dfrac{1}{x+6}=\dfrac{1}{8}\)

=>\(\dfrac{1}{x+2}-\dfrac{1}{x+6}=\dfrac{1}{8}\)

=>\(\dfrac{x+6-x-2}{\left(x+2\right)\left(x+6\right)}=\dfrac{1}{8}\)

=>(x+2)(x+6)=32

=>\(x^2+8x-20=0\)

=>(x+10)(x-2)=0

=>\(\left[{}\begin{matrix}x=-10\left(nhận\right)\\x=2\left(nhận\right)\end{matrix}\right.\)

a: \(\dfrac{x^2}{x^2+2x+2}+\dfrac{x^2}{x^2-2x+2}-\dfrac{4x^2-20}{x^4+4}=\dfrac{322}{65}\)

=>\(\dfrac{x^2\left(x^2-2x+2\right)+x^2\left(x^2+2x+2\right)-4x^2+20}{\left(x^2+2x+2\right)\left(x^2-2x+2\right)}=\dfrac{322}{65}\)

=>\(\dfrac{x^4-2x^3+2x^2+x^4+2x^3+2x^2-4x^2+20}{x^4+4}=\dfrac{322}{65}\)

=>\(\dfrac{2x^4+20}{x^4+4}=\dfrac{322}{65}\)

=>\(322\left(x^4+4\right)=65\left(2x^4+20\right)\)

=>\(322x^4+1288-130x^4-1300=0\)

=>\(192x^4=12\)

=>\(x^4=\dfrac{1}{16}\)

=>\(\left[{}\begin{matrix}x=\dfrac{1}{2}\left(nhận\right)\\x=-\dfrac{1}{2}\left(nhận\right)\end{matrix}\right.\)

\(a)\sqrt{2+\sqrt{3}}-\sqrt{2-\sqrt{3}}\\ =\dfrac{\sqrt{4+2\sqrt{3}}}{\sqrt{2}}-\dfrac{\sqrt{4-2\sqrt{3}}}{\sqrt{2}}\\ =\dfrac{\sqrt{1^2+2\cdot1\cdot\sqrt{3}+\left(\sqrt{3}\right)^2}-\sqrt{\left(\sqrt{3}\right)^2-2\cdot\sqrt{3}\cdot1+1^2}}{\sqrt{2}}\\ =\dfrac{\sqrt{\left(\sqrt{3}+1\right)^2}-\sqrt{\left(\sqrt{3}-1\right)^2}}{\sqrt{2}}\\ =\dfrac{\sqrt{3}+1-\sqrt{3}+1}{\sqrt{2}}\\ =\dfrac{2}{\sqrt{2}}\\ =\sqrt{2}\) 

b) 

\(\sqrt{3+\sqrt{5}}-\sqrt{3-\sqrt{5}}-\sqrt{2}\\ =\dfrac{\sqrt{6+2\sqrt{5}}}{\sqrt{2}}-\dfrac{\sqrt{6-2\sqrt{5}}}{\sqrt{2}}-\sqrt{2}\\ =\dfrac{\sqrt{\left(\sqrt{5}\right)^2+2\cdot\sqrt{5}\cdot1+1^2}}{\sqrt{2}}-\dfrac{\sqrt{\left(\sqrt{5}\right)^2-2\cdot\sqrt{5}\cdot1+1^2}}{\sqrt{2}}-\sqrt{2}\\ =\dfrac{\sqrt{\left(\sqrt{5}+1\right)^2}}{\sqrt{2}}-\dfrac{\sqrt{\left(\sqrt{5}-1\right)^2}}{\sqrt{2}}-\sqrt{2}\\ =\dfrac{\sqrt{5}+1-\sqrt{5}+1-2}{\sqrt{2}}\\ =\dfrac{0}{\sqrt{2}}\\ =0\)

\(\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{45}\\\dfrac{y}{2}-\dfrac{x}{2}=28\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{45}\\\dfrac{y}{2}=\dfrac{x}{2}+28\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{x+56}=\dfrac{1}{45}\\y=x+56\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}45\left(x+56\right)+45x=x\left(x+56\right)\\y=x+56\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}90x+2520=x^2+56x\\y=x+56\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x^2-34x-2520=0\\y=x+56\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x=70\\x=-36\end{matrix}\right.\\y=x+56\end{matrix}\right.\)

Khi x = 70 => y = 70 + 56 = 126

Khi x = -36 => y = (-36) + 56 = 20

a.

Gọi \(\left\{{}\begin{matrix}n_{CaCO_3}=x\left(mol\right)\\n_{MgCO_3}=y\left(mol\right)\end{matrix}\right.\)

Theo đề có hệ phương trình: \(\left\{{}\begin{matrix}100x+84y=4,68\\x+y=\dfrac{1,2395}{24,79}=0,05\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=0,03\\y=0,02\end{matrix}\right.\)

\(m_{CaCO_3}=0,03.100=3\left(g\right),m_{MgCO_3}=0,02.84=1,68\left(g\right)\)

b.

\(CM_{HCl}=\dfrac{2\left(x+y\right)}{0,25}=0,4\left(M\right)\)

\(n_{CO_2}=\dfrac{1,2395}{24,79}=0,05\left(mol\right)\)

PTHH:

\(CaCO_3+2HCl\rightarrow CaCl_2+H_2O+CO_2\)

x                2x             x             x           x 

\(MgCO_3+2HCl\rightarrow MgCl_2+H_2O+CO_2\)

y                    2y             y            y             y 

Có hệ PT:

\(\left\{{}\begin{matrix}100x+84y=4,68\\x+y=0,05\end{matrix}\right.\)

\(\Leftrightarrow x=0,03;y=0,02\)

\(a,m_{CaCO_3}=0,03.100=3\left(g\right)\)

\(m_{MgCO_3}=4,68-3=1,68\left(g\right)\)

b, \(C_{M\left(HCl\right)}=\dfrac{0,1}{0,25}=\dfrac{2}{5}\left(M\right)\)

\(C_{M\left(CaCl_2\right)}=\dfrac{0,03}{0,25}=\dfrac{3}{25}\left(M\right)\)

\(C_{M\left(MgCl_2\right)}=\dfrac{0,02}{0,25}=\dfrac{2}{25}\left(M\right)\)

Sửa đề: B là giao điểm có hoành độ dương của (P) và (d)

Phương trình hoành độ giao điểm của (P) và (d):

−x² = x − 2

x² + x − 2 = 0

x² − x + 2x − 2 = 0
(x² − x) + (2x − 2) = 0

x(x − 1) + 2(x− 1) = 0

(x − 1)(x + 2) = 0

x − 1 = 0 hoặc x + 2 = 0

*) x − 1 = 0

x = 1

y = −1² = −1

B(1; −1)

*) x + 2 = 0

x = −2

y = −(−2)² = −4

A(−2; −4)

* Phương trình đường thẳng OB:

Gọi (d'): y = ax + b là phương trình đường thẳng OB

Do (d') đi qua O nên b = 0

=> (d'): y = ax

Do (d') đi qua B(1; −1) nên:

a = −1

=> (d'): y = −x

Gọi (d''): y = a'x + b' là đường thẳng đi qua A(−2; −4)

Do (d'') // (d') nên a' = −1

=> (d''): y = −x + b

Do (d'') đi qua A(−2; −4) nên:

−(−2) + b = −4

b = −4 − 2

b = −6

=> (d''): y = −x − 6

Kẻ đường cao BD của tam giác ABC \(\left(D\in AC\right)\)

Khi đó \(AD=AB.cosA=c.cosA\)

\(BD=AB.sinA=c\sqrt{1-cos^2A}\)

\(CD=AC-AD=b-c.cosA\)

Tam giác BCD vuông tại D

\(\Rightarrow BC^2=CD^2+BD^2\)

\(\Leftrightarrow a^2=\left(b-c.cosA\right)^2+\left(c\sqrt{1-cos^2A}\right)^2\)

\(\Leftrightarrow a^2=b^2-2bc.cosA+c^2.cos^2A+c^2\left(1-cos^2A\right)\)

\(\Leftrightarrow a^2=b^2+c^2-2bc.cosA\)

Ta có đpcm.

5)

a) \(3x+8y=26\)

 \(\Leftrightarrow y=\dfrac{26-3x}{8}\)

 Vì \(y\inℤ\) nên \(\dfrac{26-3x}{8}\inℤ\)

 \(\Rightarrow26-3x⋮8\)

 \(\Leftrightarrow3x\equiv2\left(mod8\right)\)

 Vì \(ƯCLN\left(3,8\right)=1\) nên đặt \(x=8q+r\left(0\le r< 8\right)\) thì:

 \(3\left(8q+r\right)\equiv2\left(mod8\right)\)

 \(\Leftrightarrow24q+3r\equiv2\left(mod8\right)\)

 \(\Leftrightarrow3r\equiv2\left(mod8\right)\)

 Thử từng trường hợp, ta thấy ngay \(r=6\).

 Vậy \(x=8q+6\) 

\(\Rightarrow y=\dfrac{26-3x}{8}=\dfrac{26-3\left(8q+6\right)}{8}=\dfrac{8-24q}{8}=1-3q\)

 Vậy phương trình đã cho có nghiệm nguyên là \(\left(8q+6,1-3q\right)\) với \(q\inℤ\) bất kì.

 b) Cho \(1-3q>0\Leftrightarrow q< \dfrac{1}{3}\) 

 Cho \(8q+6>0\Leftrightarrow q>-\dfrac{3}{4}\)

 Do đó \(-\dfrac{3}{4}< q< \dfrac{1}{3}\). Mà \(q\inℤ\Rightarrow q=0\)

 Thế vào \(x,y\), pt sẽ có nghiệm nguyên dương là \(\left(6;1\right)\)

 Câu 6 làm tương tự nhé bạn.

Điều chế Na từ NaCl: 

Đem dung dịch NaCl điện phân nóng chảy, ta thu được kim loại Na.

\(NaCl\underrightarrow{đpnc}Na+\dfrac{1}{2}Cl_2\)

Điều chế Na từ NaOH: 

Đem dung dịch NaOH phản ứng vừa đủ với dung dịch \(MgCl_2\) đến kết tủa tối đa, lọc kết tủa rồi đem dung dịch sau phản ứng điện phân nóng chảy, ta thu được kim loại Na.

\(2NaOH+MgCl_2\rightarrow Mg\left(OH\right)_2+2NaCl\)

\(NaCl\underrightarrow{đpnc}Na+\dfrac{1}{2}Cl_2\)

Đề bài là j thế bạn

Các phương trình bậc nhất 2 ẩn là: `3x-y=3;x+2y=8;y+3y=11`

Hệ số a,b,c của các pt là: 

+) `3x-y=3` có `a=3; b=-1;c=3` 

+) `x+2y=8` có `a=1;b=2;c=8` 

+) `y+3x=11` có `a=3;b=1;c=11`