Tìm nghiệm nguyên của phương trình: \(x^2-xy=6x-5y-8\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta đặt y = x + k với k \(\inℤ\)
Khi đó 3x2 - y2 - 2xy - 2x - 2y + 40 = 0
<=> 3x2 - (x + k)2 - 2x(x + k) - 2x - 2(x + k) + 40 = 0
<=> k2 + 4xk + 4x + 2k - 40 = 0
<=> (k + 1)2 + 4x(k + 1) = 41
<=> (k + 1)(4x + k + 1) = 41
Ta lập bảng ta được :
| k + 1 | 1 | 41 | -1 | -41 |
| 4x + k + 1 | 41 | 1 | -41 | -1 |
| x | 10 | -10 | -10 | 10 |
| k | 0 | 40 | -2 | -42 |
lại có y = x + k
ta được các cặp (x;y) cần tìm là (10;10) ; (-10 ; 30) ; (-10 ; -12) ; (10;-32)

\(a,P=\left[\dfrac{3\sqrt{a}}{\sqrt{a}+4}+\dfrac{\sqrt{a}}{\sqrt{a}-4}+\dfrac{4\left(a+2\right)}{16-a}\right]:\left(1-\dfrac{2\sqrt{a}+5}{\sqrt{a}+4}\right)\left(dk:a\ge0,a\ne16\right)\\ =\left[\dfrac{3\sqrt{a}}{\sqrt{a}+4}+\dfrac{\sqrt{a}}{\sqrt{a}-4}-\dfrac{4a+8}{a-16}\right]:\left(\dfrac{\sqrt{a}+4-2\sqrt{a}-5}{\sqrt{a}+4}\right)\)
\(=\left[\dfrac{3\sqrt{a}\left(\sqrt{a}-4\right)+\sqrt{a}\left(\sqrt{a}+4\right)-\left(4a+8\right)}{\left(\sqrt{a}-4\right)\left(\sqrt{a}+4\right)}\right].\dfrac{\sqrt{a}+4}{-\sqrt{a}-1}\)
\(=\dfrac{3a-12\sqrt{a}+a+4\sqrt{a}-4a-8}{\sqrt{a}-4}.\left(-\sqrt{a}-1\right)\\ =\dfrac{-8\sqrt{a}-8}{-\left(\sqrt{a}-4\right)\left(\sqrt{a}+1\right)}\\ =\dfrac{-8\left(\sqrt{a}+1\right)}{-\left(\sqrt{a}-4\right)\left(\sqrt{a}+1\right)}\\ =\dfrac{8}{\sqrt{a}-4}\)

A = \(\sqrt{3+2\sqrt{2}}\) - \(\sqrt{\dfrac{\sqrt{2}-1}{\sqrt{2}+1}}\)
A = \(\sqrt{2+2\sqrt{2}+1}\) - \(\sqrt{\dfrac{\left(\sqrt{2}-1\right)\left(\sqrt{2}-1\right)}{\left(\sqrt{2}+1\right)\left(\sqrt{2}-1\right)}}\)
A = \(\sqrt{\left(\sqrt{2}+1\right)^2}\) - \(\sqrt{\dfrac{\left(\sqrt{2}-1\right)^2}{2-1}}\)
A = \(\sqrt{2}\) + 1 - \(\sqrt{2}\) + 1
A = 2
A = \(\dfrac{2+\sqrt{3}}{\sqrt{7-4\sqrt{3}}}\) - \(\dfrac{2-\sqrt{3}}{\sqrt{7+4\sqrt{3}}}\)
A = \(\dfrac{2+\sqrt{3}}{\sqrt{4-4\sqrt{3}+3}}\) - \(\dfrac{2-\sqrt{3}}{\sqrt{4+4\sqrt{3}+3}}\)
A = \(\dfrac{2+\sqrt{3}}{\sqrt{\left(2-\sqrt{3}\right)^2}}\) - \(\dfrac{2-\sqrt{3}}{\sqrt{\left(2+\sqrt{3}\right)^2}}\)
A = \(\dfrac{2+\sqrt{3}}{2-\sqrt{3}}\) - \(\dfrac{2-\sqrt{3}}{2+\sqrt{3}}\)
A = \(\dfrac{\left(2+\sqrt{3}\right)^2-\left(2-\sqrt{3}\right)^2}{\left(2-\sqrt{3}\right).\left(2+\sqrt{3}\right)}\)
A = \(\dfrac{\left(2+\sqrt{3}-2+\sqrt{3}\right)\left(2+\sqrt{3}+2-\sqrt{3}\right)}{4-3}\)
A = \(\dfrac{2\sqrt{3}.4}{1}\)
A = 8\(\sqrt{3}\)

`a,` Ta có `ΔABC` vuông tại `A,`
`=>` `HBA` là góc vuông, có số đo là `90^o`
`b,` Ta có `ΔABC` vuông tại `A`
`=>` `AH` là đường cao của `ΔABC`
Theo định lý Euclid, trong một tam giác vuông, đường cao chia tam giác thành hai tam giác nhỏ có tỉ lệ bằng độ dài các cạnh gần góc vuông.
Vậy ta có: `(AD)/(AB)` `=` `(HD)/(HC)`
Vì `ΔABC` vuông tại `A`
`=> AB` `= AC`
`=>` `(AD)/(AC)` `=` `(HD)/(HC)`
Nhân cả hai vế của phương trình trên với `AC,` ta có:
`AD .` `(AC)/(AC)` `= HD .` `(HC)/(HC)`
`AD =` `HD.``HC`
`=>` `AD.AC` `=` `HB.HC.`

Bài 36:
a.
Nếu $a,b>0$ thì:
\(A=\frac{\sqrt{a}-\sqrt{b}}{\sqrt{b}}.\frac{\sqrt{a}+\sqrt{b}}{\sqrt{a}}:\frac{a^2-b^2}{ab}\\ =\frac{a-b}{\sqrt{ab}}.\frac{ab}{(a-b)(a+b)}=\frac{\sqrt{ab}}{a+b}\)
Nếu $a,b<0$ thì:
\(A=\frac{\sqrt{-a}-\sqrt{-b}}{\sqrt{-b}}.\frac{\sqrt{-a}+\sqrt{-b}}{\sqrt{-a}}:\frac{a^2-b^2}{ab}\\ =\frac{(-a)-(-b)}{\sqrt{ab}}.\frac{ab}{(a-b)(a+b)}=\frac{\sqrt{ab}}{-(a+b)}\)
Vậy không có đáp án đúng.
b.
$b=1$ thì $b>0, a>0$.
Khi đó: $A=\frac{\sqrt{ab}}{a+b}=2$
$\Leftrightarrow \frac{\sqrt{a}}{a+1}=2$
$\Leftrightarrow \sqrt{a}=2a+2$
$\Leftrightarrow 2a-\sqrt{a}+2=0$
$\Leftrightarrow (\sqrt{a}-0,5)^2+a+1,75=0$
$\Leftrightarrow (\sqrt{a}-0,5)^2+a=-1,75<0$ (vô lý với mọi $a>0$)
Đáp án D.

\(\dfrac{3+2\sqrt{3}}{\sqrt{3}}+\dfrac{2\sqrt{2}}{\sqrt{2}+1}-\left(3+\sqrt{3}-2\sqrt{2}\right)\\ =\dfrac{\sqrt{3}\left(\sqrt{3}+2\right)}{\sqrt{3}}+\dfrac{2\sqrt{2}\left(\sqrt{2}-1\right)}{\left(\sqrt{2}-1\right)\left(\sqrt{2}+1\right)}-3-\sqrt{3}+2\sqrt{2}\\ =\sqrt{3}+2+\dfrac{4-2\sqrt{2}}{2-1}-3-\sqrt{3}+2\sqrt{2}\\ =-1+2\sqrt{2}+\dfrac{4-2\sqrt{2}}{1}\\ =-1+2\sqrt{2}+4-2\sqrt{2}\\ =3\)

1) \(\dfrac{\sqrt[]{6}+\sqrt[]{14}}{2\sqrt[]{3}+\sqrt[]{28}}=\dfrac{\sqrt[]{2}\left(\sqrt[]{3}+\sqrt[]{7}\right)}{2\left(\sqrt[]{3}+\sqrt[]{7}\right)}=\dfrac{\sqrt[]{2}}{2}\)
2) \(\dfrac{\sqrt[]{5}-\sqrt[]{10}}{\sqrt[]{3}-\sqrt[]{6}}=\dfrac{\sqrt[]{5}\left(1-\sqrt[]{2}\right)}{\sqrt[]{3}\left(1-\sqrt[]{2}\right)}=\sqrt[]{\dfrac{5}{3}}\)
3) \(...=\dfrac{4\sqrt[]{2}-2\sqrt[]{3}}{3\sqrt[]{2}-4\sqrt[]{3}}-\dfrac{\sqrt[]{5}+\sqrt[]{27}}{\sqrt[]{6}\left(\sqrt[]{5}+\sqrt[]{27}\right)}\)
\(=\dfrac{2\left(2\sqrt[]{2}-\sqrt[]{3}\right)}{3\sqrt[]{2}-4\sqrt[]{3}}-\dfrac{1}{\sqrt[]{6}}\)
\(=\dfrac{2\sqrt[]{6}\left(2\sqrt[]{2}-\sqrt[]{3}\right)-\left(3\sqrt[]{2}-4\sqrt[]{3}\right)}{\sqrt[]{6}\left(3\sqrt[]{2}-4\sqrt[]{3}\right)}\)
\(=\dfrac{8\sqrt[]{3}-6\sqrt[]{2}-3\sqrt[]{2}+4\sqrt[]{3}}{6\sqrt[]{3}-12\sqrt[]{3}}\)
\(=\dfrac{12\sqrt[]{3}-9\sqrt[]{2}}{-6\sqrt[]{3}}=-2+\sqrt[]{\dfrac{3}{2}}\)
Bài 4 bạn tự làm nhé
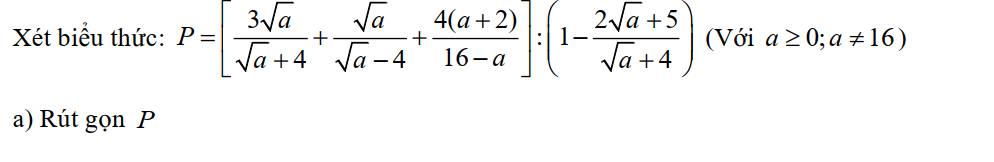

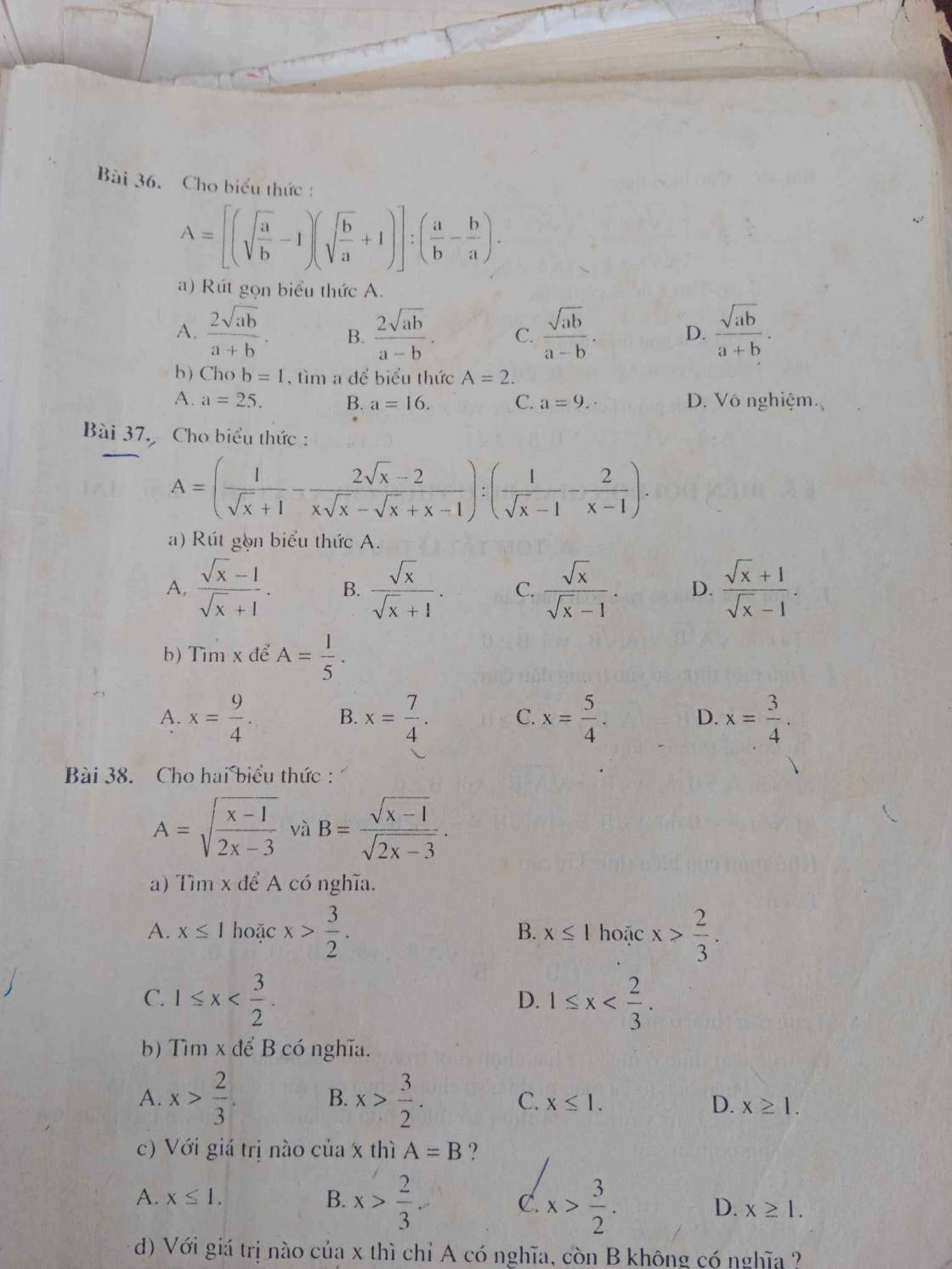
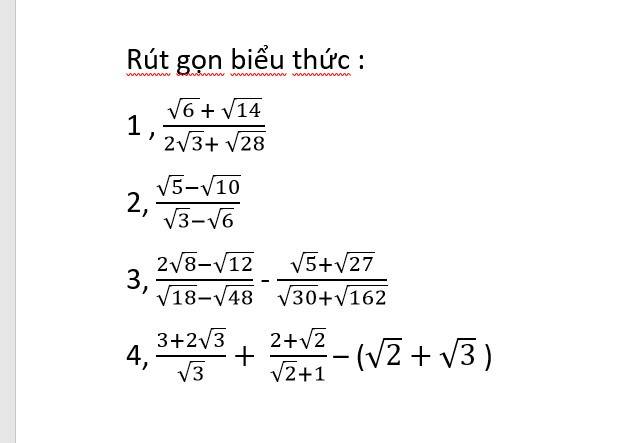
Biện pháp tu từ được sử dụng trong câu "sương vô tình đậu trên mắt rưng rưng" là sự lặp lại âm tiết "rưng rưng". Tác dụng của biện pháp này là tạo ra hiệu ứng âm thanh đặc biệt, tăng cường tính hài hòa và nhấn mạnh sự mơ hồ, mờ ảo của cảnh tượng mà câu muốn diễn tả. Ngoài ra, biện pháp tu từ còn giúp tạo ra sự nhấn mạnh, tăng cường tính cảm xúc và sự chú ý của người đọc đối với câu. có đúng khum thì ko bít nữa nhớ tick ạ
nhầm bài r bạn