Tìm các số nguyên n để phân số sau có giá trị nguyên: \(\dfrac{n-3}{n-5}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Giải:
Tổng số học sinh của lớp 6a luôn không đổi,
Số học sinh giỏi kì I bằng:
3 : (3 + 7) = \(\dfrac{3}{10}\) (tổng số học sinh của lớp 6a)
Số học sinh giỏi kì II bằng:
2 : (2 + 3) = \(\dfrac{2}{5}\) (tổng số học sinh của lớp 6a)
4 học sinh ứng với phân số là:
\(\dfrac{2}{5}-\dfrac{3}{10}\) = \(\dfrac{1}{10}\) (tổng số học sinh của lớp 6a)
Tổng số học sinh của lớp 6a là:
4 : \(\dfrac{1}{10}\) = 40 (học sinh)
Kết luận: lớp 6a có 40 học sinh.
Gọi a là số HSG ở kì 1.
Vì số HSG kì 1 bằng 3/7 số còn lại:
-> a=\(\dfrac{3}{3+7}\)=\(\dfrac{3}{10}\) (số hs cả lớp)
Gọi b là số HSG ở kì 2.
-> b=\(\dfrac{2}{3+2}\)=\(\dfrac{2}{5}\) (số hs cả lớp)
4 hs chiếm số phần của cả lớp là:
\(\dfrac{2}{5}\)-\(\dfrac{3}{10}\)=\(\dfrac{1}{10}\) (số học sinh cả lớp)
Số hs lớp 6a là:
4:\(\dfrac{1}{10}\)=40 (học sinh)
Đáp số: 40 học sinh.

26.1252
= 26.(53)2
=26.56
= (2.5)6
= 106
= 1000000
\(2^6.125^2\)
=\(2^6.\left(5^3\right)^2\)
=\(2^6.5^6\)
=\(\left(2.5\right)^6\)
=\(10^6=1000000\)

\(\dfrac{2}{5}\)\(x^2\) = \(\dfrac{1}{3}\)\(x\)
\(\dfrac{2}{5}x^2\) - \(\dfrac{1}{3}x\) = 0
\(x\).(\(\dfrac{2}{5}\)\(x\) - \(\dfrac{1}{3}\)) = 0
\(\left[{}\begin{matrix}x=0\\\dfrac{2}{5}x=\dfrac{1}{3}\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=0\\x=\dfrac{1}{3}:\dfrac{2}{5}\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=0\\x=\dfrac{5}{6}\end{matrix}\right.\)
vậy \(x\) \(\in\) {0; \(\dfrac{5}{6}\)}

Giải:
Cứ 1 điểm sẽ tạo với n - 1 điểm còn lại n - 1 đường thẳng
với n điểm sẽ tạo được (n - 1)n đường thẳng
Theo cách tính trên mỗi đường thẳng sẽ được tính hai lần nên thực tế số đường thẳng được tạo là:
(n - 1).n : 2 (đường thẳng)
Theo bài ra ta có: (n - 1)n : 2 = 105
(n - 1)n = 105.2
(n - 1).n = 210
(n - 1).n = 14.15
n = 15
Vậy n = 15

Olm chào em đây là dạng toán nâng cao chuyên đề toán tư duy logic, cấu trúc thi chuyên, thi học sinh giỏi các cấp, thi violympic. Hôm nay, Olm sẽ hướng dẫn các em giải chi tiết dạng này như sau:
Giải
Bình chứa \(\dfrac{1}{2}\) lượng nước hơn bình chứa \(\dfrac{1}{3}\) lượng nước là:
\(\dfrac{1}{2}\) - \(\dfrac{1}{3}\) = \(\dfrac{1}{6}\) (lượng nước)
\(\dfrac{1}{6}\) lượng nước ứng với:
700 - 500 = 200 (g)
\(\dfrac{1}{2}\) lượng nước gấp \(\dfrac{1}{6}\) lượng nước số lần là:
\(\dfrac{1}{2}\) : \(\dfrac{1}{6}\) = 3 (lần)
\(\dfrac{1}{2}\) lượng nước nặng là:
200 x 3 = 600 (g)
Vò bình nặng là:
700 - 600 = 100 (g)
Khi đầy bình nước thì bình cân nặng:
600 x 2 + 100 = 1300 (g)
Đáp số: 1300 g

\(B=2019\cdot\left(2020-164\right)-2020\left(2019-164\right)\)
\(=2019\cdot2020-2019\cdot164-2020\cdot2019+2020\cdot164\)
\(=2020\cdot164-2019\cdot164=164\)

Ngày thứ nhất đọc được:
\(80\cdot25\%=20\left(trang\right)\)
Sau ngày 1 còn lại 80-20=60(trang)
Ngày thứ hai đọc được: \(60\cdot\dfrac{1}{3}=20\left(trang\right)\)
=>Số trang còn lại sau ngày 2 là 60-20=40(trang)
Ngày thứ 4 đọc được:
\(40\left(1-60\%\right)=40\cdot0,4=16\left(bạn\right)\)

Đây là toán nâng cao chuyên đề chuyển động, cấu trúc thi chuyên, thi học sinh giỏi. Hôm nay Olm sẽ hướng dẫn các em giải chi tiết dạng này bằng phương pháp lập phương trình như sau:
Giải:
Gọi quãng đường AB có độ dài là \(x\) (km); \(x\) > 0
Thời gian ô tô đi \(\dfrac{2}{3}\) quãng đường đầu là: \(\dfrac{2}{3}\)\(x\) : 40 = \(\dfrac{1}{60}\)\(x\) (giờ)
Quãng đường còn lại là: \(x\) - \(\dfrac{2}{3}\)\(x\) = \(\dfrac{1}{3}\)\(x\) (km)
Thời gian ô tô đi quãng đường còn lại là;
\(\dfrac{1}{3}\)\(x\) : 60 = \(\dfrac{1}{180}x\) (giờ)
Vì thời gian đi bằng thời gian về nên thời gian về của người đó là;
\(\dfrac{1}{60}x+\dfrac{1}{180}x\) = \(\dfrac{1}{45}\)\(x\) (giờ)
Vận tốc của người đó khi về là:
\(x\) :( \(\dfrac{1}{45}\)\(x\)) = 45 (km/h)
Kết luận vận tốc khi về của người đó là 45 km/h

a: \(\widehat{AOC}+\widehat{AOB}=180^0\)(hai góc kề bù)
=>\(\widehat{AOC}+124^0=180^0\)
=>\(\widehat{AOC}=56^0\)
b: 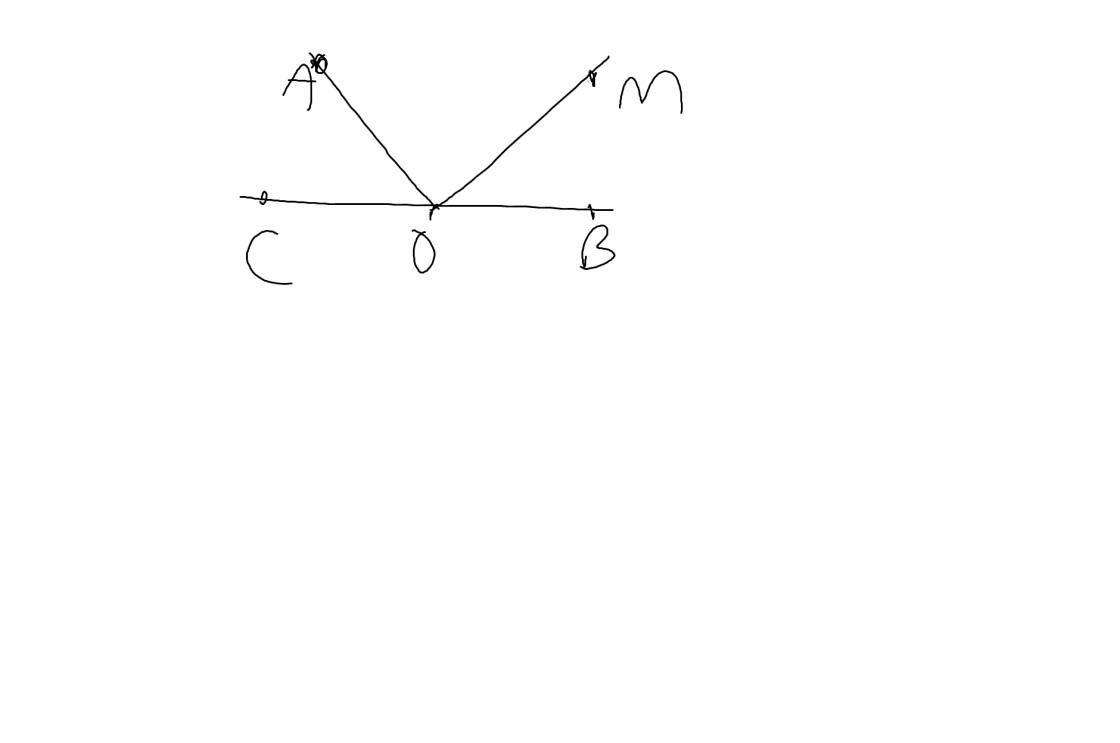
ĐKXĐ: n<>5
Để \(\dfrac{n-3}{n-5}\) là số nguyên thì \(n-3⋮n-5\)
=>\(n-5+2⋮n-5\)
=>\(2⋮n-5\)
=>\(n-5\in\left\{1;-1;2;-2\right\}\)
=>\(n\in\left\{6;4;7;3\right\}\)