cho đường thẳng d: y=(m-1)*x+2 khoẳng cách từ điểm M(2;1) đến (d) là \(\dfrac{3\sqrt{x}}{2}\). Khi đó tổng các giá trị của m thỏa mãn là
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(A=\sqrt[]{1+2015^2+\dfrac{2015^2}{2016^2}}+\dfrac{2015}{2016}\)
\(\Leftrightarrow A=\sqrt[]{\left(1+2015\right)^2-2.2015+\dfrac{2015^2}{\left(2015+1\right)^2}}+\dfrac{2015}{2016}\)
\(\Leftrightarrow A=\sqrt[]{\left(1+2015-\dfrac{2015}{2015+1}\right)^2}+\dfrac{2015}{2016}\)
\(\Leftrightarrow A=\left|1+2015-\dfrac{2015}{2016}\right|+\dfrac{2015}{2016}\)
\(\Leftrightarrow A=1+2015-\dfrac{2015}{2016}+\dfrac{2015}{2016}\)
\(\Leftrightarrow A=1+2015=2016\)

Lời giải:
Đặt $x=a; \frac{y}{2}=b$ thì bài toán trở thành:
Tìm min $A=\frac{1}{a^2+1}+\frac{1}{b^2+1}+2ab$ với $ab\geq 1$
----------------------------------
Với $ab\geq 1$, ta có BĐT khá quen thuộc:
$\frac{1}{a^2+1}+\frac{1}{b^2+1}\geq \frac{2}{ab+1}$ (để cm BĐT này bạn chỉ cần biến đổi tương đương)
Áp dụng vào bài và sử dụng thêm BĐT AM-GM:
$A\geq \frac{2}{ab+1}+2ab=\frac{2}{ab+1}+\frac{ab+1}{2}+\frac{3ab-1}{2}$
$\geq 2\sqrt{\frac{2}{ab+1}.\frac{ab+1}{2}}+\frac{3ab-1}{2}$
$=2+\frac{3ab-1}{2}\geq 2+\frac{3.1-1}{2}=3$
Vậy $A_{\min}=3$.

Đặt \(A=\sqrt{a+1}+\sqrt{a+3}\)
\(\Rightarrow A^2=2a+4+2\sqrt{\left(a+1\right)\left(a+3\right)}\)
Đặt \(B=2\sqrt{a+2}\)
\(\Rightarrow B^2=4a+8\)
Xét hiệu \(B^2-A^2=2a+4-2\sqrt{\left(a+1\right)\left(a+3\right)}\)
Áp dụng BĐT Cô-si, ta có \(2a+4=\left(a+1\right)+\left(a+3\right)\) \(>2\sqrt{\left(a+1\right)\left(a+3\right)}\)
(Dấu "=" không thể xảy ra vì khi đó sẽ suy ra đẳng thức vô lí là \(1=3\))
Từ đó suy ra \(B^2-A^2>0\) \(\Leftrightarrow B^2>A^2\), và do A, B dương nên suy ra \(B>A\). Nói cách khác, \(2\sqrt{a+2}>\sqrt{a+1}+\sqrt{a+3}\)
sao em bấm máy tính thì dấu bằng xảy ra khi x=10000000 vậy ạ.

tick cho mình cái
Đáp án 2
a, Cmr: MC=2c
Gọi N là trung điểm của BC.
Ta có:
AN = NC = BC/2 = c/2M là trung điểm của AC, nên:
AM = MC = c/2Do đó:
MC = 2cb, Tính độ dài AC, BC theo c
Ta có:
AC = AB = c BC = 2MC = 2cc, Tinh tỉ số lượng giác các góc
Ta có:
sin A = BC/AB = 2c/c = 2 cos A = AB/AC = c/c = 1 tan A = BC/AC = 2c/c = 2 sin B = AC/BC = c/2c = 1/2 cos B = AB/BC = c/2c = 1/2 tan B = AC/AB = c/c = 1 sin C = AB/BC = c/2c = 1/2 cos C = AC/BC = c/2c = 1/2 tan C = AB/AC = c/c = 1Kết luận
- MC = 2c
- AC = c
- BC = 2c
- sin A = 2, cos A = 1, tan A = 2
- sin B = 1/2, cos B = 1/2, tan B = 1
- sin C = 1/2, cos C = 1/2, tan C = 1
Đáp án 3
a, Cmr: AABC – DBA và x^ = 2y(x+2y)
Ta có:
AH^2 = AB^2 - BH^2 = x^2 - (x/2)^2 = 3x^2/4Do đó:
AH = x^2/2Ta có:
SABC = 1/2 * BC * AB SDBA = 1/2 * BC * BD BD = AB/2 = x/2Do đó:
SABC - SDBA = 1/2 * BC * AB - 1/2 * BC * BD = 1/2 * BC * (AB - BD) = 1/2 * BC * (x - x/2) = 1/4 * BC * xDo đó:
SABC - SDBA = 1/4 * BC * x = 1/4 * 2y(x+2y)b, Tinh x và AH theo y
Từ (1), ta có:
AH = x^2/2Từ (2), ta có:
x^2/2 = 1/4 * 2y(x+2y) x^2 = 1/2 * 2y(x+2y) x^2 = y(x+2y) x^2 - yx - 2y^2 = 0Giải phương trình trên, ta có:
x = y ± √(y^2 + 8y^2) x = y ± 3yDo x > 0, nên x = y + 3y = 4y
Thay x = 4y vào (1), ta có:
AH = (4y)^2/2 = 8y^2/2 = 4yc, Tỉnh tỉ số lượng giác các góc
Ta có:
sin A = BC/AB = 2y/(4y) = 1/2
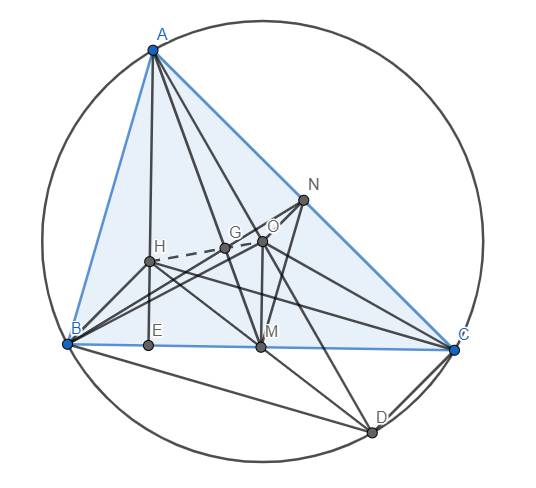
a) Ta chứng minh \(\Delta HAB~\Delta OMN\). Thật vậy, từ đề bài, dễ thấy H, O lần lượt là trực tâm và tâm đường tròn ngoại tiếp của tam giác ABC. Vẽ đường tròn ngoại tiếp này. Dựng đường kính AD của (O). AH cắt BC tại E.
Ta thấy \(\widehat{ACD}=\widehat{AEB}\left(=90^o\right)\) và \(\widehat{ADC}=\widehat{ABE}\) (góc nội tiếp cùng chắn \(\stackrel\frown{AC}\)). \(\Rightarrow\Delta ACD~\Delta AEB\left(g.g\right)\) \(\Rightarrow\widehat{BAH}=\widehat{CAO}\)
Mà \(\widehat{CAO}=\widehat{OCA}\), thêm vào đó tứ giác OMCN nội tiếp (vì \(\widehat{OMC}=\widehat{ONC}=90^o\)) nên \(\widehat{OMN}=\widehat{OCN}\). Do đó \(\widehat{HAB}=\widehat{OMN}\)
Hoàn toàn tương tự, ta suy ra \(\widehat{HBA}=\widehat{ONM}\). Từ đó suy ra \(\Delta HAB~\Delta OMN\left(g.g\right)\) (đpcm)
b) Ta thấy BH//CD\(\left(\perp AC\right)\) và CH//BD\(\left(\perp AB\right)\) nên tứ giác BDCH là hình bình hành. Mà M là trung điểm BC nên M cũng là trung điểm của DH. Lại có O là trung điểm của AD nên OM là đường trung bình của tam giác DHA \(\Rightarrow\left\{{}\begin{matrix}OM//AH\\OM=\dfrac{1}{2}AH\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}\widehat{HAG}=\widehat{GMO}\\\dfrac{AH}{OM}=\dfrac{GA}{GM}\left(=2\right)\end{matrix}\right.\)
\(\Rightarrow\Delta AHG~\Delta MOG\left(c.g.c\right)\) (đpcm)
c) Từ \(\Delta AHG~\Delta MOG\Rightarrow\widehat{AGH}=\widehat{MGO}\)
Do A, G, M thẳng hàng nên \(\widehat{AGH}+\widehat{HGM}=180^o\)
Từ đó suy ra \(\widehat{HGM}+\widehat{MGO}=180^o\) \(\Rightarrow\) H, O, G thẳng hàng.

Trong △ABC, ta có: AD là đường phân giác của (BAC)
Suy ra: (tính chất đường phân giác)
Mà AB = 15 (cm); AC = 20 (cm)
Nên
Suy ra: (tính chất tỉ lệ thức)
Suy ra:
Trong △ABC, ta có: AD là đường phân giác của (BAC)
Suy ra: (tính chất đường phân giác)
Mà AB = 15 (cm); AC = 20 (cm)
Nên
Suy ra: (tính chất tỉ lệ thức)
Suy ra:

B C A x y D E O I J G
a/
\(\widehat{BAC}=90^o\) (góc nt chắn nửa đường tròn) \(\Rightarrow AB\perp AC\Rightarrow AI\perp AC\)
\(OE\perp AC\) (Hai tiếp tuyến cùng xp từ 1 điểm thì đường thẳng nối điểm đó với tâm đường tròn vuông góc với đường thẳng nối hai tiếp điểm) \(\Rightarrow OJ\perp AC\)
=> AI//OJ (cùng vuông góc với AC) (1)
\(\widehat{BAC}=90^o\) (cmt) \(\Rightarrow AC\perp AB\Rightarrow AJ\perp AB\)
\(OD\perp AB\) (Hai tiếp tuyến cùng xp từ 1 điểm thì đường thẳng nối điểm đó với tâm đường tròn vuông góc với đường thẳng nối hai tiếp điểm) \(\Rightarrow OI\perp AB\)
=> AJ//OI (cùng vuông góc với AB) (2)
=> AIOJ là hình bình hành (Tứ giác có các cặp cạnh đối // với nhau từng đôi một là hbh)
\(\widehat{BAC}=90^o\) (cmt)
=> AIOJ là hình chữ nhật (Hình bình hành có 1 góc trong bằng 90 độ là HCN)
b/
Ta có
IA=IB (Hai tiếp tuyến cùng xp từ 1 điểm thì đường thẳng nối điểm đó với tâm đường tròn vuông góc và chia đôi đường thẳng nối hai tiếp điểm)
JA=JC (Hai tiếp tuyến cùng xp từ 1 điểm thì đường thẳng nối điểm đó với tâm đường tròn vuông góc và chia đôi đường thẳng nối hai tiếp điểm)
=> IJ là đường trung bình của tg ABC => IJ//BC
c/
G là trọng tâm tg ABC \(\Rightarrow OG=\dfrac{1}{3}AO\) không đổi
=> Khi A di chuyển trên đường tròn thì G di chuyển trên đường tròn đường kính OG
\(\widehat{BAC}=90^o\)

\(2xy^2+2x+3y^2=4\left(x;y\inℤ\right)\)
\(\Leftrightarrow2x\left(y^2+1\right)+3y^2+3-3=4\)
\(\Leftrightarrow2x\left(y^2+1\right)+3\left(y^2+1\right)=7\)
\(\Leftrightarrow\left(2x+3\right)\left(y^2+1\right)=7\)
\(\Leftrightarrow\left(2x+3\right);\left(y^2+1\right)\in U\left(7\right)=\left\{-1;1-7;7\right\}\)
\(TH1:\left\{{}\begin{matrix}2x+3=-1\\y^2+1=-7\left(loại\right)\end{matrix}\right.\)
\(TH2:\left\{{}\begin{matrix}2x+3=1\\y^2+1=7\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}2x=-2\\y^2=6\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=\pm\sqrt[]{6}\left(loại\right)\end{matrix}\right.\)
\(TH3:\left\{{}\begin{matrix}2x+3=-7\\y^2+1=-1\left(loại\right)\end{matrix}\right.\)
\(TH1:\left\{{}\begin{matrix}2x+3=7\\y^2+1=1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}2x=4\\y^2=0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=0\end{matrix}\right.\)
Vậy \(\left\{{}\begin{matrix}x=2\\y=0\end{matrix}\right.\) thỏa điều kiện đề bài
2xy² + 2x + 3y² = 4
2xy² + 2x + 3y² + 3 = 4 + 3
(2xy² + 2x) + (3y² + 3) = 7
2x(y² + 1) + 3(y² + 1) = 7
(y² + 1)(2x + 3) = 7
TH1: 2x + 3 = 1 và y² + 1 = 7
*) 2x + 3 = 1
2x = -2
x = -1 (nhận)
*) y² + 1 = 7
y² = 6
y = ±√6 (loại)
TH2: 2x + 3 = -1 và y² + 1 = -7
*) 2x + 3 = -1
2x = -4
x = -2 (nhận)
*) y² + 1 = -7
y² = -8 (vô lý)
TH3: 2x + 3 = 7 và y² + 1 = 1
*) 2x + 3 = 7
2x = 4
x = 2 (nhận)
*) y² + 1 = 1
y² = 0
y = 0 (nhận)
TH4: 2x + 3 = -7 và y² + 1 = -1
*) 2x + 3 = -7
2x = -10
x = -5 (nhận)
*) y² + 1 = -1
y² = -2 (vô lý)
Vậy ta được cặp giá trị (x; y) thỏa mãn: (2; 0)

\(P=\dfrac{x^4+5x^3-20x^2-27x+30}{x^2+4x-21}\left(1\right)\)
Điều kiện xác định khi và chỉ khi
\(x^2+4x-21\ne0\)
\(\Leftrightarrow x^2+7x-3x-21\ne0\)
\(\Leftrightarrow x\left(x+7\right)-3\left(x+7\right)\ne0\)
\(\Leftrightarrow\left(x-3\right)\left(x+7\right)\ne0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ne3\\x\ne-7\end{matrix}\right.\)
Theo đề bài : \(\)
\(x=\sqrt[]{31-12\sqrt[]{3}}=\sqrt[]{27-12\sqrt[]{3}+4}=\sqrt[]{\left(3\sqrt[]{3}-2\right)^2}=\left|3\sqrt[]{3}-2\right|=3\sqrt[]{3}-2\)
\(\left(1\right)\Leftrightarrow P=\dfrac{x^4-3x^3+8x^3-24x^2+4x^2-12x-15x+45-15}{\left(x-3\right)\left(x+7\right)}\)
\(\Leftrightarrow P=\dfrac{x^3\left(x-3\right)+8x^2\left(x-3\right)+4x\left(x-3\right)-15\left(x-3\right)-15}{\left(x-3\right)\left(x+7\right)}\)
\(\Leftrightarrow P=\dfrac{\left(x-3\right)\left(x^3+8x^2+4x-15\right)-15}{\left(x-3\right)\left(x+7\right)}\)
\(\Leftrightarrow P=\dfrac{x^3+8x^2+4x-15}{x+7}-\dfrac{15}{\left(x-3\right)\left(x+7\right)}\)
\(\Leftrightarrow P=\dfrac{x^3+7x^2+x^2+7x-3x-15}{x+7}-\dfrac{15}{\left(x-3\right)\left(x+7\right)}\)
\(\Leftrightarrow P=\dfrac{x^2\left(x+7\right)+x\left(x+7\right)-3\left(x+7\right)+6}{x+7}-\dfrac{15}{\left(x-3\right)\left(x+7\right)}\)
\(\Leftrightarrow P=\dfrac{\left(x^2+x-3\right)\left(x+7\right)+6}{x+7}-\dfrac{15}{\left(x-3\right)\left(x+7\right)}\)
\(\Leftrightarrow P=x^2+x-3+\dfrac{6}{x+7}-\dfrac{15}{\left(x-3\right)\left(x+7\right)}\)
Thay \(x=3\sqrt[]{3}-2\) vào \(P\) ta được
\(\Leftrightarrow P=\left(3\sqrt[]{3}-2\right)^2+3\sqrt[]{3}-2-3+\dfrac{6}{3\sqrt[]{3}-2+7}-\dfrac{15}{\left(3\sqrt[]{3}-2-3\right)\left(3\sqrt[]{3}-2+7\right)}\)
\(\Leftrightarrow P=31-12\sqrt[]{3}+3\sqrt[]{3}-5+\dfrac{6}{3\sqrt[]{3}+5}-\dfrac{15}{\left(3\sqrt[]{3}-5\right)\left(3\sqrt[]{3}+5\right)}\)
\(\Leftrightarrow P=26-9\sqrt[]{3}+\dfrac{6\left(3\sqrt[]{3}-5\right)}{\left(3\sqrt[]{3}+5\right)\left(3\sqrt[]{3}-5\right)}-\dfrac{15}{\left(3\sqrt[]{3}\right)^2-5^2}\)
\(\Leftrightarrow P=26-9\sqrt[]{3}+\dfrac{6\left(3\sqrt[]{3}-5\right)}{2}-\dfrac{15}{2}\)
\(\Leftrightarrow P=\dfrac{37}{2}-9\sqrt[]{3}+3\left(3\sqrt[]{3}-5\right)\)
\(\Leftrightarrow P=\dfrac{37}{2}-9\sqrt[]{3}+9\sqrt[]{3}-15\)
\(\Leftrightarrow P=\dfrac{37}{2}-15=\dfrac{7}{2}\)
 giúp mik với
giúp mik với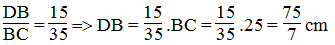
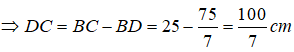
Bạn xem lại đề bài.
Bạn xem lại đề