\(\dfrac{x^2+5}{3x^2-6x+3}\).\(\dfrac{7x+35}{6x-6}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


?????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????????

Áp dụng định lý Bơ-du ta có:
f(x) ⋮ (2x+5) ⇔ f(\(\dfrac{-5}{2}\))=0 (Kiến thức nâng cao lớp 8)
⇔ (\(\dfrac{-5}{2}\))4+(\(\dfrac{-5}{2}\))3+12.(\(\dfrac{-5}{2}\))2-m=0
⇔ \(\dfrac{1575}{16}\)-m=0
⇔ m= \(\dfrac{1575}{16}\)
Vậy m=\(\dfrac{1575}{16}\) thì x4+x3+12x2-m chia hết cho 2x+5

\(B=\dfrac{x^2-x+1+4}{x^2-x+1}=1+\dfrac{4}{x^2-x+1}=1+\dfrac{4}{\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}}\)
Do \(\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4};\forall x\)
\(\Rightarrow B\le1+\dfrac{4}{\dfrac{3}{4}}=\dfrac{19}{3}\)
Vậy \(B_{max}=\dfrac{19}{3}\) khi \(x-\dfrac{1}{2}=0\Rightarrow x=\dfrac{1}{2}\)
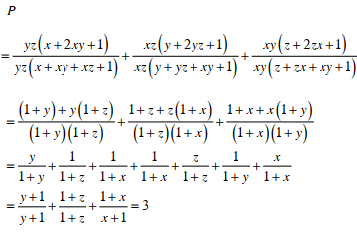
\(đk:x\ne1\)
\(\dfrac{x^2+5}{3x^2-6x+3}.\dfrac{7x+35}{6x-6}\\ =\dfrac{x^2+5}{3\left(x^2-2x+1\right)}.\dfrac{7\left(x+5\right)}{6\left(x-1\right)}\\ =\dfrac{x^2+5}{3\left(x-1\right)^2}.\dfrac{7\left(x+5\right)}{6\left(x-1\right)}\\ =\dfrac{7\left(x^2+5\right)\left(x+5\right)}{18.\left(x-1\right)^3}\)