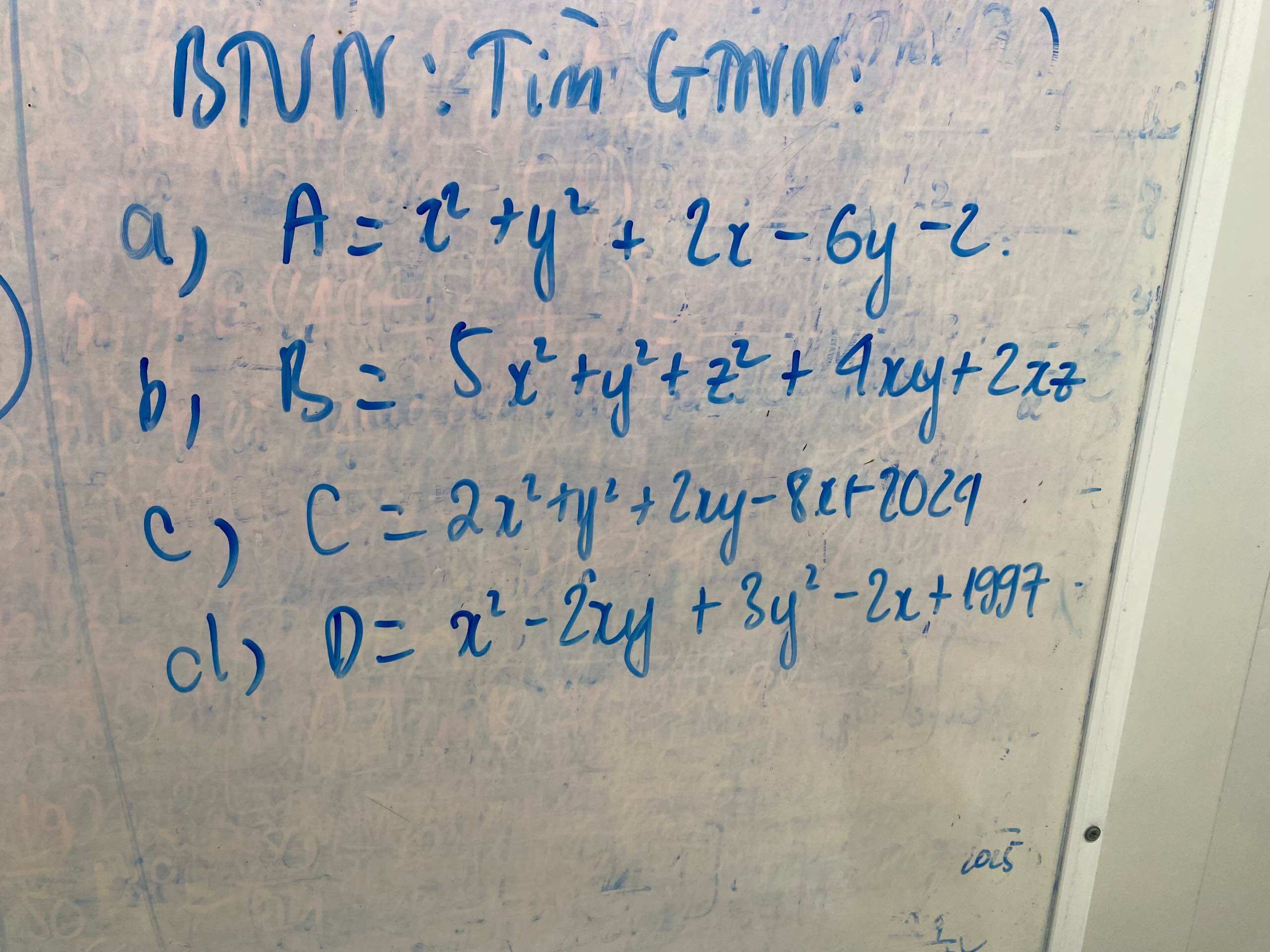1)

Gọi CTHH của X là \(Al_xCl_y\)

Theo đề có: 

\(\%m_{Al}=\dfrac{27x.100\%}{133,5}=20,225\%\Rightarrow x=1\)

\(\Rightarrow y=\dfrac{133,5-27.1}{35,5}=3\)

=> CTHH X: \(AlCl_3\)

2)

\(n_{CO_2}=\dfrac{336:1000}{24,79}=0,014\left(mol\right)\)

\(C+O_2\underrightarrow{t^o}CO_2\)

\(m_{O_2}=0,014.32=0,434\left(g\right)\\ m_C=0,014.12=0,168\left(g\right)\)

CHỊU

Xét tứ giác MNPQ ta có:

\(\widehat{M}+\widehat{N}+\widehat{P}+\widehat{Q}=360^o\) (tổng các góc trong tam giác)\

\(\widehat{M}:\widehat{N}:\widehat{P}:\widehat{Q}=1:2:3:4\\ =>\dfrac{\widehat{M}}{1}=\dfrac{\widehat{N}}{2}=\dfrac{\widehat{P}}{3}=\dfrac{\widehat{Q}}{4}\)

Áp dụng tính chất dãy tỉ số bằng nhau ta có:

\(\dfrac{\widehat{M}}{1}=\dfrac{\widehat{N}}{2}=\dfrac{\widehat{P}}{3}=\dfrac{\widehat{Q}}{4}=\dfrac{\widehat{M}+\widehat{N}+\widehat{Q}+\widehat{Q}}{1+2+3+4}=\dfrac{360^o}{10}=36^o\\ =>\widehat{M}=36^o\\ =>\dfrac{\widehat{N}}{2}=36^o=>\widehat{N}=72^o\\ =>\dfrac{\widehat{P}}{3}=36^o=>\widehat{P}=108^o\\ =>\dfrac{\widehat{Q}}{4}=36^o=>\widehat{Q}=144^o\) 

Vì: \(\widehat{M}+\widehat{Q}=36^o+144^o=180^o\) => MN//PQ => MNPQ là hình thang 

\(a,A=x^2+y^2+2x-6y-2\\ =\left(x^2+2x+1\right)+\left(y^2-6y+9\right)-12\\ =\left(x+1\right)^2+\left(y-3\right)^2-12\)

Ta có:

`(x+1)^2>=0` với mọi x

`(y-3)^2>=0` với mọi y

`=>A=(x+1)^2+(y-3)^2-12>=-12` với mọi x,y

Dấu "=" xảy ra: `x+1=0` và `y-3=0`

`<=>x=-1` và `y=3` 

\(b,B=5x^2+y^2+z^2+4xy+2xz\\ =\left(4x^2+4xy+y^2\right)+\left(x^2+2xz+z^2\right)\\ =\left(2x+y\right)^2+\left(x+z\right)^2\)

Ta có:

`(2x+y)^2>=0` với mọi x,y

`(x+z)^2>=0` với mọi x,z 

`=>B=(2x+y)^2+(x+z)^2>=0` 

Dấu "=" xảy ra: `2x+y=0` và `x+z=0`

`<=>2x=-y=-2z` 

\(c,C=2x^2+y^2+2xy-8x+2024\\ =\left(x^2+2xy+y^2\right)+\left(x^2-8x+16\right)+2008\\ =\left(x+y\right)^2+\left(x-4\right)^2+2008\)

Ta có:

`(x+y)^2>=0` với mọi x,y

`(x-4)^2>=0` với mọi x

`=>C=(x+y)^2+(x-4)^2+2008>=2008` 

Dấu "=" xảy ra: 

`x+y=0` và `x-4=0`

`<=>x=4` và `y=-4`

\(d,D=x^2-2xy+3y^2-2x+1997\\ =\left(x^2+y^2+1-2xy-2x+2y\right)+\left(2y^2-2y+\dfrac{1}{2}\right)+\dfrac{3991}{2}\\ =\left(-x+y+1\right)^2+2\left(y^2-y+\dfrac{1}{4}\right)+\dfrac{3991}{2}\\ =\left(-x+y+1\right)^2+2\left(y-\dfrac{1}{2}\right)^2+\dfrac{3991}{2}\)

Ta có:

`(-x+y+1)^2>=0` với mọi x,y

`2(y-1/2)^2>=0` với mọi y 

`=>D=(-x+y+1)^2+2(y-1/2)^2+3991/2>=3991/2` 

Dấu "=" xảy ra: `-x+y+1=0` và `y-1/2=0`

`<=>y=1/2` và `x=3/2` 

ΔEHF vuông tại H

=>\(HE^2+HF^2=EF^2\)

=>\(HE=\sqrt{5^2-3^2}=4\left(cm\right)\)

Xét ΔHEG vuông tại H và ΔHFE vuông tại H có

\(\widehat{HEG}=\widehat{HFE}\left(=90^0-\widehat{G}\right)\)

Do đó: ΔHEG~ΔHFE

=>\(\dfrac{HE}{HF}=\dfrac{HG}{HE}\)

=>\(HE^2=HF\cdot HG\)

=>\(HG=\dfrac{4^2}{3}=\dfrac{16}{3}\left(cm\right)\)

ΔEHG vuông tại H

=>\(HE^2+HG^2=EG^2\)

=>\(EG=\sqrt{\left(\dfrac{16}{3}\right)^2+4^2}=\dfrac{8\sqrt{13}}{3}\left(cm\right)\)

a: Xét ΔAEH vuông tại E và ΔAHB vuông tại H có

\(\widehat{EAH}\) chung

Do đó: ΔAEH~ΔAHB

=>\(\dfrac{AE}{AH}=\dfrac{AH}{AB}\)

=>\(AH^2=AE\cdot AB\left(1\right)\)

Xét ΔAFH vuông tại F và ΔAHC vuông tại H có

\(\widehat{FAH}\) chung

Do đó: ΔAFH~ΔAHC

=>\(\dfrac{AF}{AH}=\dfrac{AH}{AC}\)

=>\(AH^2=AF\cdot AC\left(2\right)\)

Từ (1),(2) suy ra \(AE\cdot AB=AF\cdot AC\)

b: Xét tứ giác AEHF có \(\widehat{AEH}=\widehat{AFH}=\widehat{FAE}=90^0\)

nên AEHF là hình chữ nhật

=>\(HA^2=HE^2+HF^2\)

Xét ΔEHA vuông tại H và ΔEBH vuông tại E có

\(\widehat{EHA}=\widehat{EBH}\left(=90^0-\widehat{HAE}\right)\)

Do đó: ΔEHA~ΔEBH

=>\(\dfrac{EH}{EB}=\dfrac{EA}{EH}\)

=>\(EH^2=EA\cdot EB\)

Xét ΔFHA vuông tại F và ΔFCH vuông tại F có

\(\widehat{FHA}=\widehat{FCH}\left(=90^0-\widehat{HAC}\right)\)

Do đó: ΔFHA~ΔFCH

=>\(\dfrac{FH}{FC}=\dfrac{FA}{FH}\)

=>\(FH^2=FA\cdot FC\)

\(HA^2=HE^2+HF^2=EA\cdot EB+FA\cdot FC\)

bài này ngữ pháp là gì

That's the easiest question to answer 

This textbook is easy to understand

________, I decided to stop trading with them

A.Despite of the fact that they were the biggest dealer

B.Though being the biggest dealer

C.Being the biggest dealer

D.Even though they were the biggest dealer