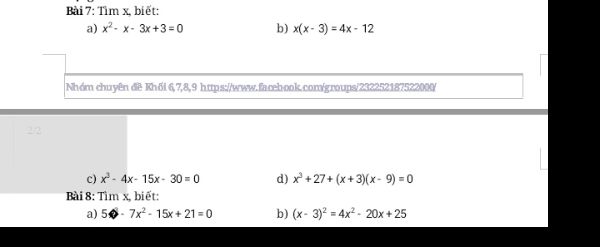
Phân tích đa thức : 2x^3 +16
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


12x-5)(4x-1)+(3x-7)(1-16x)=81
⇒48x2−32x+5−48x2+115x−7=81
⇒48x2−32x+5−48x2+115x−7=81
⇒83x=83
⇒x=1
(12x-5)(4x-1)+(3x-7)(1-16x)=81
12x ( 4x- 1) - 5(4x-1) + 3x(1-16x) -7(1-16x) =81
12x . 4x -12x - 20x + 5 + 3x - 3x. 16x - 7 + 7.16x = 81
48x^2 -12x - 20x + 5 + 3x - 48x^2 - 7 + 112x = 81
(48x^2 - 48x^2) + (112x - 12x - 20x +3x) - ( 7 - 5) =81
83x = 81 + 2
83x =83
x=1

Phân tích thành nhân tử:
x^2 - y^2 - 4x + 4
=(x^2-4x+4)-y^2
=(x-2)^2-y^2
=(x-2+y)(x-2-y)



a) \(f\left(x\right)=2x^3+9x^2-9x+m\)
Để \(f\left(x\right)\)chia hết cho \(2x-1\)thì \(f\left(x\right)=\left(2x-1\right)q\left(x\right)\)(với \(q\left(x\right)\)là đa thức thương)
Khi đó \(f\left(\frac{1}{2}\right)=0\)
Từ đây suy ra \(m=2\).
b) \(f\left(x\right)=2x^4-8x^3+5x^2+mx+n\)
Để \(f\left(x\right)\)chia hết cho \(2x^2-1\)thì \(f\left(x\right)=\left(2x^2-1\right)q\left(x\right)\)(với \(q\left(x\right)\)là đa thức thương)
Có \(2x^2-1=0\Leftrightarrow x=\pm\sqrt{\frac{1}{2}}\).
Suy ra \(f\left(\sqrt{\frac{1}{2}}\right)=f\left(-\sqrt{\frac{1}{2}}\right)=0\)
Từ đây suy ra \(\hept{\begin{cases}\sqrt{\frac{1}{2}}m+n=3-2\sqrt{2}\\-\sqrt{\frac{1}{2}}m+n=3+2\sqrt{2}\end{cases}}\Leftrightarrow\hept{\begin{cases}m=-4\\n=3\end{cases}}\).

\(2x^3+16\)
\(=2\left(x^3+8\right)\)
\(=2\left(x+2\right)\left(x^2-2x+4\right)\)