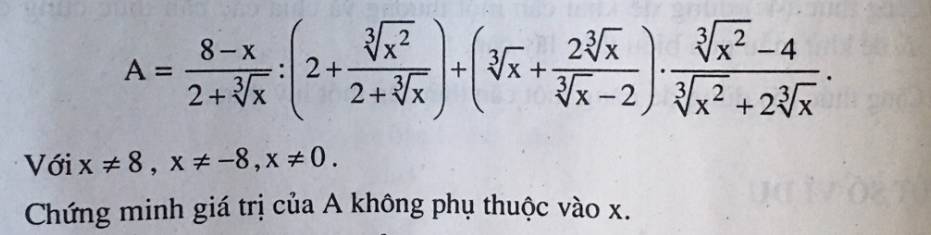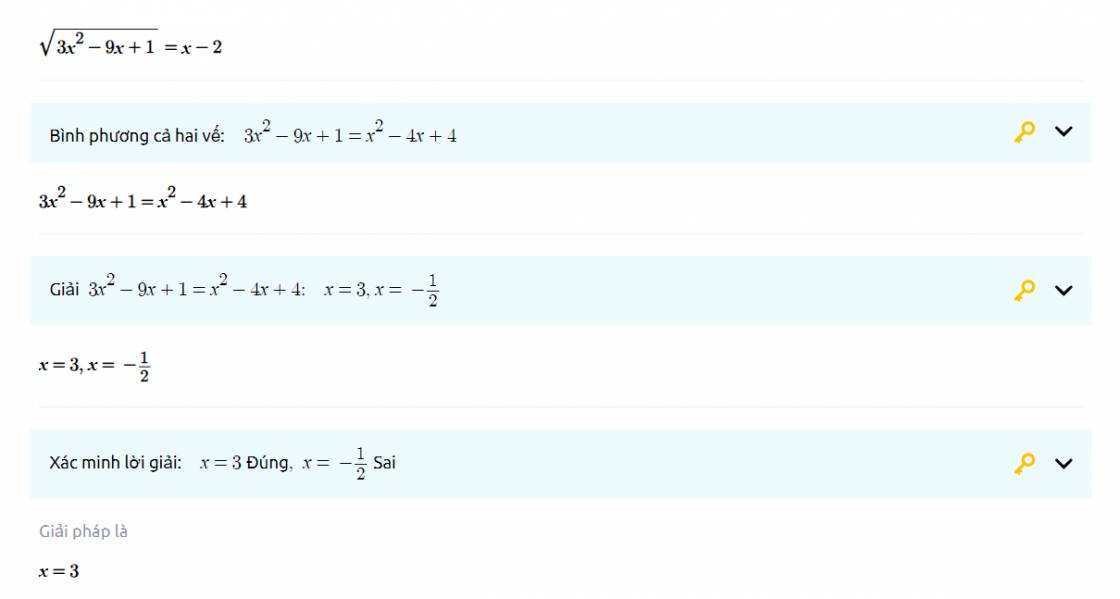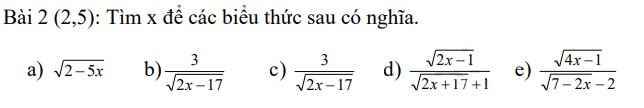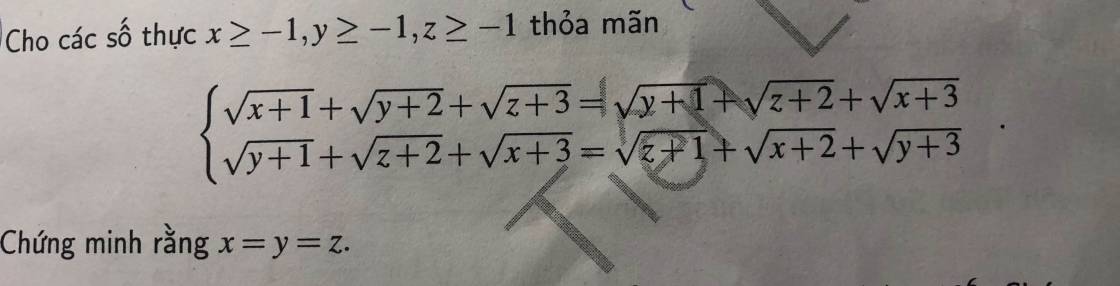
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\sqrt{2-\sqrt{3}}\)
= \(\dfrac{\sqrt{2}.\sqrt{2-\sqrt{3}}}{\sqrt{2}}\)
= \(\dfrac{\sqrt{4-2\sqrt{3}}}{\sqrt{2}}\)
= \(\dfrac{\sqrt{3-2\sqrt{3}+1}}{\sqrt{2}}\)
= \(\dfrac{\sqrt{\left(\sqrt{3}-1\right)^2}}{\sqrt{2}}\)
= \(\dfrac{|\sqrt{3}-1|}{\sqrt{2}}\)
= \(\dfrac{\sqrt{3}-1}{\sqrt{2}}\)
= \(\dfrac{\sqrt{2}.\left(\sqrt{3}-1\right)}{2}\)
= \(\dfrac{\sqrt{6}-\sqrt{2}}{2}\)


Bài này chỉ tìm được GTLN thôi nhé bạn.
Ta thấy \(A=-\dfrac{1}{3}x^2+2x\)
\(A=-\dfrac{1}{3}\left(x^2-6x\right)\)
\(A=-\dfrac{1}{3}\left(x^2-6x+9\right)+3\)
\(A=-\dfrac{1}{3}\left(x-3\right)^2+3\)
Vì \(\left(x-3\right)^2\ge0\) nên \(A\le3\) (dấu "=" xảy ra khi \(x-3=0\Leftrightarrow x=3\)). Như vậy GTLN của A là 3, đạt được khi \(x=3\).

\(\sqrt{x^2-6x+9}+x=11\); (ĐKXĐ\(\forall x\in R\))
<=> \(\sqrt{x^2-6x+9}=11-x\)
<=> \(\sqrt{\left(x-3\right)^2}=11-x\)
<=> \(|x-3|=11-x\)
<=> \(\left[{}\begin{matrix}x-3=11-x\\x-3=-11+x\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}2x=14\\0x=-8\left(vô\right)lí\left(\right)\end{matrix}\right.\)
<=> x=7 (thỏa mãn ĐKXĐ)
Vậy phương trình có nghiệm là x=7

Bạn sửa lại đề bài nhé. Tam giác ABC vuông tại C (nghĩa là \(\widehat{C}=90^o\)) thì \(\tan C\) làm sao bằng 0,5 được vậy bạn? (thực ra \(\tan C\) thậm chí còn không xác định nữa)

Từ B dựng đường thẳng vuông góc với AC cắt AC tại H
Xét tg vuông ABH có
\(\widehat{ABH}=90^o-\widehat{A}=90^o-60^o=30^o\)
\(\Rightarrow AH=\dfrac{AB}{2}=\dfrac{3}{2}=1,5cm\) (trong tg vuông cạnh đối diện góc 30 độ bằng nửa cạnh huyền)
\(\Rightarrow BH=\sqrt{AB^2-AH^2}=\sqrt{3^2-1,5^2}\)
Xét tg vuông BCH
\(\widehat{ACB}=30^o\)
=> \(BH=\dfrac{BC}{2}\Rightarrow BC=2.BH\) (lý do như trên)
Bạn tự thay số và tính nốt nhé
Xin lỗi mình nhầm từ chô \(\widehat{ACB}=30^o\)
Ta có
\(CH=AC-AH\)
Xét tg vuông BCH
\(BC=\sqrt{BH^2+CH^2}\)

\(\sqrt{3x^2-9x+1}=x-2\) (ĐK: \(x>2\) )
\(\Leftrightarrow3x^2-9x+1=\left(x-2\right)^2\)
\(\Leftrightarrow3x^2-9x+1=x^2-4x+4\)
\(\Leftrightarrow3x^2-9x+1-x^2+4x-4=0\)
\(\Leftrightarrow2x^2-5x-3=0\)
\(\Rightarrow\Delta=\left(-5\right)^2-4\cdot2\cdot\left(-3\right)=49>0\)
Vậy pt có 2 nghiệm:
\(\left\{{}\begin{matrix}x_1=\dfrac{-\left(-5\right)+\sqrt{49}}{2\cdot2}=3\\x_2=\dfrac{-\left(-5\right)-\sqrt{49}}{2\cdot2}=-\dfrac{1}{2}\left(ktm\right)\end{matrix}\right.\)
Vậy: \(S=\left\{3\right\}\)

\(e,\dfrac{\sqrt{4x-1}}{\sqrt{7-2x}-2}\) có nghĩa \(\Leftrightarrow\left[{}\begin{matrix}4x-1\ge0\\7-2x\ne4\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x\ge\dfrac{1}{4}\\x\ne-\dfrac{3}{2}\end{matrix}\right.\) \(\Leftrightarrow x\ge\dfrac{1}{4}\)
\(d,\dfrac{\sqrt{2x-1}}{\sqrt{2x+17}+1}\) có nghĩa \(\Leftrightarrow\left[{}\begin{matrix}2x-1\ge0\\2x+17\ge0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x\ge\dfrac{1}{2}\\x\ge-\dfrac{17}{2}\end{matrix}\right.\) \(\Leftrightarrow x\ge\dfrac{1}{2}\)
\(b,c,\dfrac{3}{\sqrt{2x-17}}\) có nghĩa \(\Leftrightarrow2x-17>0\Leftrightarrow x>\dfrac{17}{2}\)
\(a,\sqrt{2-5x}\) có nghĩa \(\Leftrightarrow2-5x\ge0\Leftrightarrow x\le\dfrac{2}{5}\)