NQ
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
21 tháng 8 2021
Ta có: \(A=2\left(x+y\right)^4-3\left(x+y\right)^2-5\)
\(=2\left(x+y\right)^4-5\left(x+y\right)^2+2\left(x+y\right)^2-5\)
\(=\left(x+y\right)^2\left[2\left(x+y\right)^2-5\right]+\left[2\left(x+y\right)^2-5\right]\)
\(=\left[2\left(x+y\right)^2-5\right]\left[\left(x+y\right)^2+1\right]\)
12 tháng 10 2021
\(1,\\ a,=4\left(x-2\right)^2+y\left(x-2\right)=\left(4x-8+y\right)\left(x-2\right)\\ b,=3a^2\left(x-y\right)+ab\left(x-y\right)=a\left(3a+b\right)\left(x-y\right)\\ 2,\\ a,=\left(x-y\right)\left[x\left(x-y\right)^2-y-y^2\right]\\ =\left(x-y\right)\left(x^3-2x^2y+xy^2-y-y^2\right)\\ b,=2ax^2\left(x+3\right)+6a\left(x+3\right)\\ =2a\left(x^2+3\right)\left(x+3\right)\\ 3,\\ a,=xy\left(x-y\right)-3\left(x-y\right)=\left(xy-3\right)\left(x-y\right)\\ b,Sửa:3ax^2+3bx^2+ax+bx+5a+5b\\ =3x^2\left(a+b\right)+x\left(a+b\right)+5\left(a+b\right)\\ =\left(3x^2+x+5\right)\left(a+b\right)\\ 4,\\ A=\left(b+3\right)\left(a-b\right)\\ A=\left(1997+3\right)\left(2003-1997\right)=2000\cdot6=12000\\ 5,\\ a,\Leftrightarrow\left(x-2017\right)\left(8x-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2017\\x=\dfrac{1}{4}\end{matrix}\right.\\ b,\Leftrightarrow\left(x-1\right)\left(x^2-16\right)=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=4\\x=-4\end{matrix}\right.\)
11 tháng 11 2018
xin loi bn nha
mk moi chi hok lop 6
mk nghi bn nen tu lam thi hon,ko nen dua vao olm.vn qua
chuc bn hok tot nha
PN
2
KT
14 tháng 12 2017
x3 - 5x2 + x - 5 = (x3 - 5x2) + (x - 5) = x2(x - 5) + (x - 5) = (x - 5)(x2 + 1)
14 tháng 12 2017
cho mk hỏi rút gọn bt nay nhé A= 1phần x+1 trừ x-1phân x cộng x+2 phần x^2 +x
26 tháng 10 2016
x^8+x+1= (x^8 - x^5) + (x^5 - x^2) + (x^2+x+1)
= x^5.(x^3-1) + x^2.(x^3-1) + (x^2+x+1)
= x^5.(x-1).(x^2+x+1) + x^2.(x-1).(x^2+x+1) + (x^2+x+1)
=(x^2+x+1).[x^5.(x-1)+x^2.(x-1)+1]
KT
17 tháng 7 2018
d) mk chỉnh lại đề
\(8xy^2-5xyz-24y+15z\)
\(=xy\left(8y-5z\right)-3\left(8y-5z\right)\)
\(=\left(8y-5z\right)\left(xy-3\right)\)
e) \(x^4-x^3-x+1=\left(x-1\right)^2\left(x^2+x+1\right)\)
f) \(x^4+x^2y^2+y^4=\left(x^2-xy+y^2\right)\left(x^2+xy-y^2\right)\)
g) \(x^3+3x-4=\left(x-1\right)\left(x^2+x+4\right)\)
h) \(x^3-3x^2+2=\left(x-1\right)\left(x^2-2x-2\right)\)
i) \(2x^3+x^2-4x-12=\left(x-2\right)\left(2x^2+5x+6\right)\)
k) \(25x^2\left(x-5\right)-x+y=\left(1-5x\right)\left(1+5x\right)\left(y-x\right)\)
24 tháng 9 2021
\(1,\\ 1,=15\left(x+y\right)\\ 2,=4\left(2x-3y\right)\\ 3,=x\left(y-1\right)\\ 4,=2x\left(2x-3\right)\\ 2,\\ 1,=\left(x+y\right)\left(2-5a\right)\\ 2,=\left(x-5\right)\left(a^2-3\right)\\ 3,=\left(a-b\right)\left(4x+6xy\right)=2x\left(2+3y\right)\left(a-b\right)\\ 4,=\left(x-1\right)\left(3x+5\right)\\ 3,\\ A=13\left(87+12+1\right)=13\cdot100=1300\\ B=\left(x-3\right)\left(2x+y\right)=\left(13-3\right)\left(26+4\right)=10\cdot30=300\\ 4,\\ 1,\Rightarrow\left(x-5\right)\left(x-2\right)=0\Rightarrow\left[{}\begin{matrix}x=2\\x=5\end{matrix}\right.\\ 2,\Rightarrow\left(x-7\right)\left(x+2\right)=0\Rightarrow\left[{}\begin{matrix}x=7\\x=-2\end{matrix}\right.\\ 3,\Rightarrow\left(3x-1\right)\left(x-4\right)=0\Rightarrow\left[{}\begin{matrix}x=\dfrac{1}{3}\\x=4\end{matrix}\right.\\ 4,\Rightarrow\left(2x+3\right)\left(2x-1\right)=0\\ \Rightarrow\left[{}\begin{matrix}x=-\dfrac{3}{2}\\x=\dfrac{1}{2}\end{matrix}\right.\)
TN
1
27 tháng 7 2019
21(x-y)2-7(y-x)3
=21(x2-y2)-7(y2-x2)
=21x2-21y2-7y2+7x2
=28x2-28y2
=28(x2-y2)
T
13 tháng 8 2023
1)
a) => 16x2 - 8x + 1 - 8(2x2 + 3x - 4x - 6) = 15
=> 16x2 - 8x + 1 - 8(2x2 - x - 6) = 15
=> 16x2 - 8x + 1 - 16x2 + 8x + 48 = 15
=> 49 = 15 (?) (vô lí)
=> Không tìm được x thoả mãn
b) (5x - 2)(x - 2) - 4(x - 3) = x2 + 3
=> 5x2 - 10x - 2x + 4 - 4x + 12 = x2 + 3
=> 5x2 - 16x + 16 = x2 + 3
=> 4x2 - 16x + 16 = 3
=> (2x)2 - 2.2x.4 + 42 = 3
=> (2x - 4)2 = 3
=> \(\left[{}\begin{matrix}2x-4=\sqrt{3}\\2x-4=-\sqrt{3}\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\dfrac{4+\sqrt{3}}{2}\\x=\dfrac{4-\sqrt{3}}{2}\end{matrix}\right.\)
Mong bạn xem lại đề bài!
T
13 tháng 8 2023
2)
a) 5x2 - 10xy + 5y2 - 20z2
= 5(x2 - 2xy + y2 - 4z2)
= 5[(x - y)2 - (2z)2]
= 5(x - y - 2z)(x - y + 2z)
b) a3 - ay - a2x + xy
= a(a2 - y) - x(a2 - y)
= (a - x)(a2 - y)
c) 3x2 - 6xy + 3y2 - 12z2
= 3(x2 - 2xy + y2 - 4z2)
= 3[(x - y)2 - (2z)2]
= 3(x - y - 2z)(x - y + 2z)
d) x2 - 2xy + tx - 2ty
= x(x - 2y) + t(x - 2y)
= (x + t)(x - 2y)
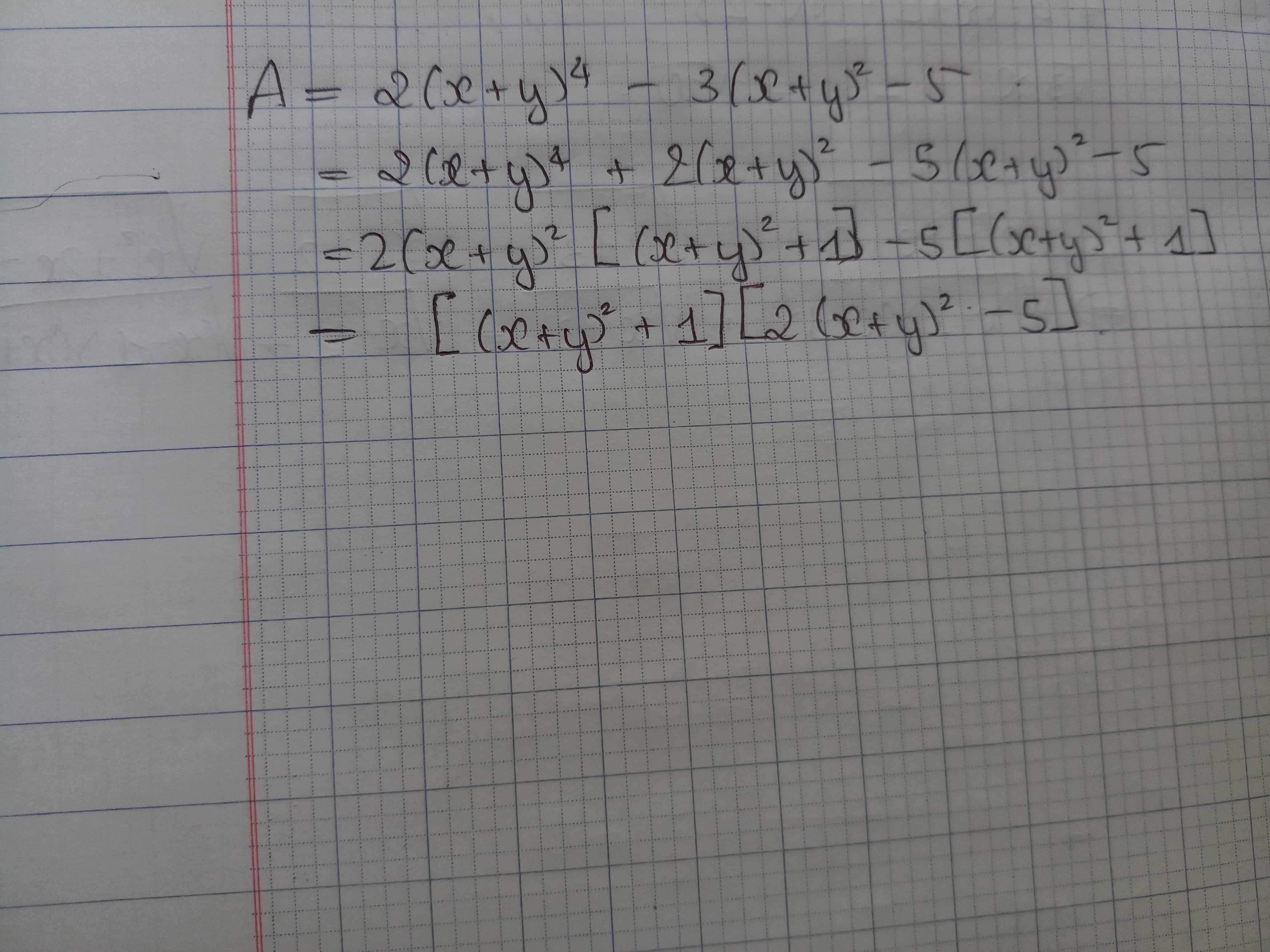
(x-1)(x-3)(x+2)(x+4)+21
\(=\left(x-1\right)\left(x+2\right)\left(x-3\right)\left(x+4\right)+21\)
\(=\left(x^2+x-2\right)\left(x^2+x-12\right)+21\)
\(=\left(x^2+x\right)^2-14\left(x^2+x\right)+24+21\)
\(=\left(x^2+x\right)^2-14\left(x^2+x\right)+45\)
\(=\left(x^2+x-5\right)\left(x^2+x-9\right)\)
Chúng ta có biểu thức:
(x−1)(x−3)(x+2)(x+4)+21(x-1)(x-3)(x+2)(x+4) + 21Bước 1: Tính (x−1)(x−3)=x2−4x+3(x-1)(x-3) = x^2 - 4x + 3 và (x+2)(x+4)=x2+6x+8(x+2)(x+4) = x^2 + 6x + 8.
Bước 2: Nhân hai kết quả lại:
(x2−4x+3)(x2+6x+8)=x4+2x3−13x2−14x+24(x^2 - 4x + 3)(x^2 + 6x + 8) = x^4 + 2x^3 - 13x^2 - 14x + 24Bước 3: Thêm 21 vào:
x4+2x3−13x2−14x+24+21=x4+2x3−13x2−14x+45x^4 + 2x^3 - 13x^2 - 14x + 24 + 21 = x^4 + 2x^3 - 13x^2 - 14x + 45Vậy, biểu thức đã phân tích là:
(x−1)(x−3)(x+2)(x+4)+21=x4+2x3−13x2−14x+45