Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

2) Phương trình hoành độ giao điểm là:
3x+m=2x-1
\(\Leftrightarrow3x-2x=-1-m\)
\(\Leftrightarrow x=-m-1\)
Để (*) cắt đồ thị của hàm số y=2x-1 tại điểm nằm trên góc vuông phần tư thứ IV thì \(\left\{{}\begin{matrix}x>0\\y< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-m-1>0\\2x-1< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-m>1\\2\left(-m-1\right)-1< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< -1\\-2m-2-1< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< -1\\-2m< 3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< -1\\m>\dfrac{-3}{2}\end{matrix}\right.\Leftrightarrow-\dfrac{3}{2}< m< -1\)

a: Thay x=1 và y=0 vào (d), ta được:
1-2m+3=0
\(\Leftrightarrow m=2\)

a) \(y=\left(m-1\right)x-3\left(1\right)\)
\(A\left(2;1\right)\in\left(1\right)\Leftrightarrow\left(m-1\right).2-3=1\)
\(\Leftrightarrow2m-2-3=1\)
\(\Leftrightarrow2m=6\)
\(\Leftrightarrow m=3\)
\(\Rightarrow y=2x-3\)
b) Để \(\left(1\right)\) đồng biến
\(\Leftrightarrow m-1>0\)
\(\Leftrightarrow m>1\)
c) \(\left(1\right)\cap\left(Ox\right)=\left(2;0\right)\)
\(\Leftrightarrow\left(m-1\right).2-3=0\)
\(\Leftrightarrow2m-5=0\)
\(\Leftrightarrow m=\dfrac{5}{2}\)
d) \(\left(1\right)\cap\left(Oy\right)=\left(0;1\right)\)
\(\Leftrightarrow\left(m-1\right).0-3=1\)
\(\Leftrightarrow0m=4\left(vô.lý\right)\)
Vậy không có giá trị m nào thỏa mãn đề bài

\(a,\Leftrightarrow1+m=-2\Leftrightarrow m=-3\\ \Leftrightarrow y=x-3\\ \text{Thay }x=2;y=5\Leftrightarrow5=2-3=-1\left(\text{vô lí}\right)\\ \Leftrightarrow E\notinđths\\ b,\text{PT giao Ox và Oy: }\left\{{}\begin{matrix}y=0\Rightarrow x=-m\Rightarrow E\left(-m;0\right)\Rightarrow OE=\left|m\right|\\x=0\Rightarrow y=m\Rightarrow F\left(0;m\right)\Rightarrow OF=\left|m\right|\end{matrix}\right.\)
Gọi H là chân đường cao từ O đến EF
Áp dụng HTL: \(\dfrac{1}{OH^2}=\dfrac{1}{OE^2}+\dfrac{1}{OF^2}=\dfrac{1}{2m^2}=\dfrac{1}{3^2}=\dfrac{1}{9}\)
\(\Leftrightarrow m^2=\dfrac{9}{2}\Leftrightarrow\left[{}\begin{matrix}m=\dfrac{3}{\sqrt{2}}\\m=-\dfrac{3}{\sqrt{2}}\end{matrix}\right.\)
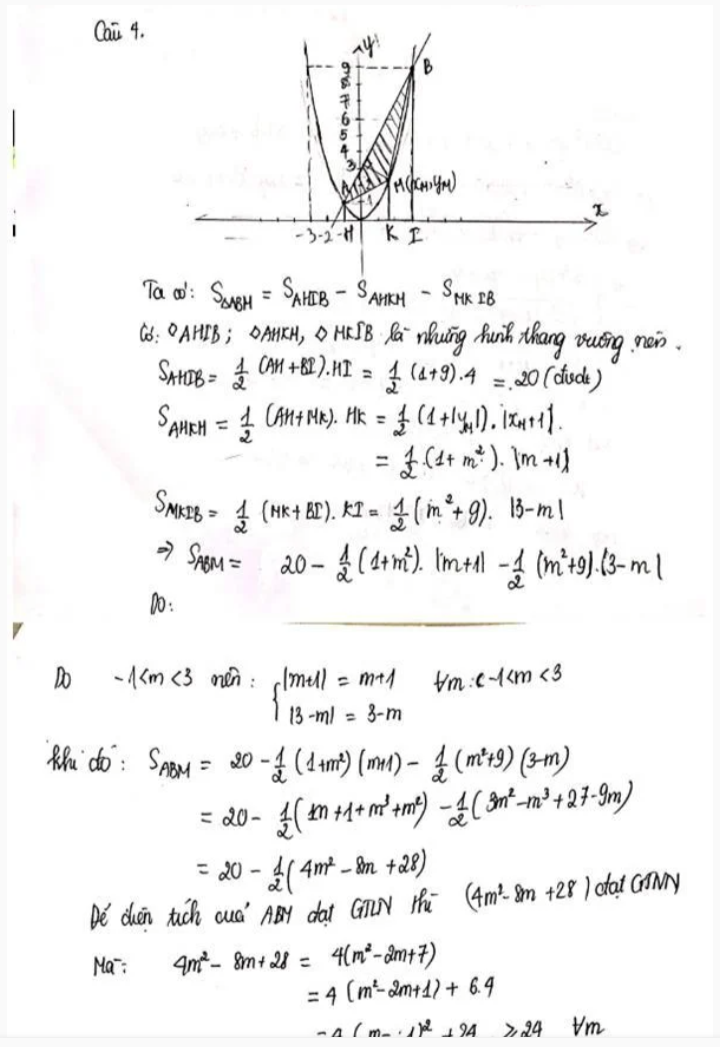

1)
aThay x=-1;y=3 vào đồ thị hàm số(*) ta được:
\(3=\left(m+2\right).\left(-1\right)^2\)
\(\Leftrightarrow m+2=3\)
\(\Leftrightarrow m=1\)
b)Thay x=\(\sqrt{2}\);y=-1 vào đồ thị hàm số (*) ta được:
\(-1=\left(m+2\right).\left(\sqrt{2}\right)^2\)
\(\Leftrightarrow2.\left(m+2\right)=-1\)
\(\Leftrightarrow2m+4=-1\)
\(\Leftrightarrow2m=-5\)
\(\Leftrightarrow m=-\frac{5}{2}\)
2)
Thay m=0 vào đồ thị hàm số (*) ta đươc: \(y=2x^2\)
Hoành độ giao điểm của đồ thị hàm số \(y=2x^2\)và đồ thị hàm số \(y=x+1\)là:
\(2x^2=x+1\)
\(\Leftrightarrow2x^2-x-1=0\)
\(\Leftrightarrow2x^2-2x+x-1=0\)
\(\Leftrightarrow2x\left(x-1\right)+\left(x-1\right)=0\)
\(\Leftrightarrow\left(2x+1\right)\left(x-1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}2x+1=0\\x-1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-\frac{1}{2}\\x=1\end{cases}}}\)
\(TH1:x=-\frac{1}{2}\)
Thay \(x=-\frac{1}{2}\)vào đồ thị hàm số \(y=x+1\)ta được:
\(y=-\frac{1}{2}+1=\frac{1}{2}\)
Ta được điểm A\(\left(-\frac{1}{2};\frac{1}{2}\right)\)
\(TH2:x=1\)
Thay \(x=1\)vào đồ thị hàm số \(y=x+1\)ta được:
\(y=1+1=2\)
Ta được điểm \(B\left(1;2\right)\)
1)
Vì đồ thị hàm số (*) đi qua điểm A(-1;3) nên x=-1 và y=3. Thay x=-1 và y=3 vào hàm số (*) ta được:
f(-1)=(m+2).(-1)2=3 <=> m+2=3 ⇔m=1
Vậy với m=1 thì đt hàm số đã cho đi qua điểm A(-1;3).
2) Thay m=0 vào hàm số (*)
ta có: y=f(x)=2x2
+) Phương trình hoành độ giao điểm của hàm số y=f(x)=2x2 và y=x+1 là:
2x2 = x+1
⇔2x2 -x-1=0
\(\left\{{}\begin{matrix}x_1=1\Rightarrow y_1=2\\x_2=\dfrac{-1}{2}\Rightarrow y_2=\dfrac{1}{2}\end{matrix}\right.\)
Vậy đường thẳng y=x+1 và y=f(x)=2x2 có giao điểm là M(1;2) và N(\(\dfrac{-1}{2};\dfrac{1}{2}\))