Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(L=\lim\limits_{x\rightarrow0}\frac{e^x-e^{-x}}{\sin x}=\lim\limits_{x\rightarrow0}\frac{e^x-\frac{1}{e^x}}{\sin x}=\lim\limits_{x\rightarrow0}\frac{e^{2x}-1}{e^x\sin x}=\lim\limits_{x\rightarrow0}\frac{e^{2x}-1}{2x.\frac{\sin x}{2x}.e^x}\)
\(=\lim\limits_{x\rightarrow0}\frac{e^{2x}-1}{2x}.\frac{1}{\frac{\sin x}{x}}.\frac{2}{e^x}=1.\frac{1}{1}.\frac{2}{1}=2\)

\(y=\log\left(\frac{1-\sqrt{x}}{2\sqrt{x}}\right)\Rightarrow y'=\frac{\left(\frac{1-\sqrt{x}}{2\sqrt{x}}\right)'}{\frac{1-\sqrt{x}}{x^2}\ln10}=\frac{-\frac{1}{2\sqrt{x}}.2\sqrt{x}-\frac{1}{\sqrt{x}}.\left(1-\sqrt{x}\right)}{\frac{1-\sqrt{x}}{2\sqrt{x}}\ln10}\)
\(=\frac{-1-\frac{1-\sqrt{x}}{\sqrt{x}}}{4x.\frac{1-\sqrt{x}}{2\sqrt{x}}\ln10}=\frac{1}{2x\left(\sqrt{x}-1\right)\ln10}\)

\(L=\lim\limits_{x\rightarrow0}\frac{e^x-1}{\sqrt{x+1}-1}=\lim\limits_{x\rightarrow0}\frac{\left(e^x-1\right)\left(\sqrt{x+1}-1\right)}{x}=\lim\limits_{x\rightarrow0}\left[\frac{e^x-1}{x}.\left(\sqrt{x+1}-1\right)\right]=1.0=0\)

a) Cách 1: Ta có:
y' = 6sin5x.cosx - 6cos5x.sinx + 6sinx.cos3x - 6sin3x.cosx = 6sin3x.cosx(sin2x - 1) + 6sinx.cos3x(1 - cos2x) = - 6sin3x.cos3x + 6sin3x.cos3x = 0.
Vậy y' = 0 với mọi x, tức là y' không phụ thuộc vào x.
Cách 2:
y = sin6x + cos6x + 3sin2x.cos2x(sin2x + cos2x) = sin6x + 3sin4x.cos2x + 3sin2x.cos4x + cos6x = (sin2x + cos2x)3 = 1
Do đó, y' = 0.
b) Cách 1:
Áp dụng công thức tính đạo hàm của hàm số hợp
(cos2u)' = 2cosu(-sinu).u' = -u'.sin2u
Ta được
y' =[sin - sin
] + [sin
- sin
] - 2sin2x = 2cos
.sin(-2x) + 2cos
.sin(-2x) - 2sin2x = sin2x + sin2x - 2sin2x = 0,
vì cos = cos
=
.
Vậy y' = 0 với mọi x, do đó y' không phụ thuộc vào x.
Cách 2: vì côsin của hai cung bù nhau thì đối nhau cho nên
cos2 = cos2
'
cos2 = cos2
.
Do đó
y = 2 cos2 + 2cos2
- 2sin2x = 1 +cos
+ 1 +cos
- (1 - cos2x) = 1 +cos
+ cos
+ cos2x = 1 + 2cos
.cos(-2x) + cos2x = 1 + 2
cos2x + cos2x = 1.
Do đó y' = 0.

\(y'=\frac{2x}{x^2+1}+\frac{2x-1}{\left(x^2-x+1\right)\ln2}\)

Đặt \(t=x-e\Rightarrow\begin{cases}x=t+e\\x\rightarrow e;t\rightarrow0\end{cases}\)
\(\Rightarrow L=\lim\limits_{t\rightarrow0}\frac{\ln\left(t+e\right)-\ln e}{t}=\lim\limits_{t\rightarrow0}\frac{\ln\left(\frac{t+e}{e}\right)}{t}=\lim\limits_{t\rightarrow0}\left[\frac{\ln\left(1+\frac{t}{e}\right)}{\frac{t}{e}}\right]=\frac{1}{e}\)
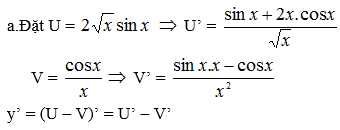
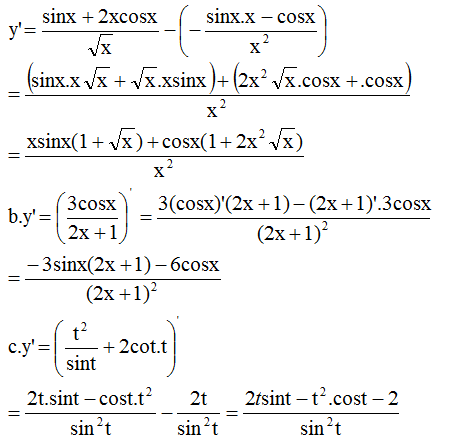
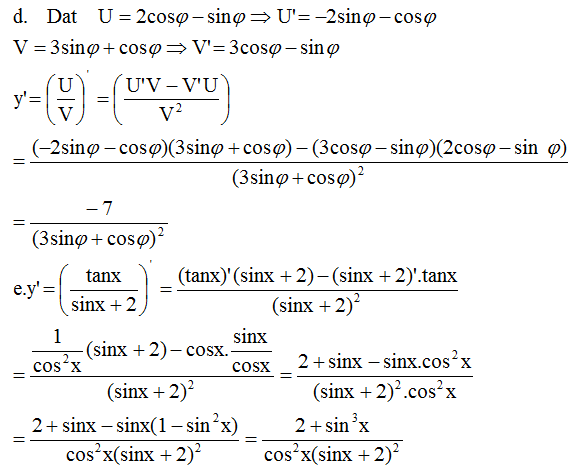
\(y'=\frac{e^x}{2\sqrt{e^x}}+3.e^{3x-1}-\left(-\sin x+\cos x\right)5^{\sin x+\cos x}\ln5\)
\(=\frac{\sqrt{e^x}}{2}+3e^{3x-1}+\left(\sin x+\cos x\right).5^{\sin x+\cos x}\ln5\)