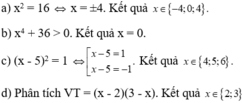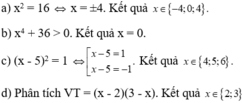
a)
x...">
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời. \(a,x^2-2x=0\) \(\Rightarrow x\left(x-2\right)=0\) \(\Rightarrow\left[{}\begin{matrix}x=0\\x-2=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=0\\x=-2\end{matrix}\right.\) Vậy ... \(b,\left(5-2x\right)^2-16=0\) \(\Rightarrow\left(5-2x\right)^2=16\) \(\Rightarrow\left(5-2x\right)^2=4^2\) \(\Rightarrow5-2x=\pm4\) \(\Rightarrow\left[{}\begin{matrix}5-2x=4\\5-2x=-4\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}2x=1\\2x=9\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=\dfrac{2}{9}\end{matrix}\right.\) Vậy ... \(c,x\left(x+3\right)-x^2-11=0\) \(\Rightarrow x^2+3x-x^2-11=0\) \(\Rightarrow3x-11=0\) \(\Rightarrow3x=11\) \(\Rightarrow x=\dfrac{11}{3}\) Vậy ... \(a.x^4-16x^2=0\Leftrightarrow\left(x^2+4x\right)\left(x^2-4x\right)=0\) \(\Leftrightarrow x^2\left(x+4\right)\left(x-4\right)=0\) \(\Leftrightarrow\left[{}\begin{matrix}x^2=0\\x+4=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-4\\x=4\end{matrix}\right.\) \(b.\left(x-5\right)^3-x+5=0\) \(\Leftrightarrow\left(x-5\right)^3-\left(x-5\right)=0\) \(\Leftrightarrow\left(x-5\right)\left[\left(x-5\right)^2-1\right]=0\) \(\Leftrightarrow\left[{}\begin{matrix}x-5=0\\\left(x-5\right)^2-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\\left(x-5\right)^2=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=6\end{matrix}\right.\) a) x4 - 16x2 = 0 <=> x2 ( x2 - 16 ) = 0 <=> \(\left[{}\begin{matrix}x^2=0\\x^2-16=0\end{matrix}\right.\) <=> \(\left[{}\begin{matrix}x=0\\x=-4\\x=4\end{matrix}\right.\) Vậy... b) ( x - 5)3 - x + 5 = 0 <=> ( x - 5)3 - (x - 5) = 0 <=> (x - 5) [ (x - 5)2 - 1] =0 <=> \(\left[{}\begin{matrix}x-5=0\\\left(x-5\right)^2-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\\left(x-5\right)^2=1\end{matrix}\right.\) <=> \(\left[{}\begin{matrix}x=5\\x-5=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=6\end{matrix}\right.\) Vậy... c) 5(x - 2) = x2 - 4 <=> 5(x - 2) - (x2 - 4) = 0 <=> (x - 2)( 5 - x - 2) = 0 <=> (x - 2)( 3 - x ) = 0 <=> \(\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\) Vậy... d) x - 3 = (3 - x)2 <=> x - 3 - (x - 3)2 = 0 <=> (x - 3)(1 - x + 3) = 0 <=> (x - 3)( 4 - x ) = 0 <=> \(\left[{}\begin{matrix}x=3\\x=4\end{matrix}\right.\) Vậy... e) x2 (x - 5) + 5 - x = 0 <=> x2 (x - 5) - (x - 5) = 0 <=> (x2 - 1)( x - 5) = 0 <=> \(\left[{}\begin{matrix}\left(x-1\right)\left(x+1\right)=0\\x-5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-1\\x=5\end{matrix}\right.\) , Bài 4 : Tìm x biết: a, 4x2 - 49 = 0 \(\Leftrightarrow\) (2x)2 - 72 = 0 \(\Leftrightarrow\) (2x - 7)(2x + 7) = 0 \(\Leftrightarrow\left\{{}\begin{matrix}2x-7=0\\2x+7=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{7}{2}\\x=-\dfrac{7}{2}\end{matrix}\right.\) b, x2 + 36 = 12x \(\Leftrightarrow\) x2 + 36 - 12x = 0 \(\Leftrightarrow\) x2 - 2.x.6 + 62 = 0 \(\Leftrightarrow\) (x - 6)2 = 0 \(\Leftrightarrow\) x = 6 e, (x - 2)2 - 16 = 0 \(\Leftrightarrow\) (x - 2)2 - 42 = 0 \(\Leftrightarrow\) (x - 2 - 4)(x - 2 + 4) = 0 \(\Leftrightarrow\) (x - 6)(x + 2) = 0 \(\Leftrightarrow\left\{{}\begin{matrix}x-6=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=6\\x=-2\end{matrix}\right.\) f, x2 - 5x -14 = 0 \(\Leftrightarrow\) x2 + 2x - 7x -14 = 0 \(\Leftrightarrow\) x(x + 2) - 7(x + 2) = 0 \(\Leftrightarrow\) (x + 2)(x - 7) = 0 \(\Leftrightarrow\left\{{}\begin{matrix}x+2=0\\x-7=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-2\\x=7\end{matrix}\right.\) a) \(\left(x+2\right)^2-9=0\) \(\Rightarrow\left(x+2\right)^2=9\) \(\Rightarrow\left(x+2\right)^2=3^2\) \(\Rightarrow x+2=3\) \(\Rightarrow x=3-2=1\) a) ( x + 2 )2 = 9 => ( x + 2 ) 2 = 9 => ( x + 2 )2 = 32 => x + 2 = + 3 => \(\orbr{\begin{cases}x+2=-3\\x+2=3\end{cases}}\) => \(\orbr{\begin{cases}x=-1\\x=5\end{cases}}\) Vậy x = -1; 5 b) ( x + 2 )2 - x2 + 4 = 0 => ( x + 2 )2 - ( x2 - 4 ) = 0 => ( x + 2 )2 - ( x + 2 ) ( x - 2 ) = 0 => ( x + 2 ) ( x + 2 - x + 2 ) = 0 => ( x + 2 ) . 4 = 0 => x + 2 = 0 => x = - 2 Vậy x = - 2 c) 5 ( 2x - 3 )2 - 5 ( x + 1 )2 - 15( x + 4 ) ( x - 4 ) = - 10 => 5 ( 4x2 - 12x + 9 ) - 5 ( x2 + 2x + 1 ) - 15 ( x2 - 42 ) = - 10 => 20x2 - 60x + 45 - 5x2 - 10x - 5 - 15x2 + 240 = -10 => - 70x + 280 = - 10 => - 70x = - 290 => x = \(\frac{29}{7}\) Vậy x = \(\frac{29}{7}\) d) x ( x + 5 ) ( x - 5 ) - ( x + 2 ) ( x2 - 2x + 4 ) = 3 => x ( x2 - 25 ) - ( x3 - 8 ) = 3 => x3 - 25x - x3 + 8 = 3 => - 25x + 8 = 3 => - 25x = -5 => x = \(\frac{1}{5}\) Vậy x = \(\frac{1}{5}\) b: =>(x+5)(x-3)=0 =>x=3 hoặc x=-5 c: \(\Leftrightarrow x\left(x^2-4x+5\right)=0\) =>x=0 d: \(\Leftrightarrow2\cdot2^x-10\cdot2^x=-16\) \(\Leftrightarrow-8\cdot2^x=-16\) \(\Leftrightarrow2^x=2\) hay x=1 a/ \(\left(x-4\right)^2-36=0\) <=> \(\left(x-4-6\right)\left(x-4+6\right)=0\) <=> \(\left(x-10\right)\left(x+2\right)=0\) <=> \(\orbr{\begin{cases}x-10=0\\x+2=0\end{cases}}\)<=> \(\orbr{\begin{cases}x=10\\x=-2\end{cases}}\) b/ \(\left(x+8\right)^2=121\) <=> \(\left(x+8\right)^2-121=0\) <=> \(\left(x+8-11\right)\left(x+8+11\right)=0\) <=> \(\left(x-3\right)\left(x+19\right)=0\) <=> \(\orbr{\begin{cases}x-3=0\\x+19=0\end{cases}}\)<=> \(\orbr{\begin{cases}x=3\\x=-19\end{cases}}\) d/ \(4x^2-12x+9=0\) <=> \(\left(2x\right)^2-2.2x.3+3^2=0\) <=> \(\left(2x-3\right)^2=0\) <=> \(2x-3=0\) <=> \(x=\frac{3}{2}\) x2 - 5x = 0 => x(x - 5) = 0 => \(\orbr{\begin{cases}x=0\\x-5=0\end{cases}}\) => \(\orbr{\begin{cases}x=0\\x=5\end{cases}}\) b) (3x - 5)2 - 4 = 0 => (3x - 5)2 = 0 + 4 => (3x - 5)2 = 4 => (3x - 5)2 = 22 => \(\orbr{\begin{cases}3x-5=2\\3x-5=-2\end{cases}}\) => \(\orbr{\begin{cases}3x=7\\3x=3\end{cases}}\) => \(\orbr{\begin{cases}x=\frac{7}{3}\\x=1\end{cases}}\)