HP
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
24 tháng 2 2022
2: \(\text{Δ}=1^2-4\cdot\left(-1\right)\cdot\left(-m\right)=1-4m\)
Để bất phương trình vô nghiệm thì \(\left\{{}\begin{matrix}1-4m< 0\\-1< 0\end{matrix}\right.\Leftrightarrow m>\dfrac{1}{4}\)
NV
Nguyễn Việt Lâm
Giáo viên
22 tháng 3 2022
a.
Pt có 2 nghiệm pb khi:
\(\left\{{}\begin{matrix}m+1\ne0\\\Delta'=\left(m+3\right)^2-\left(m+1\right)\left(-m+2\right)>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne-1\\2m^2+7m+7>0\left(\text{luôn đúng}\right)\end{matrix}\right.\)
\(\Rightarrow m\ne-1\)
b.
BPT vô nghiệm khi \(\left(m^2-4m-5\right)x^2+2\left(m-5\right)-1< 0\) nghiệm đúng với mọi x
- Với \(m=-1\) ko thỏa mãn
- Với \(m=5\) thỏa mãn
- Với \(m\ne\left\{-1;5\right\}\)
\(\Rightarrow\left\{{}\begin{matrix}m^2-4m-5< 0\\\Delta'=\left(m-5\right)^2+m^2-4m-5< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-1< m< 5\\\left(m-5\right)\left(2m-4\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-1< m< 5\\2< m< 5\end{matrix}\right.\) \(\Rightarrow2< m< 5\)
Kết hợp lại ta được: \(2< m\le5\)
NP
1
24 tháng 1 2024
\(x^2-2\left(m-1\right)x+4m+8< 0\)
\(\text{Δ}=\left[-2\left(m-1\right)\right]^2-4\cdot1\cdot\left(4m+8\right)\)
\(=4m^2-4m+1-16m+32\)
\(=4m^2-20m+33\)
Để BPT vô nghiệm thì \(\left\{{}\begin{matrix}\text{Δ}< =0\\a>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4m^2-20m+33< =0\\1>0\left(đúng\right)\end{matrix}\right.\)
=>\(4m^2-20m+33< =0\)
=>\(\left(2m-5\right)^2+8< =0\)(vô lý)
=>\(m\in\varnothing\)
28 tháng 7 2021
\(mx^2-2\left(m+2\right)x+2m-1< 0\)
\(< =>mx^2-2\left(m+2\right)x+2m-1\ge0\)
\(a=m\ne0\)
\(\Delta=\left(2m+2\right)^2-4m\left(2m-1\right)\)
\(\Delta=4m^2+8m+4-8m^2+4m\)
\(\Delta=12m-4m^2+4\)
\(< =>\hept{\begin{cases}a>0\\\Delta\le0\end{cases}\hept{\begin{cases}m>0\\12m-4m^2+4\le0\end{cases}\hept{\begin{cases}m>0\\m=\left[\frac{3-\sqrt{13}}{2};\frac{3+\sqrt{13}}{2}\right]\end{cases}}}}\)
\(< =>m=(0;\frac{3+\sqrt{13}}{2}]\)
vậy m vô số nghiệm để bpt vô nghiệm
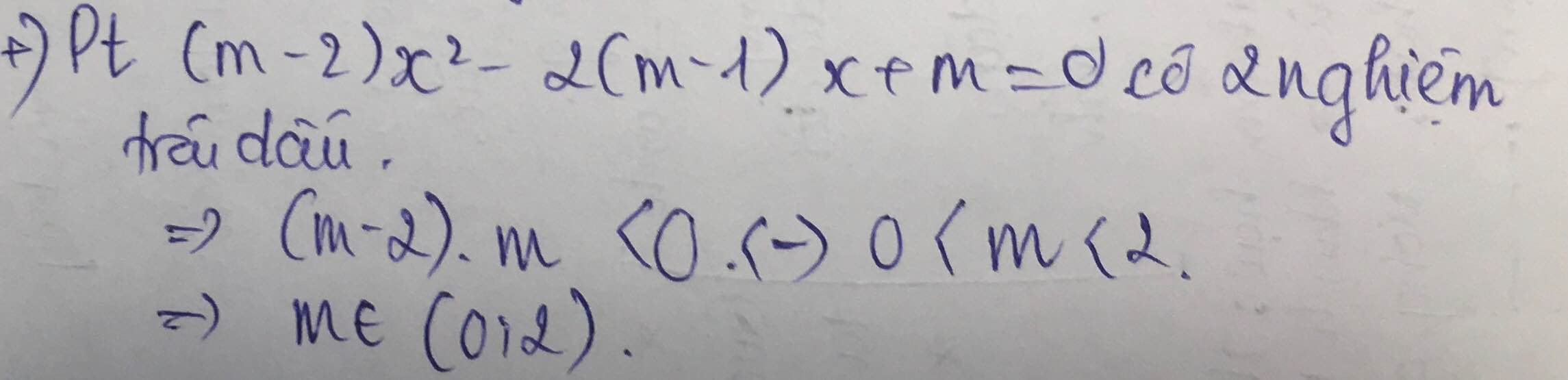
- Với \(m=1\) BPT trở thành \(1\le0\) (vô nghiệm) thỏa mãn
- Với \(m\ne1\) BPT đã cho vô nghiệm khi \(\left(m-1\right)x^2+2\left(m-1\right)x+1>0\) nghiệm đúng với mọi x
\(\Leftrightarrow\left\{{}\begin{matrix}m-1>0\\\Delta'=\left(m-1\right)^2-\left(m-1\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m>1\\1< m< 2\end{matrix}\right.\) \(\Rightarrow1< m< 2\)
Kết hợp lại ta được: \(1\le m< 2\)
- Với �=1m=1 BPT trở thành 1≤01≤0 (vô nghiệm) thỏa mãn
- Với �≠1m=1 BPT đã cho vô nghiệm khi (�−1)�2+2(�−1)�+1>0(m−1)x2+2(m−1)x+1>0 nghiệm đúng với mọi x
⇔{�−1>0Δ′=(�−1)2−(�−1)<0⇔{m−1>0Δ′=(m−1)2−(m−1)<0
⇔{�>11<�<2⇔{m>11<m<2 ⇒1<�<2⇒1<m<2
Kết hợp lại ta được: 1≤�<21≤m<2