Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đáp án B
Đặt t = 3sin x - 4 cos x => -5 ≤ t ≤ 5
Ta có: y = t2 – 2t + 2m – 1 = (t – 1)2 + 2m - 2
Với mọi t ta có (t – 1)2 ≥ 0 nên y ≥ 2m - 2 => min y = 2m - 2
Hàm số chỉ nhận giá trị dương ⇔ y > 0 ∀x ∈ R ⇔ min y > 0
⇔ 2m - 2 > 0 ⇔ m > 1


Đặt \(a=3sinx-4cosx\Rightarrow a^2\le\left(3^2+4^2\right)\left(sin^2x+cos^2x\right)=25\)
\(\Rightarrow-5\le a\le5\)
\(y=a^2-2a+1\ge2m\)
\(\Rightarrow\left(a-1\right)^2\ge2m\)
Để BPT đúng với mọi x thuộc R
\(\Leftrightarrow2m\le\min\limits_{\left[-5;5\right]}\left(a-1\right)^2\)
Mà \(\left(a-1\right)^2\ge0\) \(\forall a\Rightarrow2m\le0\Rightarrow m\le0\)

Đặt \(t=cosx;t\in\left[-1;1\right]\)
Để hàm số có tập xác định R
\(\Leftrightarrow cosx^2-\left(2+m\right)cosx+2m\ge0;\forall x\)
\(\Leftrightarrow t^2-\left(2+m\right)t+2m\ge0\) với mọi \(t\in\left[-1;1\right]\)
Đặt \(f\left(t\right)=t^2-\left(2+m\right)t+2m\); \(I\left(\dfrac{2+m}{2};f\left(\dfrac{2+m}{2}\right)\right)\)
TH1: \(\dfrac{2+m}{2}< -1\) \(\Leftrightarrow m< -4\)
Để \(f\left(t\right)\ge0;\forall t\in\left[-1;1\right]\) \(\Leftrightarrow\)\(f\left(t\right)_{min}=f\left(-1\right)\ge0\) \(\Leftrightarrow3+3m\ge0\Leftrightarrow m\ge-1\)(ktm đk)
TH2: \(-1\le\dfrac{m+2}{2}\le1\)\(\Leftrightarrow-4\le m\le0\)
Để \(f\left(t\right)\ge0;\forall t\in\left[-1;1\right]\) \(\Leftrightarrow f\left(t\right)_{min}=f\left(\dfrac{2+m}{2}\right)\ge0\)\(\Leftrightarrow-m^2+4m-4\ge0\)\(\Leftrightarrow m=2\) (ktm đk)
TH3:\(\dfrac{m+2}{2}>1\) \(\Leftrightarrow m>0\)
Để \(f\left(t\right)\ge0;\forall t\in\left[-1;1\right]\)\(\Leftrightarrow f\left(t\right)_{min}=f\left(1\right)\ge0\)\(\Leftrightarrow m-1\ge0\Leftrightarrow m\ge1\)
Kết hợp cả ba TH \(\Rightarrow m\ge1\)
Vậy...
Đơn giản hơn:
\(t^2-\left(m+2\right)t+2m\ge0\) ; \(\forall t\in\left[-1;1\right]\)
\(\Leftrightarrow t\left(t-2\right)-m\left(t-2\right)\ge0\)
\(\Leftrightarrow\left(t-m\right)\left(t-2\right)\ge0\) (1)
Do \(t-2< 0\) ; \(\forall t\in\left[-1;1\right]\) nên (1) tương đương:
\(t-m\le0\)
\(\Leftrightarrow m\ge t\) ; \(\forall t\in\left[-1;1\right]\)
\(\Rightarrow m\ge1\)

\(\Leftrightarrow\left(3sinx-4cosx\right)^2-2\left(3sinx-4cosx\right)\le2m-1\)
Đặt \(3sinx-4cosx=5\left(\frac{3}{5}sinx-\frac{4}{5}cosx\right)=5sin\left(x-a\right)=t\)
\(\Rightarrow-5\le t\le5\)
BPT trở thành: \(t^2-2t+1\le2m\)
\(\Leftrightarrow\left(t-1\right)^2\le2m\)
Để pt nghiệm đúng với mọi x thì \(2m\ge\max\limits_{\left[-5;5\right]}\left(t-1\right)^2\)
Mà \(\left(t-1\right)^2\le\left(-5-1\right)^2=36\)
\(\Rightarrow2m\ge36\Rightarrow m\ge18\)
Có \(2019-18+1=2002\) giá trị
Không đáp án nào đúng

ĐKXĐ: 2m-3sinx>=0
=>3sin x<=2m
=>sin x<=2m/3
mà -1<=sin x<=1
nên -1<=2m/3<=1
=>-3<=2m<=3
=>-3/2<=m<=3/2

Hàm xác định trên R khi và chỉ khi \(cos^2x-\left(m+2\right)cosx+2m\ge0\) ;\(\forall x\)
\(\Leftrightarrow cos^2x-2cosx-\left(m.cosx-2m\right)\ge0\)
\(\Leftrightarrow cosx\left(cosx-2\right)-m\left(cosx-2\right)\ge0\)
\(\Leftrightarrow\left(cosx-m\right)\left(cosx-2\right)\ge0\) ;\(\forall x\) (1)
Mà \(cosx\le1\Rightarrow cosx-2< 0\)
\(\left(1\right)\Leftrightarrow cosx-m\le0\) ;\(\forall x\)
\(\Leftrightarrow m\ge cosx;\) \(\forall x\Leftrightarrow m\ge max\left(cosx\right)=1\)
Vậy \(m\ge1\)

Đáp án D
Đặt t = 3sin x - 4cos x => -5 ≤ t ≤ 5 (dùng bất đẳng thức bunhiacopxki)
Ta có: y = (3sin x – 4cos x)2 – 6sin x + 8cos x
= t2 – 2t = (t – 2)2 -1
Do -5 ≤ t ≤ 5 => 0 ≤ (t – 2)2 ≤ 36 => min y = -1
Suy ra yêu cầu bài toán -1 ≥ 2m - 1 ⇔ m ≤ 0.
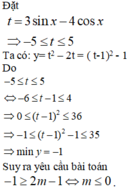
Hàm xác định trên R khi và chỉ khi:
\(8cosx-6sinx-\left(3sinx-4cosx\right)^2-2m\ge0;\forall x\) (1)
Đặt \(3sinx-4cosx=t\)
\(\Rightarrow t^2=\left(3sinx-4cosx\right)^2\le\left(3^2+\left(-4\right)^2\right)\left(sin^2x+cos^2x\right)=25\)
\(\Rightarrow-5\le t\le5\)
(1) tương đương:
\(-2t-t^2-2m\ge0;\forall t\in\left[-5;5\right]\)
\(\Leftrightarrow2m\le-t^2-2t;\forall t\in\left[-5;5\right]\)
\(\Leftrightarrow2m\le\min\limits_{t\in\left[-5;5\right]}\left(-t^2-2t\right)\)
Xét hàm \(f\left(t\right)=-t^2-2t\) trên \(\left[-5;5\right]\)
\(-\dfrac{b}{2a}=-1\) ; \(f\left(-5\right)=-15\) ; \(f\left(-1\right)=1\) ; \(f\left(5\right)=-35\)
\(\Rightarrow2m\le-35\Rightarrow m\le-\dfrac{35}{2}\)