Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

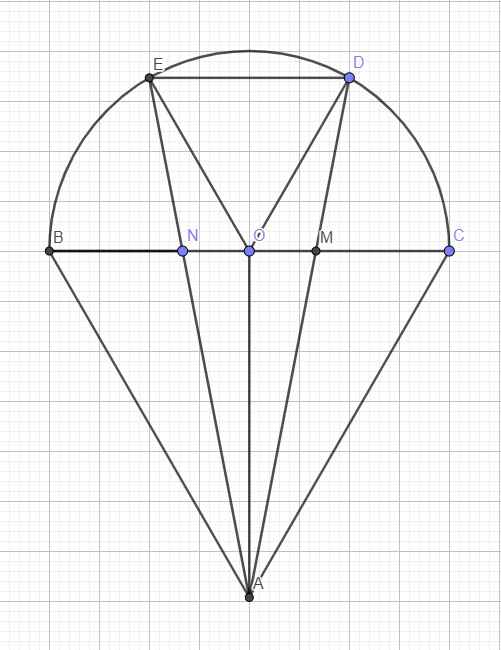
Gọi O là tâm đường tròn \(\Rightarrow\) O là trung điểm BC
\(\stackrel\frown{BE}=\stackrel\frown{ED}=\stackrel\frown{DC}\Rightarrow\widehat{BOE}=\widehat{EOD}=\widehat{DOC}=\dfrac{180^0}{3}=60^0\)
Mà \(OD=OE=R\Rightarrow\Delta ODE\) đều
\(\Rightarrow ED=R\)
\(BN=NM=MC=\dfrac{2R}{3}\Rightarrow\dfrac{NM}{ED}=\dfrac{2}{3}\)
\(\stackrel\frown{BE}=\stackrel\frown{DC}\Rightarrow ED||BC\)
Áp dụng định lý talet:
\(\dfrac{AN}{AE}=\dfrac{MN}{ED}=\dfrac{2}{3}\Rightarrow\dfrac{EN}{AN}=\dfrac{1}{2}\)
\(\dfrac{ON}{BN}=\dfrac{OB-BN}{BN}=\dfrac{R-\dfrac{2R}{3}}{\dfrac{2R}{3}}=\dfrac{1}{2}\)
\(\Rightarrow\dfrac{EN}{AN}=\dfrac{ON}{BN}=\dfrac{1}{2}\) và \(\widehat{ENO}=\widehat{ANB}\) (đối đỉnh)
\(\Rightarrow\Delta ENO\sim ANB\left(c.g.c\right)\)
\(\Rightarrow\widehat{NBA}=\widehat{NOE}=60^0\)
Hoàn toàn tương tự, ta có \(\Delta MDO\sim\Delta MAC\Rightarrow\widehat{MCA}=\widehat{MOD}=60^0\)
\(\Rightarrow\Delta ABC\) đều

Gọi số ngày hoàn thành công việc nếu làm riêng của người thứ nhất là x, người thứ 2 là y(ngày),(x,y>0)
1 ngày người thứ nhất làm được:\(\frac{1}{x}\)
1 ngày người thứ hai làm được:\(\frac{1}{y}\)
=> 1 ngày cả người làm được:\(\frac{1}{x}+\frac{1}{y}=\frac{1}{12}\)(1)
3 ngày người thứ nhất làm được:\(\frac{3}{x}\)
Vì sau 3 ngày, người thứ 2 làm nốt 15 ngày nên: Số ngày người thứ 2 làm là 15+3=18
18 ngày người thứ hai làm được \(\frac{18}{x}\)
Do đó, ta được:\(\frac{3}{x}+\frac{18}{y}=1\)(2)
Từ (1) và (2) , ta có hệ: \(\hept{\begin{cases}\frac{1}{x}+\frac{1}{y}=\frac{1}{12}\\\frac{3}{x}+\frac{18}{y}=1\end{cases}}\)
Đặt \(\frac{1}{x}\)= a, \(\frac{1}{y}\)= b, ta được
\(\hept{\begin{cases}a+b=\frac{1}{12}\\3a+18b=1\end{cases}}\)<=>\(\hept{\begin{cases}a=\frac{1}{30}\\b=\frac{1}{20}\end{cases}}\)<=>\(\hept{\begin{cases}x=30\\y=20\end{cases}}\). Vậy......



Bài 1:
Kẻ \(OM\perp AB\), \(OM\)cắt \(CD\)tại \(N\).
Khi đó \(MN=8cm\).
TH1: \(AB,CD\)nằm cùng phía đối với \(O\).
\(R^2=OC^2=ON^2+CN^2=h^2+\left(\frac{25}{2}\right)^2\)(\(h=CN\)) (1)
\(R^2=OA^2=OM^2+AM^2=\left(h+8\right)^2+\left(\frac{15}{2}\right)^2\)(2)
Từ (1) và (2) suy ra \(R=\frac{\sqrt{2581}}{4},h=\frac{9}{4}\).
TH2: \(AB,CD\)nằm khác phía với \(O\).
\(R^2=OC^2=ON^2+CN^2=h^2+\left(\frac{25}{2}\right)^2\)(\(h=CN\)) (3)
\(R^2=OA^2=OM^2+AM^2=\left(8-h\right)^2+\left(\frac{15}{2}\right)^2\)(4)
Từ (3) và (4) suy ra \(R=\frac{\sqrt{2581}}{4},h=\frac{-9}{4}\)(loại).
Bài 3:
Lấy \(A'\)đối xứng với \(A\)qua \(Ox\), khi đó \(A'\)có tọa độ là \(\left(1,-2\right)\).
\(MA+MB=MA'+MB\ge A'B\)
Dấu \(=\)xảy ra khi \(M\)là giao điểm của \(A'B\)với trục \(Ox\).
Suy ra \(M\left(\frac{5}{3},0\right)\).

đây là bài lớp 10 chứ nhỉ
ta có \(AC=20\times2=40\text{ hải lí}\), \(AB=15\times2=30\text{ hải lí}\)
áp dụng định lý cosin ta có :
\(BC=\sqrt{AB^2+AC^2-2AB.AC\text{c}osA}=\sqrt{40^2+30^2-2\times30\times40\times cos60^o}\simeq36.06\text{ hải lí}\)

a, \(P=\frac{a^3-a+2b-\frac{b^2}{a}}{\left(1-\sqrt{\frac{a+b}{a^2}}\right)\left(a+\sqrt{a+b}\right)}:\left[\frac{a^2\left(a+b\right)+a\left(a+b\right)}{\left(a-b\right)\left(a+b\right)}+\frac{b}{a-b}\right]\)
\(=\frac{\frac{a^4-a^2-2ab-b^2}{a}}{\frac{\left(a-\sqrt{a+b}\right)\left(a+\sqrt{a+b}\right)}{a}}:\left[\frac{\left(a+b\right)\left(a^2+a\right)}{\left(a+b\right)\left(a-b\right)}+\frac{b}{a-b}\right]\)
\(=\frac{a^4-a^2-2ab-b^2}{a^2-a-b}:\frac{a^2+a+b}{a-b}\)
\(=\frac{a^4-a^2-2ab-b^2}{a^2-\left(a+b\right)}.\frac{a-b}{a^2+\left(a+b\right)}\)
\(=\frac{\left(a^4-a^2-2ab-b^2\right).\left(a-b\right)}{a^4-\left(a+b\right)^2}=\frac{\left[a^4-\left(a+b\right)^2\right].\left(a-b\right)}{a^4-\left(a+b\right)^2}=a-b\)
b, Có \(P=a-b=1\)\(\Rightarrow a=1+b\)
\(a^3-b^3=7\Leftrightarrow\left(a^2+ab+b^2\right)\left(a-b\right)=7\)
\(\Rightarrow a^2+ab+b^2=7\)
\(\Leftrightarrow\left(1+b\right)^2+\left(1+b\right)b+b^2=7\)
\(\Leftrightarrow b^2+2b+1+b^2+b+b^2=7\)
\(\Leftrightarrow3b^2+3b-6=0\)
Bạn tự giải phương trình tìm b => a
Bài 2 :
\(a,y=\left(m+1\right)x-2m-5\) \(\Leftrightarrow\left(m+1\right)x-2m-5-y=0\)
\(\Leftrightarrow mx+x-2m-5-y=0\)\(\Leftrightarrow m\left(x-2\right)+x-y-5=0\)
Có y luôn qua điểm A cố định với A( x0 ; y0 ) \(\orbr{\begin{cases}x_0-2=0\\x_0-y_0-5=0\end{cases}}\Rightarrow\orbr{\begin{cases}x_0=2\\y_0=-3\end{cases}}\)
=> A( 2;-3)
Gọi H là chân đường vuông góc hạ từ O xuống d => \(OH\le OA\)
\(OH_{max}=OA\)khi \(H\equiv A\)\(\left(d\perp OA\right)\)
=> đường thẳng OA qua O( 0;0 ) và A( 2;-3 ) => \(y=-\frac{3}{2}x\)
\(\Rightarrow d\perp OA\)=> hệ số góc \(m.\) \(-\frac{3}{2}=-1\Rightarrow m=\frac{2}{3}\)
b, \(y=0\Rightarrow\left(m+1\right)x-2m-5=0\)\(\Rightarrow x=\frac{2m+5}{m+1}\)\(\Rightarrow A\left(\frac{2m+5}{m+1};0\right)\)
\(x=0\Rightarrow y=-2m-5\Rightarrow B\left(0;-2m-5\right)\)
\(\Rightarrow OA=\sqrt{\frac{2m+5}{m+1}};OB=\sqrt{-2m-5}\)
\(\Rightarrow\frac{1}{2}.OA.OB=\frac{3}{2}\Rightarrow OA.OB=3\)
\(\Rightarrow\left(OA.OB\right)^2=9\Rightarrow\frac{\left(2m+5\right)^2}{m+1}=9\)
\(\Rightarrow4m^2+20m+25-9m-9=\)
\(\Rightarrow4m^2+11m+16=0\)

Ta có : \(\frac{AB}{AC}=\frac{1}{4}\Rightarrow AB=\frac{1}{4}AC\)
Xét tam giác ABC vuông tại A, đường cao AH
* Áp dụng hệ thức : \(\frac{1}{AH^2}=\frac{1}{AB^2}+\frac{1}{AC^2}\Leftrightarrow\frac{1}{64}=\frac{1}{\left(\frac{1}{4}AC\right)^2}+\frac{1}{AC^2}\Leftrightarrow AC=8\sqrt{17}\)cm
\(\Rightarrow AB=\frac{8\sqrt{17}}{4}=2\sqrt{17}\)cm
Theo định lí Pytago tam giác ABC vuông tại A
\(BC=\sqrt{AB^2+AC^2}=34\)cm
* Áp dụng hệ thức : \(AB^2=BH.BC\Rightarrow BH=\frac{AB^2}{BC}=2\)cm
-> HC = BC - HB = 32 cm
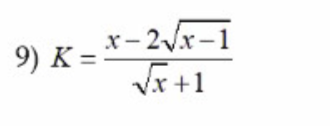






 Giải giúp mình với ạ, cần gấp lắm, cảm ơn
Giải giúp mình với ạ, cần gấp lắm, cảm ơn

Lời giải:
$K=\frac{x-2\sqrt{x-1}}{\sqrt{x}+1}=\frac{(x-1)-2\sqrt{x-1}+1}{\sqrt{x}+1}$
$=\frac{(\sqrt{x-1}-1)^2}{\sqrt{x}+1}$
Ta thấy:
$(\sqrt{x-1}-1)^2\geq 0$ với mọi $x\geq 0$
$\sqrt{x}+1>0$
$\Rightarrow K\geq 0$
Vậy $K_{\min}=0$. Giá trị này đạt tại $\sqrt{x-1}-1=0$
$\Leftrightarrow x=2$