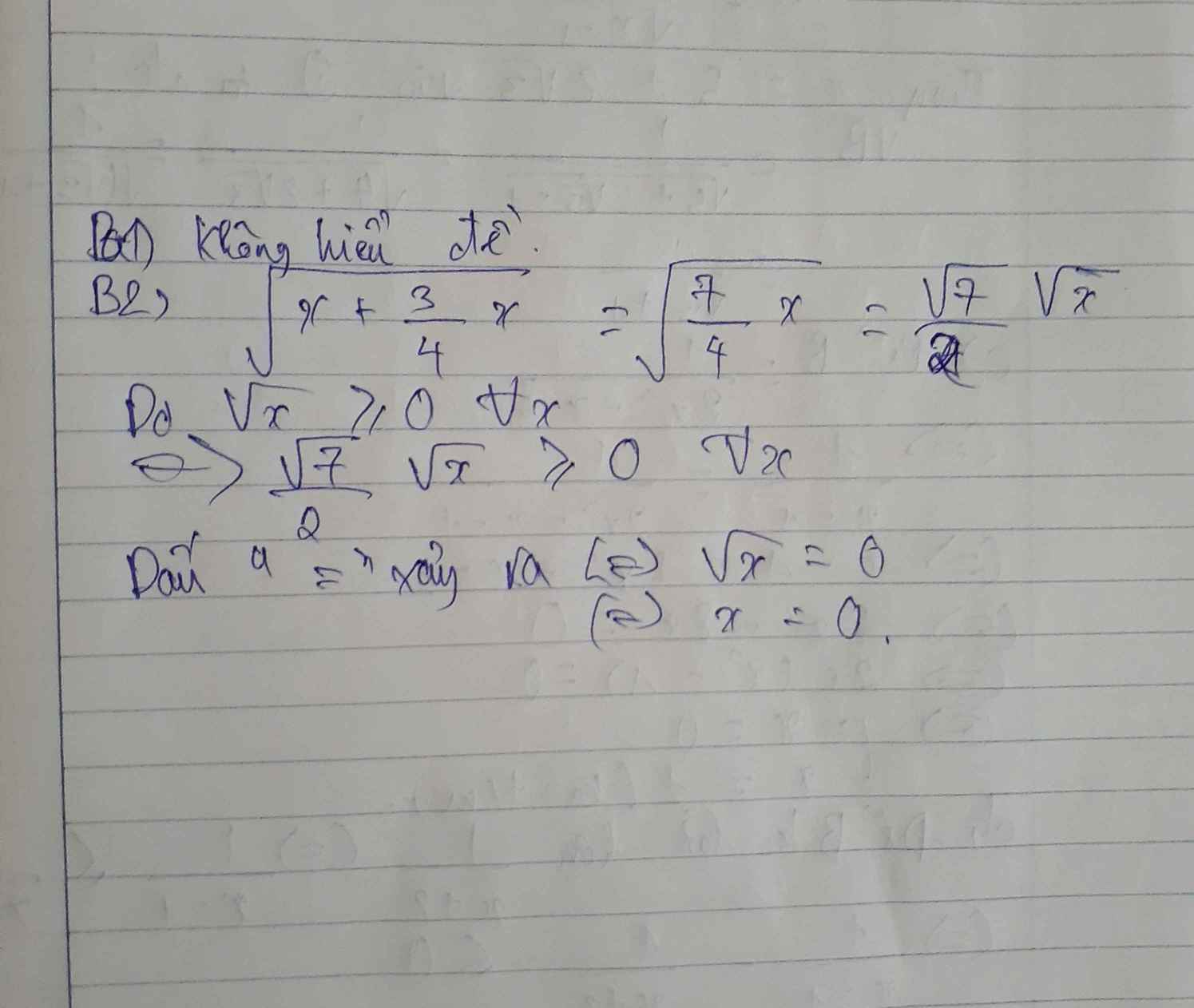K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
TT
1
16 tháng 7 2021
Ta có: \(\sqrt{9x+9}+\sqrt{4x+4}-2\sqrt{16x+16}=\sqrt{x+1}-8\)
\(\Leftrightarrow3\sqrt{x+1}+2\sqrt{x+1}-8\sqrt{x+1}-\sqrt{x+1}=-8\)
\(\Leftrightarrow\sqrt{x+1}=2\)
\(\Leftrightarrow x+1=4\)
hay x=3
17 tháng 5 2021
`A=(1/(x-sqrtx)+1/(sqrtx-1)):(sqrtx+1)/(sqrtx-1)^2`
`=((sqrtx+1)/(x-sqrtx)).(sqrtx-1)^2/(sqrtx+1)`
`=(sqrtx-1)^2/(x-sqrtx)`
`=(sqrtx-1)/sqrtx`
TN
0
25 tháng 8 2021
Ta có \(\dfrac{2\sqrt{x}}{x+1}=\dfrac{2}{\sqrt{x}+\dfrac{1}{\sqrt{x}}}\)
Áp dụng BĐT cosi, ta có:
\(\sqrt{x}+\dfrac{1}{\sqrt{x}}\ge2\Leftrightarrow\dfrac{1}{\sqrt{x}+\dfrac{1}{\sqrt{x}}}\le\dfrac{1}{2}\\ \Leftrightarrow\dfrac{2}{\sqrt{x}+\dfrac{1}{\sqrt{x}}}\le1\)
Vậy GTLN của \(\dfrac{2\sqrt{x}}{x+1}\) là 1. Dấu \("="\) xảy ra \(\Leftrightarrow\sqrt{x}=\dfrac{1}{\sqrt{x}}\Leftrightarrow x=1\)