
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

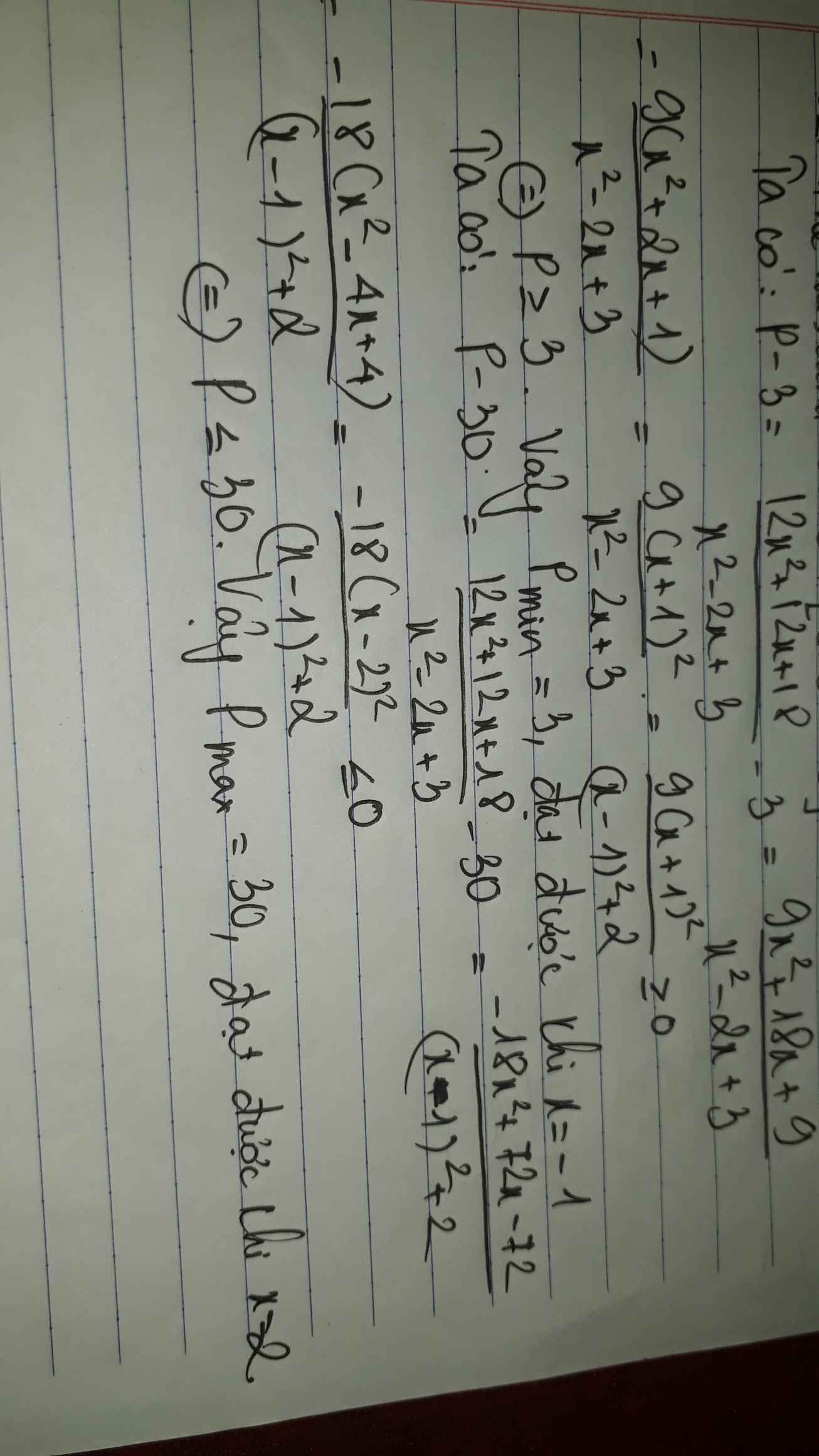


GTNN :\(A=\frac{\left(2x^2+2\right)+\left(x^2-2x+1\right)}{x^2+1}=2+\frac{\left(x-1\right)^2}{x^2+1}\ge2\forall x\) có GTNN là 2
GTLN : \(A=\frac{\left(4x^2+4\right)-\left(x^2+2x+1\right)}{x^2+1}=4-\frac{\left(x+1\right)^2}{x^2+1}\le4\forall x\) có GTLN là 4

\(Y=\sqrt{\left(3x+2\right)^2+7}\ge\sqrt{0+7}=\sqrt{7}\)
\(Y_{Min}=\sqrt{7}\Leftrightarrow3x+2=0\Leftrightarrow x=-\frac{2}{3}\)

\(M=2x+\sqrt{5-x^2}\)
\(\Leftrightarrow M-2x=\sqrt{5-x^2}\)
\(\Leftrightarrow M^2-4Mx+4x^2=5-x^2\)
\(\Leftrightarrow5x^2-4Mx+M^2-5=0\)
Để PT theo nghiệm x có nghiệm thì
\(\Delta'=4M^2-5.\left(M^2-5\right)\ge0\)
\(\Leftrightarrow M^2\le25\)
\(\Leftrightarrow-5\le M\le5\)

Đặt \(C=\sqrt{4x^2-4x+1}+\sqrt{4x^2-12x+9}\)
\(=\sqrt{\left(2x-1\right)^2}+\sqrt{\left(2x-3\right)^2}\)
\(=\left|2x-1\right|+\left|2x-3\right|\)
\(=\left|2x-1\right|+\left|3-2x\right|\)
\(\ge\left|\left(2x-1\right)+\left(3-2x\right)\right|=\left|2\right|=2\)
Vậy \(C_{min}=2\)

\(A=\frac{3-4x}{2x^2+2}\)
\(\Leftrightarrow2Ax^2+2A=3-4x\)
\(\Leftrightarrow2Ax^2+4x+2A-3=0\)
*Nếu A = 0 thì \(x=\frac{3}{4}\)
*Nếu A # 0 thì pt trên là pt bậc 2
Pt có nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow4-2A\left(2A-3\right)\ge0\)
\(\Leftrightarrow4-4A^2+6A\ge0\)
\(\Leftrightarrow-\frac{1}{2}\le A\le2\)
Vì \(-\frac{1}{2}< 0\Rightarrow\hept{\begin{cases}A_{min}=-\frac{1}{2}\Leftrightarrow x=...\\A_{max}=2\Leftrightarrow x=...\end{cases}}\)(CHỗ ... là tự làm nhé)

Em kiểm tra lại đề bài nhé:
\(\hept{\begin{cases}3x+2y=-1\\12x-my=2\end{cases}\Leftrightarrow}\hept{\begin{cases}3x+2y+1=0\\12x-my-2=0\end{cases}}\)
=> \(T=\left(3x+2y+1\right)^2+\left(12x-my-2\right)^2=0^2+0^2=0\)