Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
cho đến khi màn hình hiện ra 
để lấy 4 chữ số phần thập phân. Kết quả hiện ra trên màn hình là 8183.0047.
a. \(\left\{{}\begin{matrix}3x-5y=6\\4x+7y=-8\end{matrix}\right.\)
\(x=\dfrac{2}{41}\) ; \(y=\dfrac{-48}{41}\)
b. \(\left\{{}\begin{matrix}\text{−2x+3y=5}\\5x+2y=4\end{matrix}\right.\)
\(x=\dfrac{2}{19};y=\dfrac{33}{19}\)
c.\(\left\{{}\begin{matrix}\text{2x−3y+4z=−5}\\-4x+5y-z=6\\3x+4y-3z=7\end{matrix}\right.\)
\(x=\dfrac{22}{101};y=\dfrac{131}{101};z=\dfrac{-39}{101}\)
d. \(\left\{{}\begin{matrix}\text{− x + 2 y − 3 z = 2}\\2x+y+2z=-3\\-2x-3y+z=5\end{matrix}\right.\)
\(x=-4;y=\dfrac{11}{7};z=\dfrac{12}{7}\)
a)x=0,05 ; y=-1,17
b.x=0,11 ; y=1,74
c.x=0,22 ;y=1,29 z=-0.39
d.x=-4 y=1,57 z=1,71
\(\left(\left|\overrightarrow{a}+\overrightarrow{b}\right|\right)^2=a^2+2ab.\cos30^0+b^2\)
\(=4+2.2.\sqrt{3}.\frac{\sqrt{3}}{2}+3=13\)
\(\Rightarrow\left|\overrightarrow{a}+\overrightarrow{b}\right|=\sqrt{13}\)
1: ĐKXĐ: \(x^3-6x^2+11x-6< >0\)
\(\Leftrightarrow x^3-x^2-5x^2+5x+6x-6\ne0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2-5x+6\right)\ne0\)
\(\Leftrightarrow\left(x-1\right)\left(x-2\right)\left(x-3\right)\ne0\)
hay \(x\notin\left\{1;2;3\right\}\)
2; ĐKXĐ: \(\left\{{}\begin{matrix}3-2x>=0\\x+1< >-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< =\dfrac{3}{2}\\x< >-2\end{matrix}\right.\)
3: ĐKXĐ: \(\left\{{}\begin{matrix}x+2< >0\\x-1< >0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< >-2\\x< >1\end{matrix}\right.\Leftrightarrow x\in R\)
\(A=\sqrt[3]{2-\sqrt{5}}\left(\sqrt[6]{\left(2+\sqrt{5}\right)^2}+\sqrt[3]{2+\sqrt{5}}\right)\)
\(=\sqrt[3]{2-\sqrt{5}}.2\sqrt[3]{2+\sqrt{5}}=2\sqrt[3]{4-5}=-2\)
\(B=\sqrt[4]{\left(3-2\sqrt{2}\right)^2}-\sqrt{2}=\sqrt{3-2\sqrt{2}}-\sqrt{2}\)
\(=\sqrt{\left(\sqrt{2}-1\right)^2}-\sqrt{2}=\sqrt{2}-1-\sqrt{2}=-1\)
\(C=\sqrt[4]{\left(6-2\sqrt{5}\right)^2}=\sqrt{6-2\sqrt{5}}=\sqrt{\left(\sqrt{5}-1\right)^2}=\sqrt{5}-1\)
\(D=1+\sqrt[4]{\left(4-2\sqrt{3}\right)^2}=1+\sqrt{4-2\sqrt{3}}\)
\(=1+\sqrt{\left(\sqrt{3}-1\right)^2}=1+\sqrt{3}-1=\sqrt{3}\)
Câu e lấy nguyên văn từ sách thầy Vũ Hữu Bình:
Đặt \(x=\sqrt[4]{5}\Rightarrow x^4=5\Rightarrow5-x^4=0\)
\(E=\frac{2}{\sqrt{4-3x+2x^2-x^3}}=\frac{2\left(x+1\right)}{\sqrt{\left(x+1\right)^2\left(4-3x+2x^2-x^3\right)}}=\frac{2\left(x+1\right)}{\sqrt{-x^5+5x+4}}\)
\(E=\frac{2\left(x+1\right)}{\sqrt{x\left(5-x^4\right)+4}}=\frac{2\left(x+1\right)}{\sqrt{4}}=x+1=\sqrt[4]{5}+1\)
Không hiểu ý tưởng nhân cả tử và mẫu với \(x+1\) từ đâu ra luôn
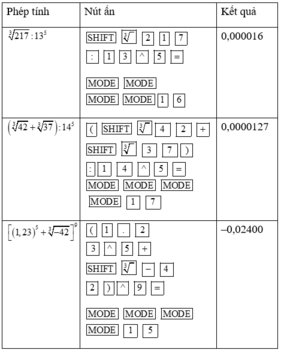
a) Nếu dùng máy tính CASIO fx-500 MS ta làm như sau
Ấn
Ấn liên tiếp phím
cho đến khi màn hình hiện ra
Ấn liên tiếp
để lấy chữ số thập phân.
Kết quả hiện ra trên màn hình là 0.000016.
b)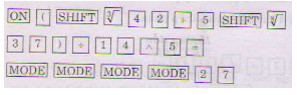
Kết quả 1029138.10-5
c)
Kết quả: -2,3997.10-2.