Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(1-2cos^2x-sinx=0\)
\(\Leftrightarrow1-2\left(1-sin^2x\right)-sinx=0\)
\(\Leftrightarrow2sin^2x-sinx-1=0\Rightarrow\left[{}\begin{matrix}sinx=1\\sinx=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k2\pi\\x=-\dfrac{\pi}{6}+k2\pi\\x=\dfrac{7\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Rightarrow x=\left\{\dfrac{\pi}{2};\dfrac{7\pi}{6};\dfrac{11\pi}{6};\dfrac{5\pi}{2}\right\}\)
\(\Rightarrow\sum x=6\pi\)

D=sin(pi+x)+sinx+cot(pi-x)+tan(pi/2-x)
=-sinx+sinx-cotx+cotx=0

ĐKXĐ:
a. \(cos\left(x-\dfrac{2\pi}{3}\right)\ne0\Rightarrow x-\dfrac{2\pi}{3}\ne\dfrac{\pi}{2}+k\pi\Rightarrow x\ne\dfrac{\pi}{6}+k\pi\)
b. \(sin\left(x+\dfrac{\pi}{6}\right)\ne0\Rightarrow x+\dfrac{\pi}{6}\ne k\pi\Rightarrow x\ne-\dfrac{\pi}{6}+k\pi\)
c. \(\dfrac{1+x}{2-x}\ge0\Rightarrow-1\le x< 2\)

pi<x<3/2pi
=>cosx<0
pi<x<3/2pi
=>pi/2<1/2x<3/4pi
=>cos(x/2)<0
1+tan^2x=1/cos^2x
=>1/cos^2x=1+8=9
=>cosx=-1/3
\(cosx=2\cdot cos^2\left(\dfrac{x}{2}\right)-1\)
=>\(2\cdot cos^2\left(\dfrac{x}{2}\right)=\dfrac{2}{3}\)
=>\(cos^2\left(\dfrac{x}{2}\right)=\dfrac{1}{3}\)
=>cos(x/2)=1/căn 3
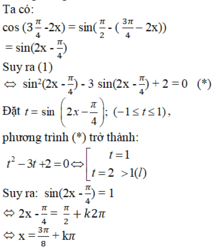

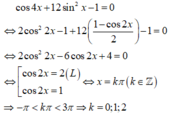
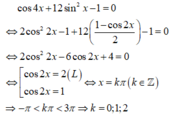


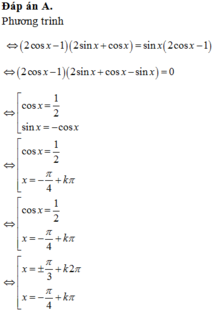
Chọn A