BP
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NA
0
SD
0
CD
0
BP
0
QH
2
QM
1
23 tháng 6 2021
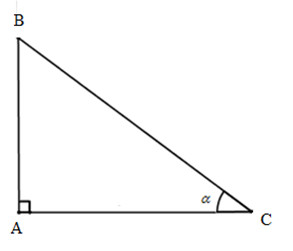
Vì tam giác ABC vuông nên ta có:
\(\text{cosB=sinC=0,8}\)
\(\text{cosC=}\)\(\sqrt{1-sin^2C}\) (theo công thức trong SGK ^^)=\(\sqrt{1-0,8^2}=0,6\)
\(tangC=\dfrac{sinC}{cosC}=\dfrac{0,8}{0,6}=\dfrac{4}{3}\left(\approx1,3\right)\)
\(cotangC=\dfrac{cosC}{sinC}=\dfrac{0,6}{0,8}=0,75\)
NV
Nguyễn Việt Lâm
Giáo viên
9 tháng 8 2021
Ta có:
\(cosB=\dfrac{AB}{BC}\Rightarrow AB=BC.cosB=10.0,8=8\left(cm\right)\)
\(AC=\sqrt{BC^2-AB^2}=6\left(cm\right)\)
b.
\(sinC=\dfrac{AB}{BC}=\dfrac{8}{10}=0,8\)
\(cosC=\dfrac{AC}{BC}=\dfrac{6}{10}=0,6\)
\(tanC=\dfrac{AB}{AC}=\dfrac{8}{6}=\dfrac{4}{3}\)
\(cotC=\dfrac{AC}{AB}=\dfrac{3}{4}\)
DT
1
10 tháng 9 2019
Vì tam giác ABC vuông tại A nên góc C nhọn. Vì thế:
sinC>0;cosC>0;tanC>0;cotC>0sinC>0;cosC>0;tanC>0;cotC>0
Vì hai góc B và C phụ nhau nên sinC = cosB = 0,8.
Ta có:
Sin2C+cos2C=1Sin2C+cos2C=1
⇒cos2C=1−sin2C=1−(0,8)2=0,36⇒cos2C=1−sin2C=1−(0,8)2=0,36
⇒cosC=0,6;⇒cosC=0,6;
tgC=sinCcosC=0,80,6=43;tgC=sinCcosC=0,80,6=43;
cotgC=cosCsinC=0,60,8=34