UI
11
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PG
1
14 tháng 8 2020
ta có bđt \(\left(\frac{a+b}{2}\right)^n\le\frac{a^n+b^n}{2}\) với mọi \(a+b\ge0\) và \(n\inℝ\)
\(1+\sqrt[1995]{1995}=2\sqrt[1995]{\left(\frac{1+\sqrt[1995]{1995}}{2}\right)^{1995}}\le2\sqrt[1995]{\frac{1+1995}{2}}=2\sqrt[1995]{\frac{1996}{2}}\)
\(=\sqrt[1995]{2^{1994}.1996}=\sqrt[1995]{2.2...2.1996}< \sqrt[1995]{2.3...1995.1996}=\sqrt[1995]{1996!}\)
17 tháng 8 2021
1, A=\(\left(1-\dfrac{2\sqrt{a}}{a+1}\right):\left(\dfrac{1}{\sqrt{a}+1}-\dfrac{2\sqrt{a}}{a\sqrt{a}+\sqrt{a}+a+1}\right)\)
ĐKXĐ: a≥0
A=\(\left(1-\dfrac{2\sqrt{a}}{a+1}\right):\left(\dfrac{1}{\sqrt{a}+1}-\dfrac{2\sqrt{a}}{\sqrt{a}\left(a+1\right)+1\left(a+1\right)}\right)\)
A=\(\left(\dfrac{a+1}{a+1}-\dfrac{2\sqrt{a}}{a+1}\right):\left(\dfrac{a+1}{\left(\sqrt{a}+1\right)\left(a+1\right)}-\dfrac{2\sqrt{a}}{\left(\sqrt{a}+1\right)\left(a+1\right)}\right)\)
A=\(\left(\dfrac{a+1-2\sqrt{a}}{a+1}\right):\left(\dfrac{a+1-2\sqrt{a}}{\left(\sqrt{a}+1\right)\left(a+1\right)}\right)\)
A=\(\left(\dfrac{a+1-2\sqrt{a}}{a+1}\right).\left(\dfrac{\left(a+1\right)\left(\sqrt{a}+1\right)}{a+1-2\sqrt{a}}\right)\)
A=\(\sqrt{a}+1\)
Vậy A=\(\sqrt{a}+1\)
2, a=1996-2\(\sqrt{1995}\)
a=\(1995-2\sqrt{1995}+1\)
a=\(\left(\sqrt{1995}-1\right)^2\) (TMĐKXĐ)
thay a=\(\left(\sqrt{1995}-1\right)^2\) vào A ta có:
A=\(\sqrt{\left(\sqrt{1995}-1\right)^2}+1\)
A=\(\sqrt{1995}\)
Vậy a=1996-2\(\sqrt{1995}\) thì A=\(\sqrt{1995}\)
NT
2
1 tháng 11 2017
a,hay \(\left(1995\cdot1997\right)^n\)và \(\left(1996\cdot1996\right)^n\)
hay so sánh \(1995\cdot1997\)và \(1996\cdot1996\)
ta có 1995*1997=1995*(1996+1)=1995*1996+1995
1996*1996=1996*(1995+1)=1996*1995+1996
vì 1995<1996 => \(\left(1995\cdot1997\right)^n\)<\(\left(1996\cdot1996\right)^n\)
12 tháng 11 2018
c) \(\sqrt{x-4}-\sqrt{x+11}=-3\) (đk \(x\ge4\))
\(\Leftrightarrow\sqrt{x-4}+3=\sqrt{x+11}\)
\(\Leftrightarrow\left(\sqrt{x-4}+3\right)^2=x+11\)
\(\Leftrightarrow x-4+6\sqrt{x-4}+9=x+11\)
\(\Leftrightarrow6\sqrt{x-4}=6\)
\(\Leftrightarrow\sqrt{x-4}=1\)
\(\Leftrightarrow x-4=1\)
\(\Leftrightarrow x=5\)
7 tháng 11 2018
a) đặc : \(x^2=t\left(t\ge0\right)\)
\(\Rightarrow pt\Leftrightarrow t^2+\sqrt{t+1995}=1995\)
\(\Leftrightarrow\sqrt{t+1995}=1995-t^2\)
\(\Leftrightarrow t^4-3990t^2-t+1995.1994\)
\(\Leftrightarrow t^4+t^3-1994t^2-t^3-t^2+1994t-1995t^2-1995t+1995.1994=0\)
\(\Leftrightarrow t^2\left(t^2+t-1994\right)-t\left(t+t-1994\right)-1995\left(t^2+t-1994\right)=0\)
\(\Leftrightarrow\left(t^2-t-1995\right)\left(t^2+t-1994\right)=0\)
===> ...
câu b và c tương tự mấy câu bên kia nha
7 tháng 11 2018
a) đặc : \(x^2=t\left(t\ge0\right)\)
\(\Rightarrow pt\Leftrightarrow t^2+\sqrt{t+1995}=1995\)
\(\Leftrightarrow\sqrt{t+1995}=1995-t^2\)
\(\Leftrightarrow t^4-3990t^2-t+1995.1994\)
\(\Leftrightarrow t^4+t^3-1994t^2-t^3-t^2+1994t-1995t^2-1995t+1995.1994=0\)
\(\Leftrightarrow t^2\left(t^2+t-1994\right)-t\left(t+t-1994\right)-1995\left(t^2+t-1994\right)=0\)
\(\Leftrightarrow\left(t^2-t-1995\right)\left(t^2+t-1994\right)=0\)
===> ...
câu b và c tương tự mấy câu bên kia nha
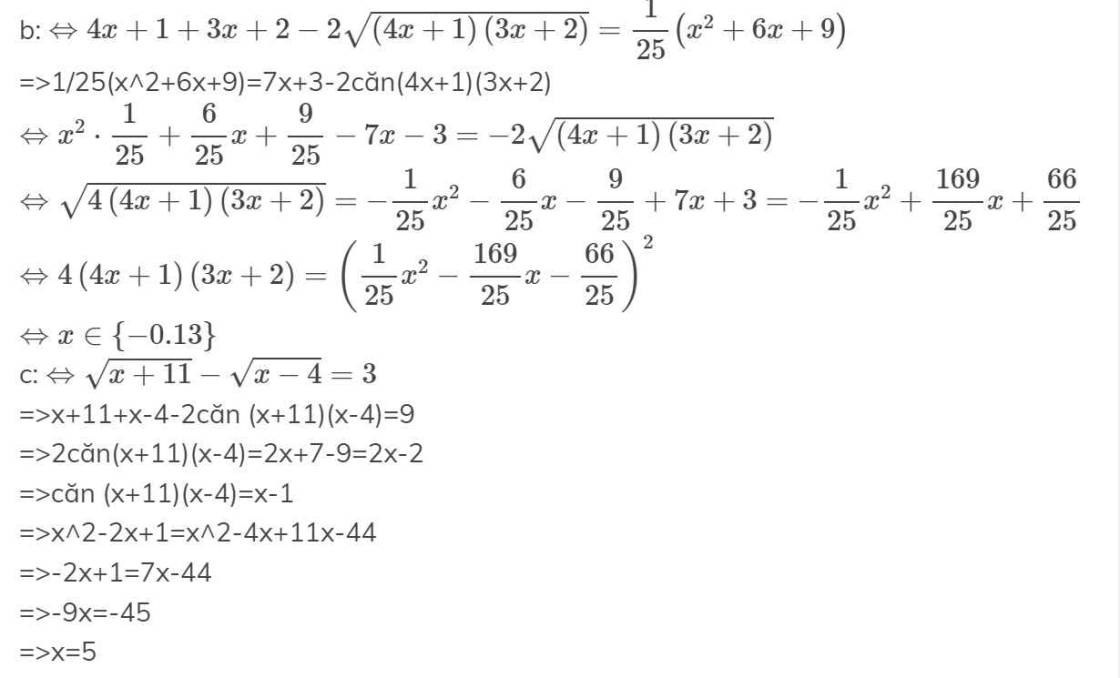
Ta có :1996! = 1.2.3 . ... . 1995 . 1996
: 1995! = 1.2.3 . ... . 1995
=> 1996! > 1995 !
=> \(\sqrt[1995]{1996}>\sqrt[1995]{1995!}\)
Ban Shadow oi, ban thieu so 1 o B roi nhe