

Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



a) (x3 – 7x + 3 – x2) : (x – 3)

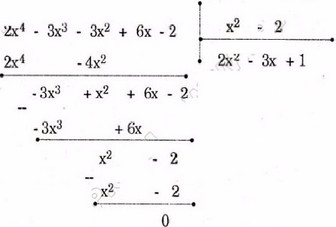
b) (2x4 – 3x2 – 3x2 – 2 + 6x) : (x2 – 2)
Xem thêm tại: http://loigiaihay.com/bai-67-trang-31-sgk-toan-8-tap-1-c43a4815.html#ixzz4ensEy1dY

a,A(\(x\)) = 13\(x^4\) + 3\(x^2\) + 15\(x\) - 8\(x\) - 7 - 7\(x\) + 7\(x^2\) - 10\(x^4\)
A(\(x\)) = (13\(x^4\) - 10\(x^4\)) + (3\(x^2\) + 7\(x^2\)) + (15\(x\) - 8\(x\) - 7\(x\)) - 7
A(\(x\)) = 3\(x^4\) + 10\(x^2\) + 0 - 7
A(\(x\)) = 3\(x^4\) + 10\(x^2\) - 7
B(\(x\)) = -4\(x^4\) - 10\(x^2\) + 10 + 5\(x^4\) - 3\(x\) - 18 + 30 - 5\(x^2\)
B(\(x\)) = (-4\(x^4\) + 5\(x^4\)) - (10\(x^2\) + 5\(x^2\)) - 3\(x\) + (10 + 30 - 18)
B(\(x\)) = \(x^4\) - 15\(x^2\) - 3\(x\) + 22
b,C(\(x\)) = A(\(x\)) + B(\(x\)) = 3\(x^4\) + 10\(x^2\) - 7 + \(x^4\) - 15\(x^2\) - 3\(x\) + 22
C(\(x\)) = 4\(x^4\) - (15\(x^2\) - 10\(x^2\)) - 3\(x\) + 22
C(\(x\)) = 4\(x^4\) - 5\(x^2\) - 3\(x\) + 15
c, D(\(x\)) = B(\(x\)) - A(\(x\)) = \(x^4\) - 15\(x^2\) - 3\(x\) + 22 - 3\(x^4\) - 10\(x^2\) + 7
D(\(x\)) = (\(x^4\) - 3\(x^4\)) - (15\(x^2\) + 10\(x^2\)) + (22 + 7)
D(\(x\)) = - 2\(x^4\) - 25\(x^2\) + 29
d, Thay \(x\) = 1 vào C(\(x\)) ta có: C(1) = 4.14 - 5.12 -3.1 + 15 = 11 (xem lại đề bài em nhá)

a: \(=\dfrac{x^4-6x^3+12x^2-14x+3}{x^2-4x+1}\)
\(=\dfrac{x^4-4x^3+x^2-2x^3+8x^2-2x+3x^2-12x+3}{x^2-4x+1}\)
\(=x^2-2x+3\)
b: \(=\dfrac{x^5-3x^4+5x^3-x^2+3x-5}{x^2-3x+5}=x^2-1\)
c: \(=\dfrac{2x^4-5x^3+2x^2+2x-1}{x^2-x-1}\)
\(=\dfrac{2x^4-2x^3-2x^2-3x^3+3x^2+3x+x^2-x-1}{x^2-x-1}\)
\(=2x^2-3x+1\)

1, \(\left(2x^4-5x^2y^2+3xy^3\right)\left(5x^3+x^2y-y^3\right)\)
\(=10x^7-25x^5y^2+15x^4y^3+2x^6y-5x^4y^3+5x^2y^5+3xy^6\)
2, a, \(4-2x+5x^2-4x^2\&5x-3+x^2\)
Sắp xếp: \(4-2x+5x^2-4x^2=5x^2-4x^2-2x+4=x^2-2x+4\)
\(5x-3+x^2=x^2+5x-3\)
- \(\left(x^2-2x+4\right)\left(x^2+5x-3\right)=x^4+3x^3-9x^2-14x-12\)
b, Làm tương tự câu a
1 ) \(\left(2x^4-5x^2y^2+3xy^3\right)\left(5x^3+x^2y-y^3\right)\)
\(=2x^4\left(5x^3+x^2y-y^3\right)-5x^2y^2\left(5x^3+x^2y-y^3\right)+3xy^3\left(5x^3+x^2y-y^3\right)\)\(=10x^7+2x^6y-2x^4y-25x^5y^2-5x^4y^3+5x^2y^5+15x^4y^3+3x^3y^4-3xy^6\)2 ) a ) \(4-2x+5x^2-4x^2=x^2-2x+4\)
\(5x-3+x^2=x^2+5x-3\)
\(\left(x^2-2x+4\right)\left(x^2+5x-3\right)\)
\(=x^4-2x^3+4x^2+5x^3-10x^2+20x-3x^2+6x-12\)
\(=x^4+3x^3-9x^2+26x-12\)
b ) \(10-x^4+3x-4x^2=-x^4-4x^2+3x+10\)
\(2x+x^3-1=x^3+2x-1\)
\(\left(-x^4-4x^2+3x+10\right)\left(x^3+2x-1\right)\)
\(=-x^4\left(x^3+2x-1\right)-4x^2\left(x^3+2x-1\right)+3x\left(x^3+2x-1\right)+10\left(x^3+2x-1\right)\)\(=-x^7-2x^5+x^4-4x^5-8x^3+4x^2+3x^4+6x^2-3x+10x^3+20x-10\)\(=-x^7-\left(2x^5+4x^5\right)+\left(3x^4+x^4\right)+\left(10x^3-8x^3\right)+\left(4x^2+6x^2\right)+\left(20x-3x\right)-10\)\(=-x^7-6x^5+4x^4+2x^3+10x^2+17x-10\)

a: \(P\left(x\right)=-2x^5+x^4+3x^3-x-\dfrac{1}{2}\)
\(Q\left(x\right)=\dfrac{-2}{3}x^5-x^4-2x^3+\dfrac{1}{2}x\)
b: \(P\left(x\right)+Q\left(x\right)=-2x^5+x^4+3x^3-x-\dfrac{1}{2}-\dfrac{2}{3}x^5-x^4-2x^3+\dfrac{1}{2}x\)
\(=\dfrac{-8}{3}x^5+x^3-\dfrac{1}{2}x-\dfrac{1}{2}\)
b: \(P\left(x\right)-Q\left(x\right)=-2x^5+x^4+3x^3-x-\dfrac{1}{2}+\dfrac{2}{3}x^5+x^4+2x^3-\dfrac{1}{2}x\)
\(=-\dfrac{4}{3}x^5+2x^4+5x^3-\dfrac{3}{2}x-\dfrac{1}{2}\)

Ta có: \(P\left(x\right)=x^2+5x^4-3x^3+x^2+4x^4+3x^3-x+5\)
\(=9x^4+2x^2-x+5\)
Ta có: \(Q\left(x\right)=x-5x^3-x^2-x^4+4x^3-x^2-3x-1\)
\(=-x^4-x^3-2x^2-2x-1\)
Ta có: P(x)+Q(x)
\(=9x^4+2x^2-x+5-x^4-x^3-2x^2-2x-1\)
\(=8x^4-x^3-3x+4\)
Ta có: P(x)-Q(x)
\(=9x^4+2x^2-x+5+x^4+x^3+2x^2+2x+1\)
\(=10x^4+x^3+4x^2+x+6\)