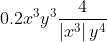Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
a/ \(\frac{y}{x}.\left(\sqrt{\frac{x^2}{y^4}}\right)=\frac{y}{x}.\frac{x}{y^2}=\frac{1}{y}\)
b/ \(2y^2.\sqrt{\frac{x^4}{4y^2}}=2y^2.\sqrt{\frac{\left(x^2\right)^2}{\left(-2y\right)^2}}=2y^2.\frac{x^2}{-2y}=-y.x^2\)
c/ \(5xy.\sqrt{\frac{25x^2}{y^6}}=5xy.\sqrt{\frac{\left(-5x\right)^2}{\left(y^3\right)^2}}=5xy.\frac{-5x}{y^3}=\frac{-25x^2}{y^2}\)
d/\(0,2.x^3y^3.\sqrt{\frac{4^2}{\left(x^2y^4\right)^2}}=\frac{1}{5}.x^3y^3.\frac{4}{x^2y^4}=\frac{4x}{5y}\)
Trần Việt Linh sai phần b,c,d r bn
Sửa lại:
b) 2y\(^2\).\(\sqrt{\frac{x^4}{4y^2}}\) với y<0
Ta có : 2y\(^2\).\(\sqrt{\frac{x^4}{4y^2}}\)=2y\(^2\).\(\frac{x^2}{\left|y\right|}\)
Vì y>0 nên |y| = -y.Ta có : 2y\(^2\).\(\frac{x^2}{2\left|y\right|}\)= -2y\(^2\).\(\frac{x^2}{2y}\) = -2x\(^2\)y
c) 5xy.\(\sqrt{\frac{25x^2}{y^6}}\) với x<0,y>0
Ta có :5xy\(\sqrt{\frac{25x^2}{y^6}}\)=5xy.\(\frac{5\left|x\right|}{y^3}\) ( y>0)
Vì x<0 nên |x| =-x .Ta có : 5xy.\(\frac{5\left|x\right|}{y^3}\)= -5xy.\(\frac{5x}{y^3}\) =\(\frac{-25x^2}{y^2}\)
d) 0,,2x\(^3\)y\(^3\).\(\sqrt{\frac{16}{x^4y^8}}\) với x#o,y#0
Ta có: 0,2x\(^3\)y\(^3\)\(\frac{4}{x^2y^4}\)=\(\frac{0,8x}{y}\) ( vì #0,y#0)
a. Ta có:\(\frac{x}{y}\sqrt{\frac{y^2}{x^4}=}\) \(\frac{x}{y}.\frac{\left|y\right|}{x^2}=\frac{x.y}{x^2y}\)\(=\frac{1}{x}\)(Vì \(x\ne0;y>0\))
b \(3x^2\sqrt{\frac{8}{x^2}}=3x^2\frac{2\sqrt{2}}{\left|x\right|}=\frac{6x^2\sqrt{2}}{-x}=-6x\sqrt{2}\)( Vì \(x< 0\))
\(a,\dfrac{x+2\sqrt{x}-3}{\sqrt{x}-1}\)
\(\Leftrightarrow\dfrac{x+3\sqrt{x}-\sqrt{x}-3}{\sqrt{x}-1}\)
\(\Leftrightarrow\dfrac{\sqrt{x}.\left(\sqrt{x}+3\right)-\left(\sqrt{x}+3\right)}{\sqrt{x}-1}\)
\(\Leftrightarrow\dfrac{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}{\sqrt{x}-1}\)
\(\Rightarrow\sqrt{x}+3\)
\(b,\dfrac{4y+3\sqrt{y}-7}{4\sqrt{y}+7}\)
\(\Leftrightarrow\dfrac{4y+7\sqrt{y}-4\sqrt{y}-7}{4\sqrt{y}+7}\)
\(\Leftrightarrow\dfrac{\sqrt{y}.\left(4\sqrt{y}\right)-\left(4\sqrt{y}+7\right)}{4\sqrt{y}+7}\)
\(\Leftrightarrow\dfrac{\left(4\sqrt{y}+7\right).\left(\sqrt{y}-1\right)}{4\sqrt{y}+7}\)
\(\Rightarrow\sqrt{y}-1\)
\(c,\dfrac{x\sqrt{y}-y\sqrt{x}}{\sqrt{x}-\sqrt{y}}\)
\(\Leftrightarrow\dfrac{\sqrt{xy}.\left(\sqrt{x}-\sqrt{y}\right)}{\sqrt{x}-\sqrt{y}}\)
\(\Rightarrow\sqrt{xy}\)
\(d,\dfrac{x-3\sqrt{x}-4}{x-\sqrt{x}-12}\)
\(\Leftrightarrow\dfrac{x+\sqrt{x}-4\sqrt{x}-4}{x+3\sqrt{x}-4\sqrt{x}-12}\)
\(\Leftrightarrow\dfrac{\sqrt{x}.\left(\sqrt{x}+1\right)-4\left(\sqrt{x}+1\right)}{\sqrt{x}.\left(x+3\right)-4\left(\sqrt{x}+3\right)}\)
\(\Leftrightarrow\dfrac{\left(\sqrt{x}+1\right).\left(\sqrt{x}-4\right)}{\left(\sqrt{x}+3\right).\left(\sqrt{x}-4\right)}\)
\(\Leftrightarrow\dfrac{\sqrt{x}+1}{\sqrt{x}+3}\)
\(\Rightarrow\dfrac{x-2\sqrt{x}-3}{x-9}\)
\(e,\dfrac{1+\sqrt{x}+\sqrt{y}+\sqrt{xy}}{1+\sqrt{4}}\)
\(\Leftrightarrow\dfrac{1+\sqrt{x}+\sqrt{y}+\sqrt{xy}}{1+2}\)
\(\Rightarrow\dfrac{1+\sqrt{x}+\sqrt{y}+\sqrt{xy}}{3}\)
Bài 1:
a. ta có \(\dfrac{x\sqrt{x}+y\sqrt{y}}{\sqrt{x}+\sqrt{y}}-\left(\sqrt{x}-\sqrt{y}\right)^2\)
= \(\dfrac{\left(\sqrt{x}+\sqrt{y}\right)\left(x-\sqrt{xy}+y\right)}{\sqrt{x}+\sqrt{y}}-x+2\sqrt{xy}-y\)
= \(x-\sqrt{xy}+y-x+2\sqrt{xy}-y\)
=\(\sqrt{xy}\)
b.ĐK: x ≠ 1
Ta có: A= \(\sqrt{\dfrac{x+2\sqrt{x}+1}{x-2\sqrt{x}+1}}\)=\(\sqrt{\dfrac{\left(\sqrt{x}+1\right)^2}{\left(\sqrt{x}-1\right)^2}}\)=\(\dfrac{\sqrt{x}+1}{\left|\sqrt{x}-1\right|}\)
*Nếu \(\sqrt{x}-1\ge0\Rightarrow\sqrt{x}\ge1\)
⇒ A = \(\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\)
*Nếu \(\sqrt{x}-1< 0\Rightarrow\sqrt{x}< 1\)
⇒ A=\(\dfrac{\sqrt{x}+1}{-\sqrt{x}+1}\)
c.Ta có:
a: \(A=6-3\sqrt{3}+4+\sqrt{3}+2\sqrt{3}=10\)
b: \(B=\sqrt{x}-\sqrt{y}-\sqrt{x}-\sqrt{y}=-2\sqrt{y}\)
c: \(C=\dfrac{\sqrt{3}-1}{\sqrt{6}-\sqrt{2}}=\dfrac{1}{\sqrt{2}}=\dfrac{\sqrt{2}}{2}\)
a) \(\dfrac{\sqrt{16a^4b^6}}{\sqrt{128a^6b^6}}\)
\(=\dfrac{4a^2b^3}{8\sqrt{2}a^3b^3}\)
\(=\dfrac{1}{2\sqrt{2}a}\)
\(=\dfrac{\sqrt{2}}{4a}\)
b) \(\sqrt{\dfrac{x-2\sqrt{x}+1}{x+2\sqrt{x}+1}}\)
chịu đấy :v
c) \(\sqrt{\dfrac{\left(x-2\right)^2}{\left(3-x\right)^2}}+\dfrac{x^2-1}{x-3}\)
\(=\dfrac{x-2}{3-x}+\dfrac{x^2-1}{x-3}\)
\(=\dfrac{x-2}{-\left(x-3\right)}+\dfrac{x^2-1}{x-3}\)
\(=-\dfrac{x-2}{x-3}+\dfrac{x^2-1}{x-3}\)
\(=\dfrac{-\left(x-2\right)+x^2-1}{x-3}\)
\(=\dfrac{-x+1+x^2}{x-3}\)
d) \(\dfrac{x-1}{\sqrt{y}-1}\cdot\sqrt{\dfrac{\left(y-2\sqrt{y}+1^2\right)}{\left(x-1\right)^4}}\)
\(=\dfrac{x-1}{\sqrt{y}-1}\cdot\sqrt{\dfrac{y-2\sqrt{y}+1}{\left(x-1\right)^4}}\)
\(=\dfrac{x-1}{\sqrt{y}-1}\cdot\dfrac{\sqrt{y-2\sqrt{y}+1}}{\left(x-1\right)^2}\)
\(=\dfrac{1}{\sqrt{y}-1}\cdot\dfrac{\sqrt{y-2\sqrt{y}+1}}{x-1}\)
\(=\dfrac{\sqrt{y-2\sqrt{y}+1}}{\left(\sqrt{y}-1\right)\left(x-1\right)}\)
\(=\dfrac{\sqrt{y-2\sqrt{y}+1}}{x\sqrt{y}-\sqrt{y}-x+1}\)
e) \(4x-\sqrt{8}+\dfrac{\sqrt{x^3+2x^2}}{\sqrt{x+2}}\)
\(=4x-2\sqrt{2}+\dfrac{\sqrt{x^2\cdot\left(x+2\right)}}{\sqrt{x+2}}\)
\(=4x-2\sqrt{2}+\sqrt{x^2}\)
\(=4x-2\sqrt{x}+x\)
\(=5x-2\sqrt{2}\)
\(a.\sqrt{2a}.\sqrt{18a}=\sqrt{2a}.3\sqrt{2a}=3.2a=6a\)
\(b.\sqrt{3a.27ab^2}=\sqrt{9a^2b^2.9}=9\text{ |}ab\text{ |}\)
\(c.2y^2.\sqrt{\dfrac{x^4}{4y^2}}=2y^2.\dfrac{x^2}{-2y}=-x^2y\)
\(d.\dfrac{y}{x}.\sqrt{\dfrac{x^2}{y^4}}=\dfrac{y}{x}.\dfrac{x}{y^2}=\dfrac{1}{y}\)
\(e.\sqrt{\dfrac{9a^2}{16}}=\dfrac{3\text{ |}a\text{ |}}{4}\)
\(f.\sqrt{10.16a^2}=-4a\sqrt{10}\)
\(g.\sqrt{a^4\left(3-a\right)^2}=a^2\left(a-3\right)\)
\(h.\sqrt{\dfrac{2a^2b^4}{98}}\sqrt{\dfrac{a^2b^4}{49}}=\dfrac{b^2\text{ |}a\text{ |}}{7}\)
a. \(\sqrt{\dfrac{63y^3}{7y}}\)=\(\sqrt{9y^2}\)=3y
b.\(\sqrt{\dfrac{48x^3}{3x^5}}\)=\(\sqrt{16\cdot\dfrac{1}{X^2}}\)= \(\sqrt{16}\cdot\sqrt{\dfrac{1}{X^2}}\)=\(4\cdot\dfrac{1}{X}=\dfrac{4}{X}\)
c.\(\sqrt{\dfrac{45mn^2}{20m}}=\sqrt{\dfrac{9n^2}{4}}=\dfrac{\sqrt{9n^2}}{\sqrt{4}}=\dfrac{3n}{2}\)
d. \(\sqrt{\dfrac{16a^4b^6}{128a^6b^6}}=\sqrt{\dfrac{1}{8a^2}}=\dfrac{1}{2\sqrt{2}a}\)
a) \(\dfrac{\sqrt{63y^3}}{\sqrt{7y}}=\sqrt{\dfrac{63y^3}{7y}}=\sqrt{9y^2}=3y\)
b) \(\dfrac{\sqrt{48x^3}}{\sqrt{3x^5}}=\sqrt{\dfrac{48x^3}{3x^5}}=\sqrt{\dfrac{16}{x^2}}=\dfrac{4}{x}\)
c) \(\dfrac{\sqrt{45mn^2}}{\sqrt{20m}}=\sqrt{\dfrac{45mn^2}{20m}}=\sqrt{\dfrac{9n^2}{4}}=\dfrac{3n}{2}\)
d) \(\dfrac{\sqrt{16a^4b^6}}{\sqrt{128a^6b^6}}=\sqrt{\dfrac{16a^4b^6}{128a^6b^6}}=\sqrt{\dfrac{1}{8a^2}}=\dfrac{1}{2\left|a\right|\sqrt{2}}=\dfrac{-1}{2a\sqrt{2}}\)
a)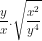
= 
.
= 
.
= 
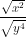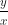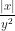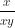vì x > 0.
Do đó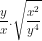
= 
.
b)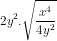
= 
.
= 
.
.
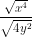Vì y < 0 nên │y│= -y. Do đó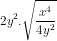
= 
.
= 
.
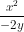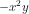c) 5xy.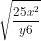
= 5xy.
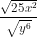= 5xy.
.
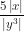Vì x < 0, y > 0 nên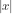
= -x và 
= 
.
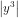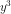Do đó: 5xy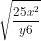
= 5xy.
= -
.
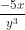d) 0,2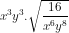
= 
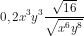= 0,2
= 
Nếu x > 0 thì
> 0 nên 
. Do đó 0,2
= 
.
Nếu x < 0 thì
< 0 nên 
. Do đó 0,2
= -
.