Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a,\(\left(\sqrt{6}-\sqrt{10}\right)\sqrt{4+\sqrt{15}}=\sqrt{6}.\sqrt{4-\sqrt{15}}-\sqrt{10}.\sqrt{4+\sqrt{15}}\)
=\(\sqrt{24+6\sqrt{15}}-\sqrt{40+10\sqrt{15}}=\sqrt{\left(\sqrt{15}+3\right)^2}-\sqrt{\left(\sqrt{15}+5\right)^2}\)
=\(\sqrt{15}+3-\sqrt{15}-5=-2\)
b,\(\left(\sqrt{3}+\sqrt{30}\right)\sqrt{10-\sqrt{41-4\sqrt{10}}}\)
=\(\sqrt{3}\left(1+\sqrt{10}\right)\sqrt{10-\sqrt{40-2\sqrt{40}+1}}\)
=\(\sqrt{3}\left(1+\sqrt{10}\right)\sqrt{10-\sqrt{\left(\sqrt{40}-1\right)^2}}\)
=\(\sqrt{3}\left(1+\sqrt{10}\right)\sqrt{10-\sqrt{40}+1}\)
=\(\sqrt{3}\left(1+\sqrt{10}\right)\sqrt{11-2\sqrt{10}}=\sqrt{3}\left(1+\sqrt{10}\right)\sqrt{\left(\sqrt{10}-1\right)^2}\)
=\(\sqrt{3}\left(1+\sqrt{10}\right)\left(\sqrt{10}-1\right)=9\sqrt{3}\)
2,\(A=\left(\frac{\sqrt{a}\left(\sqrt{a}+1\right)-a-2}{\sqrt{a}+1}\right):\left(\frac{\sqrt{a}\left(1-\sqrt{a}\right)-\sqrt{a}+4}{1-a}\right)\)
\(A=\left(\frac{a+\sqrt{a}-a-2}{\sqrt{a}+1}\right):\left(\frac{\sqrt{a}-a-\sqrt{a}+4}{1-a}\right)=\left(\frac{\sqrt{a}+2}{\sqrt{a}+1}\right).\left(\frac{1-a}{4-a}\right)\)
\(A=\frac{\sqrt{a}-2}{\sqrt{a}+1}.\frac{a-1}{a-4}=\frac{\sqrt{a}-1}{\sqrt{a}+2}\)
b, ̣để \(A=\frac{1}{2}\Rightarrow\frac{\sqrt{a}-1}{\sqrt{a}+2}=\frac{1}{2}\Leftrightarrow2\sqrt{a}-2=\sqrt{a}+2\Leftrightarrow\sqrt{a}=4\Leftrightarrow a=16\left(t.m\right)\)
Bạn oi bài 2 hàng A thú 2 phải là \(\frac{\sqrt{a}-2}{\sqrt{a}+1}\) mình nhầm

a. \(\sqrt{4\left(a-3\right)^2}=2.|a-3|=2\left(a-3\right)\) (vì a \(\ge3\) nên a-3\(\ge\) 0. Do đó: \(|a-3|=a-3\))
b. \(\sqrt{9\left(b-2\right)^2}=3.|b-2|=3\left(2-b\right)\) (vì b < 2 nên b-2 < 0. Do đó : \(|b-2|=2-b\))
c. \(\sqrt{a^2\left(a+1\right)^2}=a\left(a+1\right)\) ( vì a > 0)
d. \(\sqrt{b^2\left(b-1\right)^2}=b\left(b-1\right)\) (vì b < 0)

a) \(\frac{b-16}{4-\sqrt{b}}\left(b\ge0,b\ne16\right)\)
\(=\frac{\left(\sqrt{b}-4\right)\left(\sqrt{b}+4\right)}{4-\sqrt{b}}\)
\(=-\sqrt{b}-4\)
b) \(\frac{a-4\sqrt{a}+4}{a-4}\left(a\ge0;a\ne4\right)\)
\(=\frac{a-2.\sqrt{a}.2+4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}\)
\(=\frac{\left(\sqrt{a}-2\right)^2}{\left(\sqrt{a}+2\right)\left(\sqrt{a}-2\right)}=\frac{\sqrt{a}-2}{\sqrt{a}+2}\)
c) \(2x+\sqrt{1+4x^2-4x}\) với \(x\le\frac{1}{2}\)
\(=2x+\sqrt{\left(1-2x\right)^2}\)
\(=2x+\left|1-2x\right|=2x+1-2x=1\)
d) \(\frac{4a-4b}{\sqrt{a}-\sqrt{b}}\left(a,b\ge0;a\ne b\right)\)
\(=\frac{4\left(a-b\right)}{\sqrt{a}-\sqrt{b}}=\frac{4\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}{\sqrt{a}-\sqrt{b}}\)
\(=4\left(\sqrt{a}+\sqrt{b}\right)\)

a) \(\sqrt{\left(2x-6\right)^2}=\left|2x-6\right|=2x-6\)
b) \(\sqrt{\left(x-4\right)^2}=\left|x-4\right|=4-x\)
a) Ta có: \(\sqrt{\left(2x-6\right)^2}\)
\(=\sqrt{4\left(x-3\right)^2}\)
\(=2\left(x-3\right)=2x-6\) (vì \(x\ge3\))
b) Ta có: \(\sqrt{\left(x-4\right)^2}\)
\(=4-x\) (vì x<4)

a) =
= 0,6.│a│
Vì a < 0 nên │a│= -a. Do đó = -0,6a.
b) =
.
= │
│.│3 - a│.
Vì ≥ 0 nên │b│=
. Vì a ≥ 3 nên 3 - a ≤ 0, do đó │3 - a│= a - 3.
Vậy =
(a - 3).
c) =
=
= √81.√16.
= 9.4.│1 - a│
Vì a > 1 nên 1 - a < 0. Do đó │1 - a│= a -1.
Vậy = 36(a - 1).
d) :
=
: (
=
: (
.│a - b│)
Vì a > b nên a -b > 0, do đó│a - b│= a - b.
Vậy :
=
: (
(a - b)) =
.
a) =
= 0,6.│a│
Vì a < 0 nên │a│= -a. Do đó = -0,6a.
b) =
.
= │
│.│3 - a│.
Vì ≥ 0 nên │b│=
. Vì a ≥ 3 nên 3 - a ≤ 0, do đó │3 - a│= a - 3.
Vậy =
(a - 3).
c) =
=
= √81.√16.
= 9.4.│1 - a│
Vì a > 1 nên 1 - a < 0. Do đó │1 - a│= a -1.
Vậy = 36(a - 1).
d) :
=
: (
=
: (
.│a - b│)
Vì a > b nên a -b > 0, do đó│a - b│= a - b.
Vậy :
=
: (
(a - b)) =
.

1) \(\frac{9}{x^2}+\frac{2x}{\sqrt{2x^2+9}}=1\left(ĐK:x\ne0\right)\)
Đặt: \(\sqrt{2x^2+9}=a\left(a\ge0\right)\)
\(\Leftrightarrow2x^2+9=a^2\Leftrightarrow9=a^2-2a^2\)
Khi đó pt đã cgo trở rhanhf:
\(\frac{a^2-2x^2}{x^2}+\frac{2x}{a}=1\)
\(\Leftrightarrow\left(\frac{a}{x}\right)^2-2+\frac{2x}{a}-1=0\)
\(\Leftrightarrow\left(\frac{a}{x}\right)^2+\frac{2x}{a}-3=0\) (*)
Đặt: \(\frac{a}{x}=b\) khi đó (*) trở thành:
\(b^2+\frac{2}{b}-3=0\)
\(\Leftrightarrow b^3+2-3b=0\)
\(\Leftrightarrow\left(b^3-b\right)-\left(2b-2\right)=0\)
\(\Leftrightarrow b\left(b-1\right)\left(b+1\right)-2\left(b-1\right)=0\)
\(\Leftrightarrow\left(b-1\right)\left(b^2+b-2\right)=0\)
\(\Leftrightarrow\left(b-1\right)^2\left(b+2\right)=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}b-1=0\\b+2=0\end{array}\right.\)\(\Leftrightarrow\left[\begin{array}{nghiempt}b=1\\b=-2\end{array}\right.\)
Với: \(b=1\) ta có:
\(\frac{a}{x}=1\Leftrightarrow a=x\Leftrightarrow\sqrt{2x^2+9}=x\Leftrightarrow2x^2+9=x^2\Leftrightarrow x^2+9=0\left(loai\right)\)
Với: \(b=-2\) ta có:
\(\frac{a}{x}=-2\)
\(\Leftrightarrow a=-2x\)
\(\Leftrightarrow\sqrt{2x^2+9}=-2x\)
\(\Leftrightarrow2x^2+9=4x^2\)
\(\Leftrightarrow2x^2=9\)
\(\Leftrightarrow x^2=\frac{9}{2}\Leftrightarrow\left[\begin{array}{nghiempt}x=\frac{3}{\sqrt{2}}\\x=-\frac{3}{\sqrt{2}}\end{array}\right.\)
Thử lại ta thấy: \(x=\frac{3}{\sqrt{2}}\left(ktm\right);x=-\frac{3}{\sqrt{x}}\left(tm\right)\)
Vaayk pt đã cho có nhgieemj là \(x=-\frac{3}{\sqrt{2}}\)
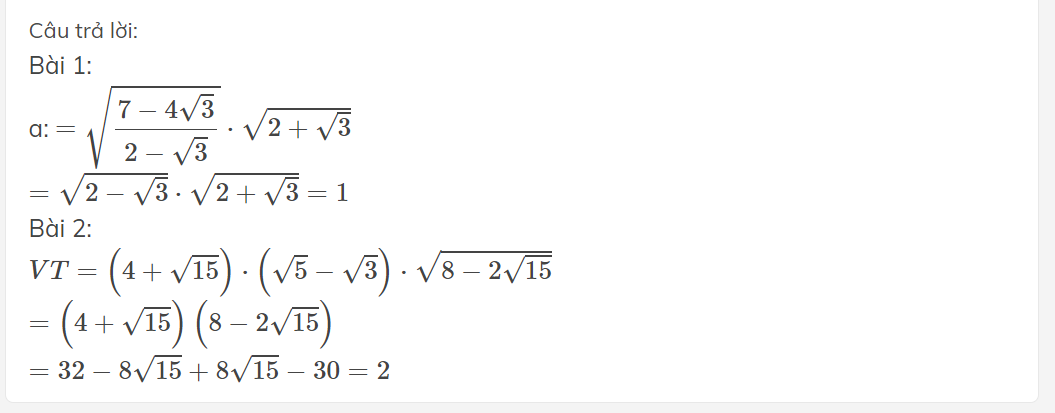


\(1,\sqrt{4\left(a-4\right)^2}\left(dkxd:a\ge4\right)\)
\(=\sqrt{4}.\sqrt{\left(a-4\right)^2}\)
\(=\sqrt{2^2}.\left|a-4\right|\)
\(=2\left(a-4\right)\)
\(=2a-8\)
\(2,\sqrt{9\left(b-5\right)^2}\left(dkxd:b< 5\right)\)
\(=\sqrt{9}.\sqrt{\left(b-5\right)^2}\)
\(=\sqrt{3^2}.\left|b-5\right|\)
\(=3\left(-b+5\right)\)
\(=-3b+15\)
Thế -b+5 khác 5-b à
Ngô Hải Nam