Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

P(x) = 3x2 – 5 + x4 – 3x3 – x6 – 2x2 – x3
= – x6 + x4 + (– 3x3 – x3) + (3x2 – 2x2) – 5
= – x6 + x4 – 4x3 + x2 – 5.
= – 5+ x2 – 4x3 + x4 – x6
Và Q(x) = x3 + 2x5 – x4 + x2 – 2x3 + x –1
= 2x5 – x4 + (x3 – 2x3) + x2 + x –1
= 2x5 – x4 – x3 + x2 + x –1.
= –1+ x + x2 – x3 – x4 + 2x5

Ta đặt và thực hiện phép tính P(x) + Q(x) và P(x) – Q(x) có
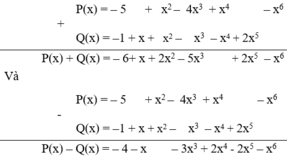
Vậy: P(x) + Q(x) = – 6 + x + 2x2 – 5x3 + 2x5 – x6
P(x) – Q(x) = – 4 – x – 3x3 + 2x4 - 2x5 – x6

Trả lời câu hỏi của tôi đi. Tí tôi trả lời của bạn chings xác 100% luôn. UY TÍN BẠN NHÉ

a) \(P\left(x\right)=3x^3-x^2-2x^4+3+2x^3+x+3x^4-x^2-2x^4+3+2x^3+x+3x^4\)
\(=2x^4+7x^3-2x^2+2x+6\)
\(Q\left(x\right)=-x^4+x^2-4x^3-2+2x^2-x-x^3-x^4+x^2-4x^3-2+2x^2-x-x^3\)
\(=-2x^4-10x^3+6x^2-2x-4\)
b) \(P\left(x\right)+Q\left(x\right)=2x^4+7x^3-2x^2+2x+6-2x^4-10x^3+6x^2-2x-4\)
\(=-3x^3+4x^2+2\)

a) \(...=P\left(x\right)=2x^4-x^4+3x^3+4x^2-3x^2+3x-x+3\)
\(P\left(x\right)=x^4+3x^3+x^2+2x+3\)
\(...=Q\left(x\right)=x^4+x^3+3x^2-x^2+4x+4-2\)
\(Q\left(x\right)=x^4+x^3+2x^2+4x+2\)
b) \(P\left(x\right)+Q\left(x\right)=\left(x^4+3x^3+x^2+2x+3\right)+\left(x^4+x^3+2x^2+4x+2\right)\)
\(\Rightarrow P\left(x\right)+Q\left(x\right)=2x^4+4x^3+3x^2+6x+5\)
\(P\left(x\right)-Q\left(x\right)=\left(x^4+3x^3+x^2+2x+3\right)-\left(x^4+x^3+2x^2+4x+2\right)\)
\(\)\(\Rightarrow P\left(x\right)-Q\left(x\right)=x^4+3x^3+x^2+2x+3-x^4-x^3-2x^2-4x-2\)
\(\Rightarrow P\left(x\right)-Q\left(x\right)=2x^3-x^2-2x+1\)

a: P(x)=2x^3-x^2+3x+20
Q(x)=-x^3-x^2-3x-4
b: K(x)=2x^3-x^2+3x+20-x^3-x^2-3x-4
=x^3-2x^2+16
H(x)=2x^3-x^2+3x+20+x^3+x^2+3x+4
=3x^3+6x+24
c: K(-2)=(-2)^3-2*(-2)^2+16=0
=>x=-2 là nghiệm của K(x)
H(-2)=3*(-2)^3+6*(-2)+24=24-12-3*8=-12<>0
=>x=-2 ko là nghiệm

a) \(M\left(x\right)=-x^6+x^4-4x^3+x^2-5\)
\(N\left(x\right)=2x^5-x^4-x^3+x^2+x-1\)
b)\(M\left(x\right)+N\left(x\right)=-x^6+x^4-4x^3+x^2-5+2x^5-x^4-x^3+x^2+x-1\) \(=-x^6+2x^5-5x^3+2x^2+x-6\)
Vậy...
\(M\left(x\right)-N\left(x\right)=-x^6+x^4-4x^3+x^2-5-\left(2x^5+x^4-x^3+x^2-x-1\right)\)\(=-x^6+x^4-4x^3+x^2-5-2x^5+x^4+x^3-x^2+x+1\) \(=-x^6-2x^5+2x^4-3x^3+x-4\) Vậy...
c) -N(x)+M(x)=.........

a. M(x) = 3x2- 5+ x4- 3x3- x6- 2x2- x3
= - 5+ (3x2- 2x2)+(-3x3- x3)+ x4- x6
= - 5 +x2+ (-4x3) +x4 -x6
N(x) = x3 + 2x5 -x4 +x2 -2x3 +x-1
= -1+x+x2+(x3- 2x3) -x4+ 2x5
= -1+ x+ x2+ (-x3) -x4+ 2x5
b. M(x) +N(x)= (- 5 +x2 -4x3 +x4 -x6) + (-1+ x+ x2 -x3 -x4+ 2x5)
= - 5 +x2 -4x3 +x4 -x6 -1+ x+ x2 -x3 -x4+ 2x5
= (-5-1) + x +( x2 +x2) +(-4x3-x3) +(x4-x4) +2x5 -x6
= -6 +x +2x2 +(-5x3) +0 +2x5-x6
= -6 +x +2x2 +(-5x3) +2x5-x6
M(x) -N(x)= (- 5 +x2 -4x3 +x4 -x6) - (-1+ x+ x2 -x3 -x4+ 2x5)
= - 5 +x2 -4x3 +x4 -x6 -1-x -x2 +x3 +x4 -2x5
= (-5-1) +x +(x2-x2) +(-4x3+x3) +(x4+x4) -2x5 -x6
= -6 +x +0 +(-3x3) +2x4 -2x5 -x6
= -6 +x +(-3x3) +2x4 -2x5 -x6
a/
\(P_{\left(x\right)}=3x^2-5+x^4-3x^3-x^6-2x^2-x^3\)
\(=\left(3x^2-2x^2\right)+\left(-3x^3-x^3\right)+x^4-x^6-5\)
\(=-x^6+x^4-4x^3+x^2-5\) (mk sắp xếp luôn ở đoạn này)
\(Q_{\left(x\right)}=x^3+2x^5-x^4+x^2-2x^3+x-1\) \(=\left(x^3-2x^3\right)+2x^5-x^4+x^2+x-1\)
\(=2x^5-x^4-x^3+x^2+x-1\)
b/ chỉ lm đc 1 cách kiểu hàng ngang thoy bn ạ, k lm theo hàng dọc đc đâu! nên bn tự lm cách hàng dọc nhé!
\(P_{\left(x\right)}+Q_{\left(x\right)}=\left(-x^6+x^4-4x^3+x^2-5\right)+\left(2x^5-x^4-x^3+x^2+x-1\right)\)
\(=-x^6+2x^5+\left(x^4-x^4\right)+\left(-4x^3-x^3\right)+\left(x^2+x^2\right)+x+\left(-5+1\right)\)
\(=-x^6+2x^5-5x^3+2x^2+x-4\)
\(P_{\left(x\right)}-Q_{\left(x\right)}\) (muộn r`, gơi ý): chuyển dấu -->giao hoán --> kết quả
@Định Quang ( Real )
@Nguyễn Nhật Minh
@Hoàng Thị Ngọc Oanh
@Hoang Hung Quan