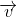Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Gọi G là giao điểm của AK, BM thì G là trọng tâm của tam giác.
Ta có  =
= 
 =>
=> 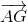 =
=

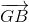 = -
= -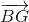 = -
= -
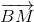 = -
= -

Theo quy tắc 3 điểm đối với tổng vec tơ:
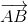 =
= 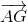 +
+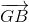 =>
=> 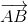 =
= 
 -
- 
 =
=  (
( -
-  ).
).
AK là trung tuyến thuộc cạnh BC nên
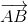 +
+ 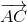 = 2
= 2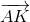 =>
=> 
 -
- 
 +
+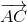 = 2
= 2
Từ đây ta có 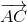 =
= 
 +
+
 =>
=> 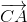 = -
= -
 -
- 
 .
.
BM là trung tuyến thuộc đỉnh B nên
 +
+ 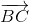 = 2
= 2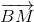 => -
=> - 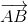 +
+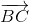 = 2
= 2
=> 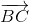 =
= 
 +
+
 .
.

A B C D I K
a)
- \(\overrightarrow{BI}=\frac{1}{2}\left(\overrightarrow{BA}+\overrightarrow{BD}\right)\) (t/c trung điểm)
\(=\frac{1}{2}\left(\overrightarrow{BA}+\frac{1}{2}\overrightarrow{BC}\right)\)
\(=\frac{1}{2}\overrightarrow{BA}+\frac{1}{4}\overrightarrow{BC}\)
- \(\overrightarrow{BK}=\overrightarrow{BA}+\overrightarrow{AK}\)
\(=\overrightarrow{BA}+\frac{1}{3}\overrightarrow{AC}\)
\(=\overrightarrow{BA}+\frac{1}{3}\left(\overrightarrow{BC}-\overrightarrow{BA}\right)\)
\(=\overrightarrow{BA}+\frac{1}{3}\overrightarrow{BC}-\frac{1}{3}\overrightarrow{BA}\)
\(=\frac{2}{3}\overrightarrow{BA}+\frac{1}{3}\overrightarrow{BC}\)
b) Ta có: \(\overrightarrow{BK}=\frac{2}{3}\overrightarrow{BA}+\frac{1}{3}\overrightarrow{BC}=\frac{4}{3}\left(\frac{1}{2}\overrightarrow{BA}+\frac{1}{4}\overrightarrow{BC}\right)=\frac{4}{3}\overrightarrow{BI}\)
=> B,K,I thẳng hàng
c) \(27\overrightarrow{MA}-8\overrightarrow{MB}=2015\overrightarrow{MC}\)
\(\Leftrightarrow27\left(\overrightarrow{MC}+\overrightarrow{CA}\right)-8\left(\overrightarrow{MC}+\overrightarrow{CB}\right)=2015\overrightarrow{MC}\)
\(\Leftrightarrow27\overrightarrow{MC}+27\overrightarrow{CA}-8\overrightarrow{MC}-8\overrightarrow{CB}-2015\overrightarrow{MC}=\overrightarrow{0}\)
\(\Leftrightarrow-1996\overrightarrow{MC}+27\overrightarrow{CA}-8\overrightarrow{CB}=\overrightarrow{0}\)
\(\Leftrightarrow1996\overrightarrow{CM}=8\overrightarrow{CB}-27\overrightarrow{CA}\)
\(\Leftrightarrow\overrightarrow{CM}=\frac{8\overrightarrow{CB}-27\overrightarrow{CA}}{1996}\)
Vậy: Dựng điểm M sao cho \(\overrightarrow{CM}=\frac{8\overrightarrow{CB}-27\overrightarrow{CA}}{1996}\)

Có \(\overrightarrow{v}=\overrightarrow{MA}+\overrightarrow{MB}-2\overrightarrow{MC}=\overrightarrow{MA}+\overrightarrow{MB}+2\overrightarrow{CM}\)
\(=\left(\overrightarrow{CM}+\overrightarrow{MA}\right)+\left(\overrightarrow{CM}+\overrightarrow{MB}\right)=\overrightarrow{CA}+\overrightarrow{CB}\) (Không phụ thuộc vào vị trí điểm M).
A B C I K
b) Dựng hình bình hành BCAD. Theo quy tắc hình bình hành:
\(\overrightarrow{CA}+\overrightarrow{CB}=\overrightarrow{CD}\).
Vậy \(\overrightarrow{CD}=\overrightarrow{v}\).
\(\overrightarrow{v}=\overrightarrow{MA}+\overrightarrow{MB}-2\overrightarrow{MC}\)
\(=2\overrightarrow{ME}-2\overrightarrow{MC}\) (E là trung điểm cạnh AB)
\(=\left(\overrightarrow{ME}-MC\right)=2\overrightarrow{CE}\)
vậy \(\overrightarrow{v}\) không phụ thuộc vị trí của điểm M
\(\overrightarrow{CD}=\overrightarrow{v}=2\overrightarrow{CE}\) thì E là trung điểm của CD
\(\Rightarrow\) ta dựng được điểm D

\(\overrightarrow{BI}=-\frac{2}{7}\overrightarrow{IC}=-\frac{2}{7}\left(\overrightarrow{BC}-\overrightarrow{BI}\right)\Rightarrow\overrightarrow{BI}=-\frac{2}{5}\overrightarrow{BC}\)
\(\Rightarrow\overrightarrow{AI}=\overrightarrow{AB}+\overrightarrow{BI}=\overrightarrow{AB}-\frac{2}{5}\overrightarrow{BC}\Rightarrow\overrightarrow{BC}=\frac{5}{2}\overrightarrow{AB}-\frac{5}{2}\overrightarrow{AI}\) (1)
\(\overrightarrow{BJ}=\frac{3}{2}\overrightarrow{IC}=-\frac{3}{7}\overrightarrow{BI}=\frac{6}{35}\overrightarrow{BC}\)
\(\overrightarrow{AJ}=\overrightarrow{AB}+\overrightarrow{BJ}=\overrightarrow{AB}+\frac{6}{35}\overrightarrow{BC}\Rightarrow\overrightarrow{BC}=\frac{35}{6}\overrightarrow{AJ}-\frac{35}{6}\overrightarrow{AB}\) (2)
Từ (1);(2) ta có:
\(\frac{5}{2}\overrightarrow{AB}-\frac{5}{2}\overrightarrow{AI}=\frac{35}{6}\overrightarrow{AJ}-\frac{35}{6}\overrightarrow{AB}\)
\(\Rightarrow\overrightarrow{AB}=...\)
Quá trình tính có thể nhầm lẫn con số và dấu, bạn kiểm tra lại


Ta có: \(\overrightarrow{MB}=3\overrightarrow{MC}\Rightarrow\overrightarrow{MB}=3\left(\overrightarrow{MB}+\overrightarrow{BC}\right)\)
\(\Rightarrow\overrightarrow{MB}=3\overrightarrow{MB}+3\overrightarrow{BC}\)
\(\Rightarrow-\overrightarrow{MB}=3\overrightarrow{BC}\)
\(\Rightarrow\overrightarrow{BM}=\dfrac{2}{3}\overrightarrow{BC}\). Mà \(\overrightarrow{BC}=\overrightarrow{AC}-\overrightarrow{AB}\) nên \(\overrightarrow{BM}=\dfrac{2}{3}\left(\overrightarrow{AC}-\overrightarrow{AB}\right)\)
Theo quy tắc 3 điểm, ta có
\(\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}\Rightarrow\overrightarrow{AM}=\overrightarrow{AB}+\dfrac{3}{2}\overrightarrow{AC}-\dfrac{3}{2}\overrightarrow{AB}\)
\(\Rightarrow\overrightarrow{AM}=-\dfrac{1}{2}\overrightarrow{AB}+\dfrac{3}{2}\overrightarrow{AC}\) hay \(\overrightarrow{AM}=-\dfrac{1}{2}\overrightarrow{u}+\dfrac{3}{2}\overrightarrow{v}\)
Trước hết ta có
=> = 3
= 3 + 3
+ 3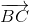
=> - = 3
= 3 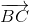
=>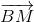 =
= 
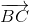
mà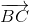 =
= 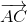 -
- 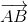 nên
nên 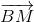 =
=  (
(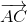 -
- 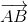 )
)
Theo quy tắc 3 điểm, ta có
=>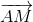 = -
= - 
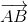 +
+
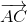 hay
hay 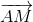 = -
= - 
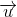 +
+