Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) Cách 1: y' = (9 -2x)'(2x3- 9x2 +1) +(9 -2x)(2x3- 9x2 +1)' = -2(2x3- 9x2 +1) +(9 -2x)(6x2 -18x) = -16x3 +108x2 -162x -2.
Cách 2: y = -4x4 +36x3 -81x2 -2x +9, do đó
y' = -16x3 +108x2 -162x -2.
b) y' = .(7x -3) +
(7x -3)'=
(7x -3) +7
.
c) y' = (x -2)'√(x2 +1) + (x -2)(√x2 +1)' = √(x2 +1) + (x -2) = √(x2 +1) + (x -2)
= √(x2 +1) +
=
.
d) y' = 2tanx.(tanx)' - (x2)' =
.
e) y' = sin
=
sin
.

1/ \(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{1+4x}.\sqrt[3]{1+6x}.\sqrt[4]{1+8x}-\sqrt[3]{1+6x}.\sqrt[4]{1+8x}}{x}+\lim\limits_{x\rightarrow0}\dfrac{\sqrt[3]{1+6x}.\sqrt[4]{1+8x}-\sqrt[3]{1+6x}}{x}+\lim\limits_{x\rightarrow0}\dfrac{\sqrt[3]{1+6x}-1}{x}\)
Liên hợp dài quá ko muốn gõ tiếp, bạn tự đặt nhân tử chung rồi liên hợp nhé, kết quả ra 5
2/ \(\lim\limits_{x\rightarrow1}\dfrac{\sqrt[3]{1+7x}-2-\left(x^3-3x+2\right)}{x-1}\)
\(=\lim\limits_{x\rightarrow1}\dfrac{\dfrac{7\left(x-1\right)}{\sqrt[3]{\left(1+7x\right)^2}+2\sqrt[3]{1+7x}+4}-\left(x-1\right)^2\left(x+2\right)}{x-1}\)
\(=\lim\limits_{x\rightarrow1}\dfrac{7}{\sqrt[3]{\left(1+7x\right)^2}+2\sqrt[3]{1+7x}+4}-\left(x-1\right)\left(x+2\right)=\dfrac{7}{12}\)
3/ \(\lim\limits_{x\rightarrow-\infty}\dfrac{x^3-x^2+1}{2x^2+3x-1}=\lim\limits_{x\rightarrow-\infty}\dfrac{x-1+\dfrac{1}{x^2}}{2+\dfrac{3}{x}-\dfrac{1}{x^2}}=-\infty\)
4/ \(\lim\limits_{x\rightarrow+\infty}\dfrac{\sqrt{x}+\sqrt[3]{x}+\sqrt[4]{x}}{\sqrt{4x+1}}=\lim\limits_{x\rightarrow+\infty}\dfrac{1+\dfrac{1}{\sqrt[6]{x}}+\dfrac{1}{\sqrt[4]{x}}}{\sqrt{4+\dfrac{1}{x}}}=\dfrac{1}{\sqrt{4}}=\dfrac{1}{2}\)
5/ \(\lim\limits_{x\rightarrow-\infty}\dfrac{x+\sqrt{x^2+2}}{\sqrt[3]{8x^3+x^2+1}}=\lim\limits_{x\rightarrow-\infty}\dfrac{1-\sqrt{1+\dfrac{2}{x^2}}}{\sqrt[3]{8+\dfrac{1}{x}+\dfrac{1}{x^3}}}=\dfrac{1-1}{\sqrt[3]{8}}=0\)
6/ \(\lim\limits_{x\rightarrow-\infty}\dfrac{\sqrt{4x^2+3x-7}}{\sqrt[3]{27x^3+5x^2+x-4}}=\lim\limits_{x\rightarrow-\infty}\dfrac{-\sqrt{4+\dfrac{3}{x}-\dfrac{7}{x^2}}}{\sqrt[3]{27+\dfrac{5}{x}+\dfrac{1}{x^2}-\dfrac{4}{x^3}}}=\dfrac{-\sqrt{4}}{\sqrt[3]{27}}=\dfrac{-2}{3}\)
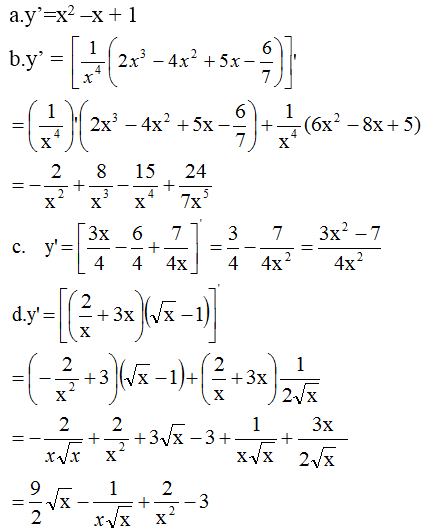
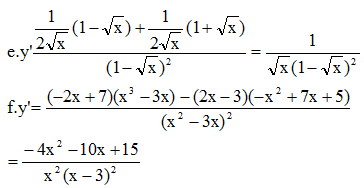
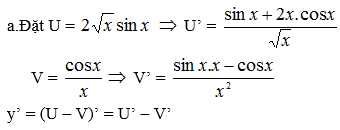
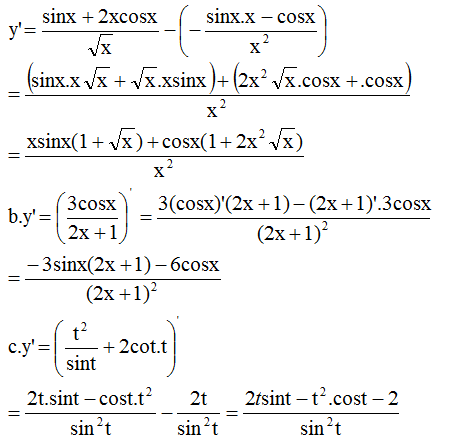
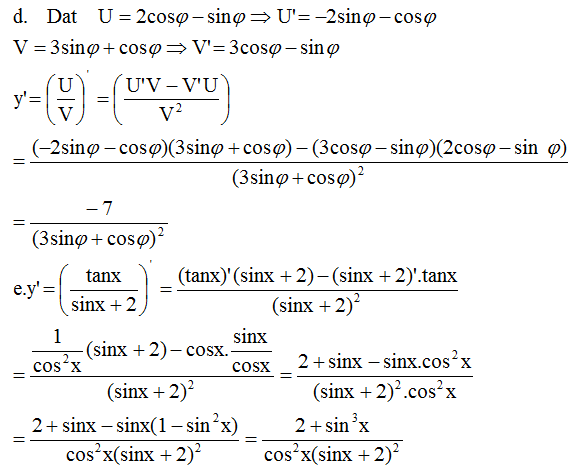
a/ \(y'=\dfrac{\left(x^3+2\sqrt{x-1}\right)'\left(x-1\right)-\left(x-1\right)'\left(x^3+2\sqrt{x-1}\right)}{\left(x-1\right)^2}\)
\(y'=\dfrac{\left(2x^2+\dfrac{1}{\sqrt{x-1}}\right)\left(x-1\right)-x^3-2\sqrt{x-1}}{\left(x-1\right)^2}=\dfrac{x^3-2x^2-\sqrt{x-1}}{\left(x-1\right)^2}\)
b/ \(y'=\dfrac{\left(4x^3+2x-3\right)'\left(\sqrt{x^2+2}\right)-\left(\sqrt{x^2+2}\right)'\left(4x^3+2x-3\right)}{x^2+2}\)
\(y'=\dfrac{\left(12x^2+2\right)\sqrt{x^2+2}-\dfrac{x}{\sqrt{x^2+2}}\left(4x^3+2x-3\right)}{x^2+2}\) (ban tu rut gon nhe)
c/ \(y'=\dfrac{\left(x^3+x+1\right)'\left(x^3+x+1\right)}{\left|x^3+x+1\right|}=\dfrac{\left(3x^2+1\right)\left(x^3+x+1\right)}{\left|x^3+x+1\right|}\)
d/ \(y'=\dfrac{3x^2-24x^3}{2\sqrt{x^3-6x^4+7}}\)
e/ \(y'=\dfrac{\left(x^5+1\right)'\left(2-\sqrt{x^2+3}\right)-\left(x^5+1\right)\left(2-\sqrt{x^2+3}\right)'}{\left(2-\sqrt{x^2+3}\right)^2}\)
\(y'=\dfrac{5x^4\left(2-\sqrt{x^2+3}\right)+\left(x^5+1\right)\dfrac{x}{\sqrt{x^2+3}}}{\left(2-\sqrt{x^2+3}\right)^2}\)