Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Rút gọn biểu thức
a) \(\sqrt{\left(1+2\sqrt{3}\right)^2}-5\sqrt{3}\)
= \(\left|1+2\sqrt{3}\right|-5\sqrt{3}\)
= \(1+2\sqrt{3}-5\sqrt{3}=1-3\sqrt{3}\)
b) \(3\sqrt{2}+4\sqrt{8}-\sqrt{18}\)
= \(3\sqrt{2}+4\sqrt{2.4}-\sqrt{2.9}\)
= \(3\sqrt{2}+8\sqrt{2}-3\sqrt{2}\) = \(8\sqrt{2}\)
a)\(\sqrt{\left(1+2\sqrt{3}\right)^2}-5\sqrt{3}=1+2\sqrt{3}-5\sqrt{3}=1-3\sqrt{3}\)
b)\(3\sqrt{2}-4\sqrt{8}-\sqrt{18}=3\sqrt{2}-8\sqrt{2}-3\sqrt{2}=-8\sqrt{2}\)
c)\(\dfrac{1}{3+\sqrt{2}}+\dfrac{1}{3-\sqrt{2}}=\dfrac{3-\sqrt{2}+3+\sqrt{2}}{\left(3+\sqrt{2}\right)\left(3-\sqrt{2}\right)}=\dfrac{6}{5}\)

************************************************************
Ta có: \(A=\sqrt{x^2+2x+1}+\sqrt{x^2-2x+1}\)
\(\Leftrightarrow A=\sqrt{\left(x+1\right)^2}+\sqrt{\left(x-1\right)^2}\)
\(\Leftrightarrow A=\left|x+1\right|+\left|1-x\right|\ge\left|x+1+1-x\right|=2\)
Vậy GTNN của A là 2 <=> \(x+1=1-x\Rightarrow x=0\)

a) l14 -3xl – 2x = 2x + 7 ⇔ l14 – 3xl = 4x + 7 (1)
ĐK: 4x + 7 ≥ 0 ⇒ x ≥ -\(\frac{7}{4}\)
(1) ⇔ 14 – 3x = 4x + 7 hoặc 14 – 3x = -4x – 7
⇔ x = 1 (thỏa mãn) hoặc x = -21 (loại)

Chứng minh được:

Suy ra được min P = 11, đạt khi x = y = \(\frac{1}{2}\)

\(A=\sqrt{\left(x+1\right)^2}+\sqrt{\left(x-1\right)^2}=\left|x+1\right|+\left|x-1\right|\)
\(=\left|x+1\right|+\left|1-x\right|\ge\left|x+1+1-x\right|\)\(\ge2\)
Dấu "=" xảy ra khi và chỉ khi \(\hept{\begin{cases}x+1\ge0\\x-1\le0\end{cases}\Rightarrow\hept{\begin{cases}x\ge-1\\x\le1\end{cases}}}\)
vậy Amin= 2 \(\Leftrightarrow-1\le x\le1\)
\(A=\sqrt{x^2+2x+1}+\sqrt{x^2-2x+1}=\sqrt{\left(x+1\right)^2}+\sqrt{\left(x-1\right)^2}\)
\(=\left|x+1\right|+\left|x-1\right|=\left|x+1\right|+\left|1-x\right|\ge\left|x+1+1-x\right|=2\)
Dấu "=" xảy ra \(\Leftrightarrow\left(x+1\right)\left(1-x\right)\ge0\Leftrightarrow-1\le x\le1\)
Vậy \(A_{min}=2\) tại \(-1\le x\le1\)
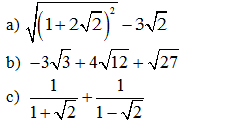
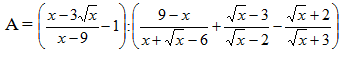

 (Với x > 0; x 1; x4)
(Với x > 0; x 1; x4)


đăng từng bài thui