K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
SG
4
26 tháng 4 2017
a) \(x^3-0,25x=0\\ < =>x\left(x^2-0,25\right)=0\\ =>\left[{}\begin{matrix}x=0\\x^2-0,25=0\end{matrix}\right.< =>\left[{}\begin{matrix}x=0\\x=\sqrt{0,25}\end{matrix}\right.\)
b) \(x^2-10x=-25\\ < =>x^2-10x+25=0\\ < =>\left(x-5\right)^2=0\\ < =>x-5=0\\=>x=5\)
30 tháng 5 2017
a) \(x^3-0,25x=0\)
\(x\left(x^2-0,25\right)=0\)
\(\Leftrightarrow x=0\) hoặc \(x^2-0,25=0\)
\(\Leftrightarrow x=0\) hoặc \(x=0,25\) hoặc \(x=-0,25\)
b) \(x^2-10x=-25\)
\(\Leftrightarrow x\left(x-10\right)=-25\)
\(\Leftrightarrow x=-25\) hoặc \(\Leftrightarrow x-10=-25\)
\(\Leftrightarrow x=-25\) hoặc x=-15
TN
1
2 tháng 8 2017
a) \(x^3-0,25x=0\)
\(\Rightarrow x^3=\dfrac{1}{4}x\)
\(\Rightarrow x^2=\dfrac{1}{4}\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=-\dfrac{1}{2}\end{matrix}\right.\)
b) \(x^2-10x=-25\)
\(\Rightarrow x^2=-25+10x\)
\(\Rightarrow\left[{}\begin{matrix}x=-25+10x\\x=-\left(-25+10x\right)\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}10x-x=-25\\-10x-x=25\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}9x=-25\\-11x=25\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{-25}{9}\\x=-\dfrac{25}{11}\end{matrix}\right.\)
NT
3
HY
18 tháng 2 2020
\(a.x^3-0,25x=0\\\Leftrightarrow x\left(x^2-0,25\right)=0\\ \Leftrightarrow x\left(x-0,5\right)\left(x+0,5\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x-0,5=0\\x+0,5=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=0,5\\x=-0,5\end{matrix}\right.\)
Vậy tập nghiệm của phương trình trên là \(S=\left\{0;0,5;-0,5\right\}\)
PT
2
TN
13 tháng 6 2017
\(x^3-0,25x=0\)
\(\Leftrightarrow x\left(x^2-0,25\right)=0\)
\(\Leftrightarrow x\left(x-0,5\right)\left(x+0,5\right)=0\)
<=>x=0 hoặc x-0,5=0 hoặc x+0,5=0
<=>x=0 hoặc x=0,5 hoặc x=-0,5
H
30 tháng 6 2017
Bài 1:
a) \(9x^2+6xy+y^2=\left(3x+y\right)^2\)
b) \(6x-9-x^2=-9+6x-x^2=-\left(3-x\right)^2\)
c) \(x^2+4y^2+4xy=x^2+4xy+4y^2=\left(x+2y\right)^2\)
Chúc bạn học tốt!
HL
30 tháng 6 2017
1)
a) \(9x^2+6xy+y^2=\left(3x+y\right)^2\)
b) \(6x-9-x^2=-\left(x^2-6x+9\right)=-\left(x-3\right)^2\)
c) \(x^2+4y^2+4xy=\left(x+2y\right)^2\)
2)
a) \(x^3-0,25x=0\)
Bài này có nghiệm x khủng bố lắm, có lẽ đề sai rồi. Nếu đề là 0,125 thì còn làm được...
b) \(x^2-10x=-25\)
\(x^2-10x+25=0\)
\(\left(x-5\right)^2=0\)
\(x-5=0\Rightarrow x=5\)
DN
4 tháng 5 2017
a) 0,25x+1,5=0
=> x = (0 - 1,5) : 0,25 = -1,5 : 0,25 = -6
Vậy x = -6.
b) 6,36−5,3x=0
=> x = (0 + 6,36) : 5,3 = 6,36 : 5,3 =\(\dfrac{6}{5}=1,2\)
Vậy x = 1,2.
c) 43x−56=12
=> x = \(\left(\dfrac{1}{2}+\dfrac{5}{6}\right)\): \(\dfrac{4}{3}\) = \(\dfrac{4}{3}:\dfrac{4}{3}=1\)
Vậy x = 1.
d) −59x+1=23x−10
=> \(\dfrac{-5}{9}x-\dfrac{2}{3}x=\dfrac{-11}{9}x=-10-1=-11\)
=> \(x=-11:\dfrac{-11}{9}=9\)
Vậy x = 9.
7 tháng 11 2021
e) \(\left(9x^2-49\right)+\left(3x+7\right)\left(7x+3\right)=0\)
\(\Rightarrow\text{[}\left(3x\right)^2-7^2\text{]}+\left(3x+7\right)\left(7x+3\right)=0\)
\(\Rightarrow\left(3x-7\right)\left(3x+7\right)+\left(3x+7\right)\left(7x+3\right)=0\)
\(\Rightarrow\left(3x+7\right)\text{[}\left(3x-7\right)+\left(7x+3\right)\text{]}=0\)
\(\Rightarrow\left(3x+7\right)\left(3x-7+7x+3\right)=0\)
\(\Rightarrow\left(3x+7\right)\left(10x-4\right)=0\)
=> 2 TH
*3x+7=0 *10x-4=0
=>3x=-7 =>10x=4
=>x=-7/3 =>x=4/10=2/5
vậy x=-7/3 hoặc x=2/5
g) \(\left(x-4\right)^2=\left(2x-1\right)^2\)
\(\Rightarrow\left(x-4\right)^2-\left(2x-1\right)^2=0\)
\(\Rightarrow\left(x-4-2x+1\right)\left(x-4+2x-1\right)=0\)
\(\Rightarrow\left(-x-3\right)\left(3x-5\right)=0\)
\(\Rightarrow-\left(x+3\right)\left(3x-5\right)=0\)
=> 2 TH
*-(x+3)=0 *3x-5=0
=>-x=-3 =>3x=5
=x=3 =>x=5/3
h)\(x^2-x^2+x-1=0\)
\(\Rightarrow0+x-1=0\)
\(\Rightarrow x-1=0\)
=>x=0+1
=>x=1
vậy x=1
k, x(x+ 16) - 7x - 42 = 0
=>x^2+16x-7x-42=0
=>x^2+9x-42=0
vì x^2>0
do đó x^2+9x-42>0
nên o có gt nào của x t/m y/cầu đề bài
m)x^2+7x+12=0
=>x^2+3x++4x+12=0
=>x(x+3)+4(x+3)=0
=>(x+4).(x+3)=0
=>2 TH
=> *x+4=0
=>x=-4
vậy x=-4
*x+3=0
=>x=-3
vậy x=-3
n)x^2-7x+12=0
=>x^2-4x-3x+12=0
=>x(x-4)-3(x-4)=0
=>(x-3).(x-4)=0
=>2 TH
*x-3=0=>x=0+3=>x=3
*x-4=0=>x=0+4=>x=4
vậy x=3 hoặc x=4
KA
7 tháng 11 2021
a)(3x−3)(5−21x)+(7x+4)(9x−5)=44⇔15x−63x2−15+63x+63x2−35x+36x−20=44⇔79x−35=44⇔79x=79⇒x=1a)(3x−3)(5−21x)+(7x+4)(9x−5)=44⇔15x−63x2−15+63x+63x2−35x+36x−20=44⇔79x−35=44⇔79x=79⇒x=1
b)(x+1)(x+2)(x+5)−x2(x+8)=27⇔x2+2x+x+2(x+5)−x3−8x2=27⇔x2(x+5)+2x(x+5)+x(x+5)+2(x+5)−x3−8x2=27⇔x3+5x2+2x2+10x+x2+5x+2x+10−x3−8x2=27⇔17x+10=27⇔17x=17⇒x=1
CD
4
N
11 tháng 12 2018
\(a,x^3-13x=0\)
\(x.\left(x^2-13\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x=0\\x^2=13\end{cases}\Rightarrow\orbr{\begin{cases}x=0\\x=\sqrt{13}\end{cases}}}\)
\(b,2-25x^2=0\)
\(\Rightarrow25x^2=2\Rightarrow x^2=\frac{2}{25}\Rightarrow x=\sqrt{\frac{2}{25}}\)
\(c,x^2-x+\frac{1}{4}=0\)
\(\left(x-\frac{1}{2}\right)^2=0\Rightarrow x=\frac{1}{2}\)
11 tháng 12 2018
a, x 3 - 13 x = 0
=> x ( x 2 - 13 ) = 0
=> \(\orbr{\begin{cases}x=0\\x^2=13\end{cases}\Rightarrow[\begin{cases}x=0\\x=\sqrt{13}\\x=-\sqrt{13}\end{cases}}\)
b, 2 - 25 x 2 = 0
=> 25 x 2 = 2
=> x 2 = 0,08
=> \(\orbr{\begin{cases}x=\frac{\sqrt{2}}{5}\\x=\frac{-\sqrt{2}}{5}\end{cases}}\)
x, x 2 - x + \(\frac{1}{4}\)= 0
=> \(\left(x-\frac{1}{2}\right)^2=0\)
=> \(x-\frac{1}{2}=0\)
=> \(x=\frac{1}{2}\)
Lời giải: Giải phương trình với tập xác định
-
1
-
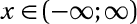
2
-
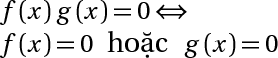
3
-
4
-
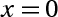
5
-
6
-
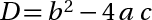
7
-
8
-
9
Ẩn lời giảiTập xác định của phương trình
Lời giải bằng phương pháp phân tích thành nhân tử
Sử dụng phép biến đổi sau
Giải phương trình
Giải phương trình
Biệt thức
Biệt thức
Nghiệm
Lời giải thu được