Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Câu 1:
\(\Leftrightarrow x^2-4x+5+\sqrt{x^2-4x+5}-5=m\)
Đặt \(\sqrt{x^2-4x+5}=\sqrt{\left(x-2\right)^2+1}=a\ge1\)
\(\Rightarrow a^2+a-5=m\) (1)
Xét phương trình: \(x^2-4x+5=a^2\Leftrightarrow x^2-4x+5-a^2=0\)
\(\left\{{}\begin{matrix}x_1+x_2=4\\x_1x_2=5-a^2\end{matrix}\right.\)
\(\Rightarrow\) Nếu \(5-a^2>0\Rightarrow1\le a< \sqrt{5}\) thì pt có 2 nghiệm dương
Nếu \(5-a^2\le0\) \(\Leftrightarrow a\ge\sqrt{5}\) thì pt có 1 nghiệm dương
Vậy để pt đã cho có đúng 2 nghiệm dương thì: (1) có đúng 1 nghiệm thỏa mãn \(1\le a< \sqrt{5}\) hoặc có 2 nghiệm pb \(a_1>a_2\ge\sqrt{5}\)
Xét \(f\left(a\right)=a^2+a-5\) với \(a\ge1\)
\(f'\left(a\right)=0\Rightarrow a=-\frac{1}{2}< 1\Rightarrow f\left(a\right)\) đồng biến \(\forall a\ge1\) \(\Rightarrow y=m\) chỉ có thể cắt \(y=f\left(a\right)\) tại nhiều nhất 1 điểm có hoành độ \(a\ge1\)
\(f\left(1\right)=-3\) ; \(f\left(\sqrt{5}\right)=\sqrt{5}\)
\(\Rightarrow\) Để pt có 2 nghiệm pb đều dương thì \(-3\le m< \sqrt{5}\)
Câu 2:
\(x^2-3x+2\le0\Leftrightarrow1\le x\le2\) (1)
Ta có: \(mx^2+\left(m+1\right)x+m+1\ge0\)
\(\Leftrightarrow m\left(x^2+x+1\right)\ge-x-1\)
\(\Leftrightarrow m\ge\frac{-x-1}{x^2+x+1}=f\left(x\right)\) (2)
Để mọi nghiệm của (1) là nghiệm của (2) \(\Leftrightarrow\left(2\right)\) đúng với mọi \(x\in\left[1;2\right]\)
\(\Rightarrow m\ge\max\limits_{\left[1;2\right]}f\left(x\right)\)
\(f'\left(x\right)=\frac{-\left(x^2+x+1\right)+\left(2x+1\right)\left(x+1\right)}{\left(x^2+x+1\right)^2}=\frac{x^2+2x}{\left(x^2+x+1\right)^2}>0\) \(\forall x\in\left[1;2\right]\)
\(\Rightarrow f\left(x\right)\) đồng biến \(\Rightarrow\max\limits_{\left[1;2\right]}f\left(x\right)=f\left(2\right)=-\frac{3}{7}\)
\(\Rightarrow m\ge-\frac{3}{7}\)

Ta có \(\sqrt{\left(m+2\right)x+m}\ge\left|x-1\right|\Leftrightarrow\left(m+2\right)x+m\ge x^2-2x+1\)
\(\Leftrightarrow m\ge\frac{x^2-4x+1}{x+1}\) (vì \(x\in\left[0;2\right]\)
Xét hàm số \(f\left(x\right)=\frac{x^2-4x+1}{x+1}\) trên đoạn \(\left[0;2\right]\) ta có
\(f'\left(x\right)=\frac{x^2+2x-5}{\left(x+1\right)^2};f'\left(x\right)=0\Leftrightarrow x=-1+\sqrt{6}\)
Lập bảng biến thiên ta được
\(f\left(0\right)=1;f\left(2\right)=-1\)
\(f\left(-1+\sqrt{6}\right)=2\sqrt{6}-6\)
Vậy bất phương trình đã cho có nghiệm thì \(m>\) min (0;2] \(f\left(x\right)=f\left(-1+\sqrt{6}\right)=2\sqrt{6-6}\)

\(\sqrt{3x^2-3}=\sqrt{m-x^3}\)(1)
đk: \(\left\{{}\begin{matrix}\left|x\right|\ge1\\x\le\sqrt[3]{m}\end{matrix}\right.\)(*) \(\Rightarrow3x^2-3=m-x^3\)(2)
để (1) có hai nghiệm phân biệt => (2) phải có hai nghiệm phân biệt thủa mãn (*)
\(\left(2\right)\Leftrightarrow x^3+3x^2-3-m=0\)
\(\Leftrightarrow\left(x+1\right)^3-3\left(x+1\right)-1-m=0\) đặt \(x+1=y\Rightarrow\left[{}\begin{matrix}y\le0\\y\ge2\end{matrix}\right.\)
\(\Leftrightarrow y^3-3y=m+1\)
xét VP
xét khi y<=0
\(A=y^3-3y\)
có \(2-A=2-y^3+3y=\left(2-y\right)\left(y+1\right)^2\) \(\left\{{}\begin{matrix}y\le0\\2-y\ge0\\\left(y+1\right)^2\ge0\end{matrix}\right.\) \(\Rightarrow\left(2-y\right)\left(y+1\right)^2\ge0\)
Vậy \(2-A\ge0\Rightarrow\left\{{}\begin{matrix}y\le0\\A\le2\end{matrix}\right.\)
xét khi y>=2
\(\left\{{}\begin{matrix}y\ge2\\2-y\le0\end{matrix}\right.\) \(\Rightarrow2-A\le0\Rightarrow A\ge2\)
Kết luận: để (1) có đúng 2 nghiệm VT=m+1=2=> m=1
Thử lại với m=1 có hai nghiệm \(\left[{}\begin{matrix}y=-1\\y=2\end{matrix}\right.\)\(\Rightarrow\left\{{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\) thỏa mãn (*)
xét thiếu khi VT <2 có 3 nghiệm nhưng loại một y<2 => thủa mãn có hai nghiệm.
để tính tiếp

Giải:
\(\sqrt{3x^2-3}=\sqrt{m-x^2}\)
Ta thấy hàm \(f(x)=\sqrt{3x^2-3}-\sqrt{m-x^2}\) là hàm chẵn , tức là nếu \(x\) là nghiệm thì \(-x\) cũng là nghiệm. Mà \(3x^2-3\geq 0\) nên \(x\neq 0\), nên phương trình luôn tồn tại hai nghiệm đối nhau phân biệt với mọi \(m\) xác định.
Lúc này, ta chỉ cần xét \(m\) thỏa mãn đkxđ của PT, tức là \(m\geq x^2\geq 1\)
Vậy \(m\geq 1\)

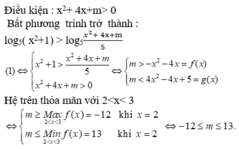
Xét \(f\left(x\right)=3\sqrt{4-3x^2}-2\sqrt{x^3+4x^2+4}\) trên \(\left[-1;1\right]\)
Để \(f\left(x\right)\ge m\) có nghiệm \(\Leftrightarrow m\le\max\limits_{\left[-1;1\right]}f\left(x\right)\)
\(f'\left(x\right)=\frac{-9x}{\sqrt{4-3x^2}}-\frac{3x^2+8x}{\sqrt{x^3+4x^2+4}}=0\)
\(\Leftrightarrow-x\left(\frac{9}{\sqrt{4-3x^2}}+\frac{3x+8}{\sqrt{x^3+4x^2+4}}\right)=0\)
\(\Rightarrow x=0\) (phần ngoặc to luôn dương với mọi \(x\ge-1\))
Từ BBT ta thấy \(\max\limits_{\left[-1;1\right]}f\left(x\right)=f\left(0\right)=2\)
\(\Rightarrow m\le2\)