SG
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
17 tháng 10 2017
Đặt \(\dfrac{x}{3}=\dfrac{y}{2}=k\left(k\in Z\right)\)
\(\Rightarrow\left\{{}\begin{matrix}x=3k\\y=2k\end{matrix}\right.\)
Mà \(x.y^2=96\)
\(\Rightarrow3k.4k^2=96\)
\(\Rightarrow12k^3=96\)
\(\Rightarrow k^3=8\)
\(\Rightarrow k=2\)
TT
17 tháng 10 2017
a. Đặt \(\dfrac{x}{3}=\dfrac{y}{2}=k\Leftrightarrow\left\{{}\begin{matrix}x=3k\\y=2k\end{matrix}\right.\)
mà x . y2 = 96
hay \(3k.\left(2k\right)^2=96\)
\(\Rightarrow3k.4.k^2=96\)
\(\Rightarrow12.k^3=96\)
\(\Rightarrow k^3=8=2^3\)
\(\Rightarrow k=2\)
Với k = 2 \(\Rightarrow\begin{matrix}x=3.2=6\\y=2.2=4\end{matrix}\)
Vậy........
b. Áp dụng t/c dãy tỉ số bằng nhau ta có :
\(\dfrac{x}{3}=\dfrac{y}{5}=\dfrac{2x}{6}=\dfrac{4y}{20}=\dfrac{2x+4y}{6+20}=\dfrac{28}{26}=\dfrac{14}{13}\)
\(\Rightarrow\left\{{}\begin{matrix}\dfrac{x}{3}=\dfrac{14}{13}\Rightarrow x=\dfrac{3.14}{13}=\dfrac{42}{13}\\\dfrac{y}{5}=\dfrac{14}{13}\Rightarrow y=\dfrac{5.14}{13}=\dfrac{70}{13}\end{matrix}\right.\)
Vậy...........
16 tháng 9 2017
1/ a, Ta có :
\(x-2y+3z=35\)
\(\dfrac{x}{3}=\dfrac{y}{4}=\dfrac{z}{5}\)
\(\Leftrightarrow\dfrac{x}{3}=\dfrac{2y}{8}=\dfrac{3z}{15}\)
Áp dụng tính chất dãy tỉ số bằng nhau ta có :
\(\dfrac{x}{3}=\dfrac{2y}{8}=\dfrac{3z}{15}=\dfrac{x-2y+3z}{3-8+15}=\dfrac{35}{10}=\dfrac{7}{2}\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{x}{3}=\dfrac{7}{2}\Leftrightarrow x=\dfrac{21}{2}\\\dfrac{x}{4}=\dfrac{7}{2}\Leftrightarrow y=14\\\dfrac{z}{5}=\dfrac{7}{2}\Leftrightarrow z=\dfrac{35}{2}\end{matrix}\right.\)
Vậy ..
12 tháng 8 2017
Theo đề bài ta có :
\(\dfrac{x-y}{3}=\dfrac{x+y}{13}\)
Áp dụng t/c dãy tỉ số bằng nhau ta có :
\(\dfrac{x-y}{3}=\dfrac{x+y}{13}=\dfrac{x-y+x+y}{3+13}=\dfrac{2x}{16}=\dfrac{x}{8}\)
\(\dfrac{x}{8}=\dfrac{xy}{200}\Leftrightarrow\) \(\dfrac{x}{xy}=\dfrac{8}{200}\Rightarrow\) \(\dfrac{1}{y}=\dfrac{1}{25}\) \(\Rightarrow y=25\)
Thay y = 25 vào biểu thức ta có :
\(\dfrac{x-25}{3}=\dfrac{x+25}{13}\)
\(\Leftrightarrow\) \(13x-325=3x+75\)
\(\Leftrightarrow13x-3x=75+325\)
\(\Leftrightarrow10x=400\)
\(\Rightarrow x=40\)
Vậy \(x=40\) ; \(y=25\)
DH
22 tháng 6 2017
a, \(\left[x\left(x+4\right)\left(x-4\right)-\left(x^2+1\right)\right]x^2-1\)
\(=\left[x\left(x^2-16\right)-\left(x^2+1\right)\right]x^2-1\)
\(=\left[x^3-16x-x^2-1\right]x^2-1\)
\(=x^5-16x^3-x^4-x^2-1\)
b, \(\left(y-3\right)y+3y^2+9-y^2+2\left(y^2-2\right)\)
\(=y^2-3y+3y^2+9-y^2+2y^2-4\)
\(=5y^2-3y+5\)
c, \(\left(x+y\right)\left(x^2x^2-xy+y^2\right)\)
\(=x^5-x^2y+xy^2+x^4y-xy^2+y^3\)
d, \(\left(\dfrac{1}{2}xy+\dfrac{3}{4}y\right).\dfrac{1}{2}xy-\dfrac{3}{4}y\)
\(=\dfrac{1}{4}x^2y^2+\dfrac{3}{8}xy^2-\dfrac{3}{4}y\)
\(=\dfrac{1}{4}y.\left(x^2y+\dfrac{3}{2}xy-3\right)\)
Chúc bạn học tốt!!!
26 tháng 10 2017
a) \(\dfrac{x^3}{8}=\dfrac{y^3}{64}=\dfrac{z^3}{216}\)
Từ \(\dfrac{x^3}{8}=\dfrac{y^3}{64}=\dfrac{z^3}{216}\Rightarrow\dfrac{x^3}{2^3}=\dfrac{y^3}{4^3}=\dfrac{z^3}{6^3}\)
\(\Leftrightarrow\dfrac{x^2}{2^2}=\dfrac{y^2}{4^2}=\dfrac{z^2}{6^2}\Leftrightarrow\dfrac{x^2}{4}=\dfrac{y^2}{16}=\dfrac{z^2}{36}\)
Áp dụng tính chất của dãy tỉ số bằng nhau, ta có:
\(\dfrac{x^2}{4}=\dfrac{y^2}{16}=\dfrac{z^2}{36}=\dfrac{x^2+y^2+z^2}{4+16+36}=\dfrac{14}{56}=\dfrac{1}{4}\)
\(\Rightarrow\dfrac{x^2}{4}=\dfrac{1}{4}\Rightarrow x^2=\dfrac{1}{4}\cdot4\Rightarrow x^2=1\Rightarrow x=1\)
\(\dfrac{y^2}{16}=\dfrac{1}{4}\Rightarrow y^2=\dfrac{1}{4}\cdot16\Rightarrow y^2=4\Rightarrow y=2\)
\(\dfrac{z^2}{36}=\dfrac{1}{4}\Rightarrow z^2=\dfrac{1}{4}\cdot36\Rightarrow z^2=9\Rightarrow z^2=3\)
Xin lỗi mình chỉ làm được câu a)
SG
5
18 tháng 4 2017
Đặt k =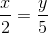 
. Ta có x = 2k, y = 5k
Từ xy=10. suy ra 2k.5k = 10 => 10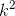 
= 10 => 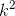= 1 => k = ± 1
Với k = 1 ta được 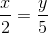= 1 suy ra x = 2, y = 5
Với k = -1 ta được 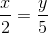= -1 suy ra x = -2, y = -5
TN
8 tháng 7 2017
Gọi \(\dfrac{x}{2}=\dfrac{y}{5}=k\)
Với \(\dfrac{x}{2}=k\Rightarrow x=2k\); \(\dfrac{y}{5}=k\Rightarrow y=5k\)
Theo đề bài,ta còn có:
\(xy=10\)
hay 2k.5k=10
10k2 =10
\(\Rightarrow k=\pm1\)
Với k=1 \(\Rightarrow\dfrac{x}{2}=\dfrac{y}{5}=1\Rightarrow x=2;y=5\)
Với k=-1 \(\Rightarrow\dfrac{x}{2}=\dfrac{y}{5}=-1\Rightarrow x=-2;y=-5\)
18 tháng 6 2018
Bài 1:
1)
\(\dfrac{3x+2}{4}\) = \(\dfrac{5x-3}{3}\)
<=> 3(3x + 2) = 4(5x - 3)
<=> 9x + 6 = 20x - 12
<=> 6 +12 = 20x - 9x
<=> 11x = 18
<=> x = \(\dfrac{18}{11}\)
Vậy: x = \(\dfrac{18}{11}\)
2)
\(\dfrac{x-1}{3x+2}\)= \(\dfrac{1}{5}\)
<=> 5(x - 1) = 3x + 2
<=> 5x - 5 = 3x + 2
<=> 5x - 3x = 2 +5
<=> 2x = 7
<=> x = \(\dfrac{7}{2}\)
Vậy : x = \(\dfrac{7}{2}\)
18 tháng 6 2018
Bài 1 :
1) Ta có :
\(\dfrac{3x+2}{4}=\dfrac{5x-3}{3}\\ \Leftrightarrow4\cdot\left(5x-3\right)=3\cdot\left(3x+2\right)\\ \Leftrightarrow20x-12=9x+6\\ \Leftrightarrow20x-18=9x\\ \Leftrightarrow20x-9x=18\\ \Leftrightarrow11x=18\\ \Leftrightarrow x=\dfrac{18}{11}\\ Vậy.,...\)
2) Ta có :
\(\dfrac{x-1}{3x+2}=\dfrac{1}{5}\Leftrightarrow5\cdot\left(x-1\right)=3x+2\\ \Leftrightarrow5x-5=3x+2\\ \Leftrightarrow5x-3x-5=2\\ \Leftrightarrow2x-5=2\\ \Leftrightarrow2x=7\\ \Leftrightarrow x=\dfrac{7}{2}\)
Vậy ....
Bài 2 ;
1) Áp dụng tính chất của dãy tỉ số bằng nhau ta có :
\(\dfrac{x}{3}=\dfrac{y}{4}=\dfrac{x+y}{3+4}=\dfrac{21}{7}=3\\ \Rightarrow\left\{{}\begin{matrix}x=3\cdot3=9\\y=3\cdot4=12\end{matrix}\right.\\ Vậy...\)
2) Ta có : \(3x=5y\Leftrightarrow\dfrac{x}{5}=\dfrac{y}{3}\)
Áp dụng tính chất của dãy tỉ số bằng nhau ta có :
\(\dfrac{x}{5}=\dfrac{y}{3}=\dfrac{x-y}{5-3}=\dfrac{-16}{2}=-8\\ \Rightarrow\left\{{}\begin{matrix}x=-8\cdot5=-40\\y=-8\cdot3=-24\end{matrix}\right.\\ Vậy....\)
3) Ta có : \(4x=7y\Leftrightarrow\dfrac{x}{7}=\dfrac{y}{4}=\dfrac{x^2}{7^2}=\dfrac{y^2}{4^2}=\dfrac{x\cdot y}{7\cdot4}\\ \Leftrightarrow\dfrac{x}{7}=\dfrac{y}{4}=\dfrac{112}{28}=4\\ \Rightarrow\left\{{}\begin{matrix}x=4\cdot7=28\\y=4\cdot4=16\end{matrix}\right.\\ Vậy...\)
TV
15 tháng 6 2018
Ta có:
\(\left\{{}\begin{matrix}x^2+xy+\dfrac{y^2}{3}=2019\\z^2+\dfrac{y^2}{3}=1011\\x^2+xz+z^2=1008\end{matrix}\right.\Leftrightarrow x^2+xy+\dfrac{y^2}{3}=z^2+\dfrac{y^2}{3}+x^2+xz+z^2\)
\(\Rightarrow xy=2z^2+xz\Leftrightarrow xy+xz=2z^2+2xz\)
\(\Rightarrow x\left(y+z\right)=2z\left(x+z\right)\Leftrightarrow\dfrac{2z}{x}=\dfrac{y+z}{x+z}\left(đpcm\right)\)
xy = 96 => x = 96/y => 2/x = y/48
=> y/48 = 3/y => y = 12 hoặc -12
=> x = 8 hoặc -8
\(\dfrac{2}{x}=\dfrac{3}{y}\) và x.y =96
\(=>\dfrac{x}{2}=\dfrac{y}{3}=k\)
=> x = 2k và y = 3k
Thay vào x.y = 96
(2k . 3k) = 96
\(6k^2=96\)
\(k^2=96:6\)
\(k^2=16\)
\(k=-4\) hoặc \(+4\)
Với k = - 4 => x = 2 . ( - 4 ) = - 8
y = 3 . ( - 4) = - 12
Với k = 4 => x = 2 . 4 = 8
y = 3 . 4 = 12