
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(A=x^4+6x^3+13x^2+12x+12\)
\(=\left(x^4+6x^3+19x^2+30x+25\right)-6x^2-18x-30+17\)
\(=\left(x^4+6x^3+19x^2+30x+25\right)-6\left(x^2+3x+5\right)+17\)
\(=\left(x^2+3x+5\right)^2-6\left(x^2+3x+5\right)+17\)
Đặt \(t=x^2+3x+5\)
Khi đó \(A=t^2-6t+17=t^2-2.t.3+9+8=\left(t-3\right)^2+8\ge8\)
Dấu "=" xảy ra <=> t - 3 = 0 <=> t = 3
<=> \(x^2+3x+5=3\Leftrightarrow x^2+3x+2=0\)
\(\Leftrightarrow x^2+x+2x+2=0\)
\(\Leftrightarrow x\left(x+1\right)+2\left(x+1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=-1\\x=-2\end{cases}}\)
Vậy AMin = 8 khi và chỉ khi x = -1 hoặc x = -2

\(A=\left(x^4-3x^3+2x^2\right)-3\left(x^3-3x^2+2x\right)+2\left(x^2-3x+2\right)+2019\)
\(=x^2\left(x^2-3x+2\right)-3x\left(x^2-3x+2\right)+2\left(x^2-3x+2\right)+2019\)
\(=\left(x^2-3x+2\right)\left(x^2-3x+2\right)+2019\)
\(=\left(x^2-3x+2\right)^2+2019\ge2019\)
\(A_{min}=2019\) khi \(\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)

a: \(A=\left(\dfrac{2\left(2x+1\right)}{2\left(2x+4\right)}-\dfrac{x}{3x-6}-\dfrac{2x^3}{3x^3-12x}\right):\dfrac{6x+13x^2}{24x-12x^2}\)
\(=\left(\dfrac{2x+1}{2\left(x+2\right)}-\dfrac{x}{3\left(x-2\right)}-\dfrac{2x^3}{3x\left(x^2-4\right)}\right):\dfrac{x\left(13x+6\right)}{x\left(24-12x\right)}\)
\(=\left(\dfrac{2x+1}{2\left(x+2\right)}-\dfrac{x}{3\left(x-2\right)}-\dfrac{2x^2}{3\left(x-2\right)\left(x+2\right)}\right):\dfrac{13x+6}{-12\left(x-2\right)}\)
\(=\dfrac{3\left(2x+1\right)\left(x-2\right)-2x\left(x+2\right)-4x^2}{6\left(x+2\right)\left(x-2\right)}\cdot\dfrac{-12\left(x-2\right)}{13x+6}\)
\(=\dfrac{3\left(2x^2-3x-2\right)-2x^2-4x-4x^2}{x-2}\cdot\dfrac{-2}{13x+6}\)
\(=\dfrac{6x^2-9x-6-6x^2-4x}{x-2}\cdot\dfrac{-2}{13x+6}\)
\(=\dfrac{-\left(13x+6\right)\cdot\left(-2\right)}{\left(13x+6\right)\left(x-2\right)}=\dfrac{2}{x-2}\)
b: Để A>0 thì x-2>0
hay x>2
Để A>-1 thì A+1>0
\(\Leftrightarrow\dfrac{2+x-2}{x-2}>0\)
=>x/x-2>0
=>x>2 hoặc x<0


Đề sai một chút nha bạn : mình sửa bạn thử tham khảo xem đúng không \(P=\frac{12x^2-6x+4}{\left(x-1\right)^2}\)
Mình làm luôn nha
Giải
Theo bài ra , ta có :
\(P=\frac{12x^2-6x+4}{\left(x-1\right)^2}=\frac{12\left(x^2-2x+1\right)+18x-8+10x-10+10}{\left(x-1\right)^2}=\frac{12\left(x-1\right)^2+18\left(x-1\right)+10}{\left(x-1\right)^2}=12+\frac{18}{x-1}+\frac{10}{\left(x-1\right)^2}\)
Đặt \(\frac{2}{x-1}=y\)
Đến đây bạn tự làm tiếp nhé

a)1-6x2-x =0<=>-(6x2+x-1)=0<=>6x2+x-1=0
<=>(6x2+3x)-(2x+1)=0<=>3x(2x+1)-(2x+1)=0
<=>(3x-1)(2x+1)=0
=>3x-1=0 hoặc 2x+1=0=>x=\(\dfrac13\) hoặc x=-\(\dfrac12\)
Vậy S={\(\dfrac13\);-\(\dfrac12\)}
b)12x2+13x+3=0<=>12x2+9x+4x+3=0<=>(12x2+9x)+(4x+3)=0
<=>3x(4x+3)+(4x+3)=0<=>(3x+1)(4x+3)=0
=>3x+1=0 hoặc 4x+3=0 <=>x=-\(\dfrac13 \) hoặc x=-\(\dfrac34\)
Vậy S={-\(\dfrac13 \);-\(\dfrac34 \)}
c)x3-11x2+30x=0<=>x(x2-11x+30)=0<=>x[(x2-6x)-(5x-30)]=0
<=>x[x(x-6)-5(x-6)]=0<=>x(x-5)(x-6)=0
=>x=0 hoặc x-5=0 hoặc x-6=0=>x=0 hoặc x=5 hoặc x=6
Vậy S={0;5;6}
d)Ta có:(x2+x+1)(x2+x+2)-12=0
Đặt:t=x2+x+1
Khi đó:a(a+1)-12=0<=>a2+a-12=0<=>(a2+4a)-(3a+12)=0
<=>a(a+4)-3(a+4)=0<=>(a-3)(a+4)=0
hay (x2+x-2)(x2+x+5)=0
<=>(x-1)(x+2)(x2+x+5)=0(x2+x-2=(x-1)(x+2))
=>x-1=0 hoặc x+2=0(vì x2+x+5=(x+\(\dfrac12\))2+\(\dfrac{19}{4}\)>0)
=>x=1 hoặc x=-2
Vậy S={1;-2}
e)Ta có:2x2+x+6>x2+x+6=(x+\(\dfrac12\))2+\(\dfrac{23}{4}\)>0
nên PT vô nghiệm
Vậy S=\(\varnothing\)
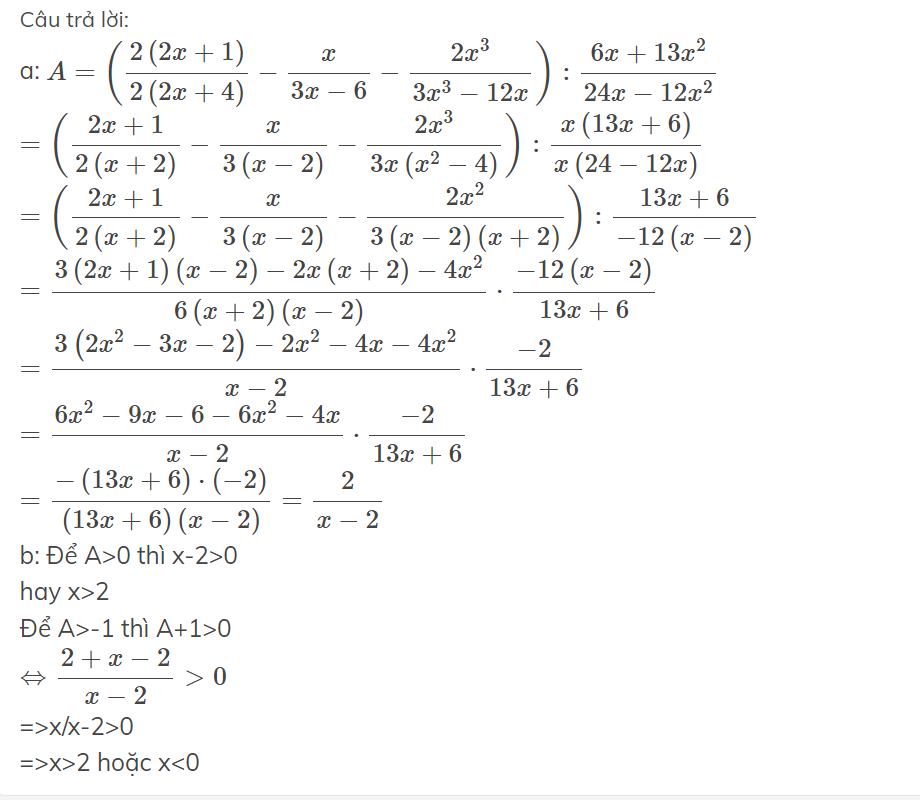
Cách mình dài hơn ạ :
\(A=x^4+6x^3+9x^2+4x^2+12x+12\)
\(=\left(x^2+3x\right)^2+4\left(x^2+3x\right)+4+8\)
\(=\left(x^2+3x+2\right)^2+8\ge8\)
Dấu "=" xảy ra khi \(\left[{}\begin{matrix}x=-1\\x=-2\end{matrix}\right.\)