H
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
H
1
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 3 2020
\(x^2-5x+6\le0\Leftrightarrow\left(x-2\right)\left(x-3\right)\le0\Rightarrow2\le x\le3\) (1)
\(x^2-4\le0\Leftrightarrow\left(x-2\right)\left(x+2\right)\le0\Rightarrow-2\le x\le2\) (2)
(1);(2) \(\Rightarrow x=2\)
D
1
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 3 2022
Tập nghiệm của BPT là: \(\left[{}\begin{matrix}-3< x\le-1\\0\le x< 1\\x>1\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 5 2019
ĐKXĐ: \(x\left(x+1\right)\left(x-3\right)\ge0\Rightarrow\left[{}\begin{matrix}-1\le x\le0\\x\ge3\end{matrix}\right.\)
\(\left(x-2\right)\sqrt{x\left(x+1\right)\left(x-3\right)}\le0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2\le0\\\sqrt{x\left(x+1\right)\left(x-3\right)}=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x\le2\\x=-1\\x=0\\x=3\end{matrix}\right.\)
Kết hợp với ĐKXĐ ta được: \(\left[{}\begin{matrix}-1\le x\le0\\x=3\end{matrix}\right.\)
Vậy \(\left\{{}\begin{matrix}x_1=-1\\x_2=3\end{matrix}\right.\) \(\Rightarrow S=5\)
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 5 2019
ĐKXĐ: \(9-x^2>0\Rightarrow-3< x< 3\)
\(\frac{\left(x^2-3x\right)\sqrt{9-x^2}}{\sqrt{9-x^2}}\ge0\Leftrightarrow x^2-3x\ge0\Leftrightarrow\left[{}\begin{matrix}x\le0\\x\ge3\end{matrix}\right.\)
Kết hợp ĐKXĐ ta được nghiệm của BPT: \(-3< x\le0\)
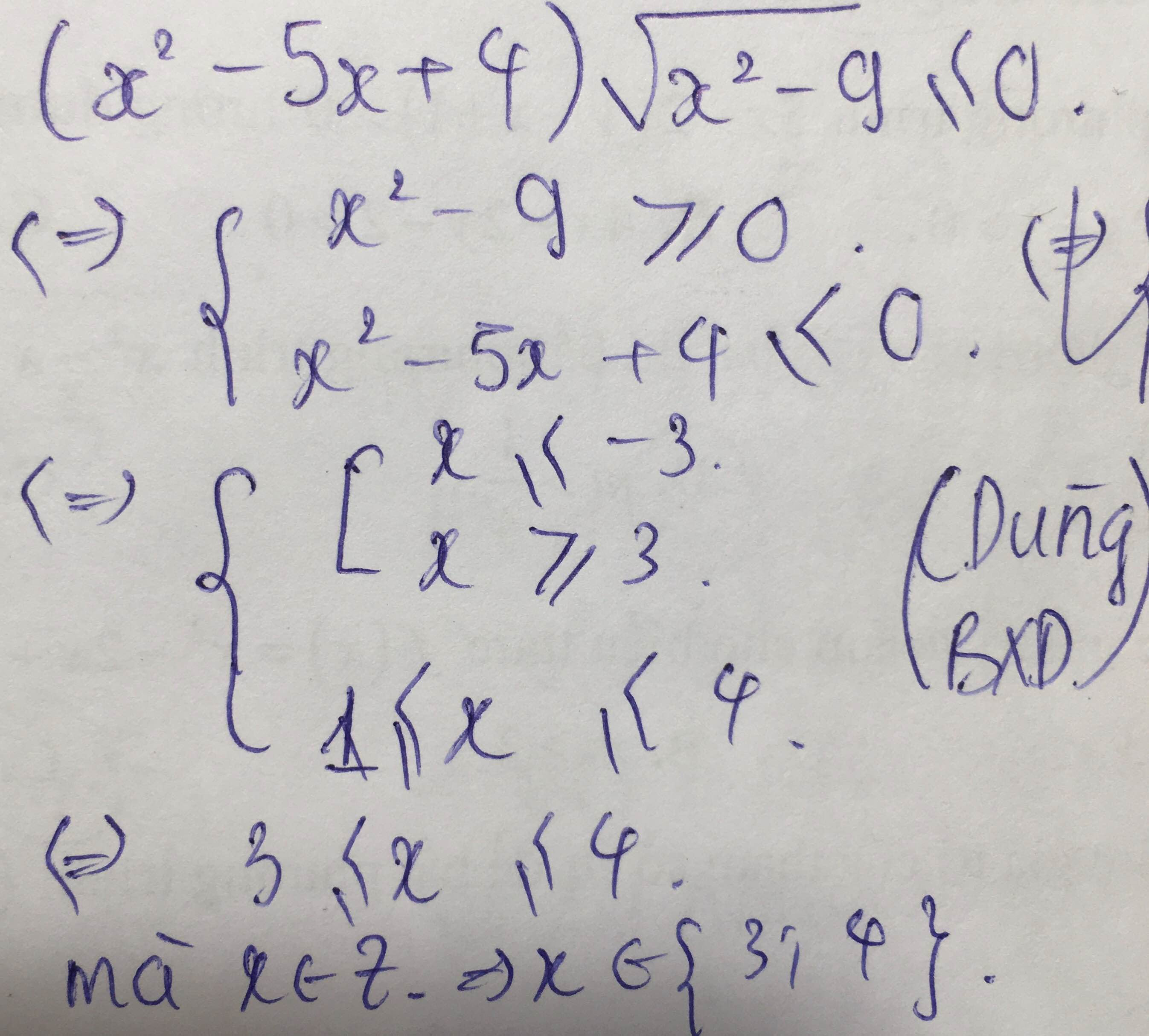
x=\(\left\{{}\begin{matrix}x=3\\x=-3\\x=-2\end{matrix}\right.\)
đề là \(\le\) 0 mà bạn